A disc of radius R and mass M is plvoted at the rim and is set for small oscillation. if simple pendlum has to have the same period as that the of the disc, the length of the simple pendlum should to
54R
23R
34R
32R
The correct Answer is:D
Time period of a physical pendulum
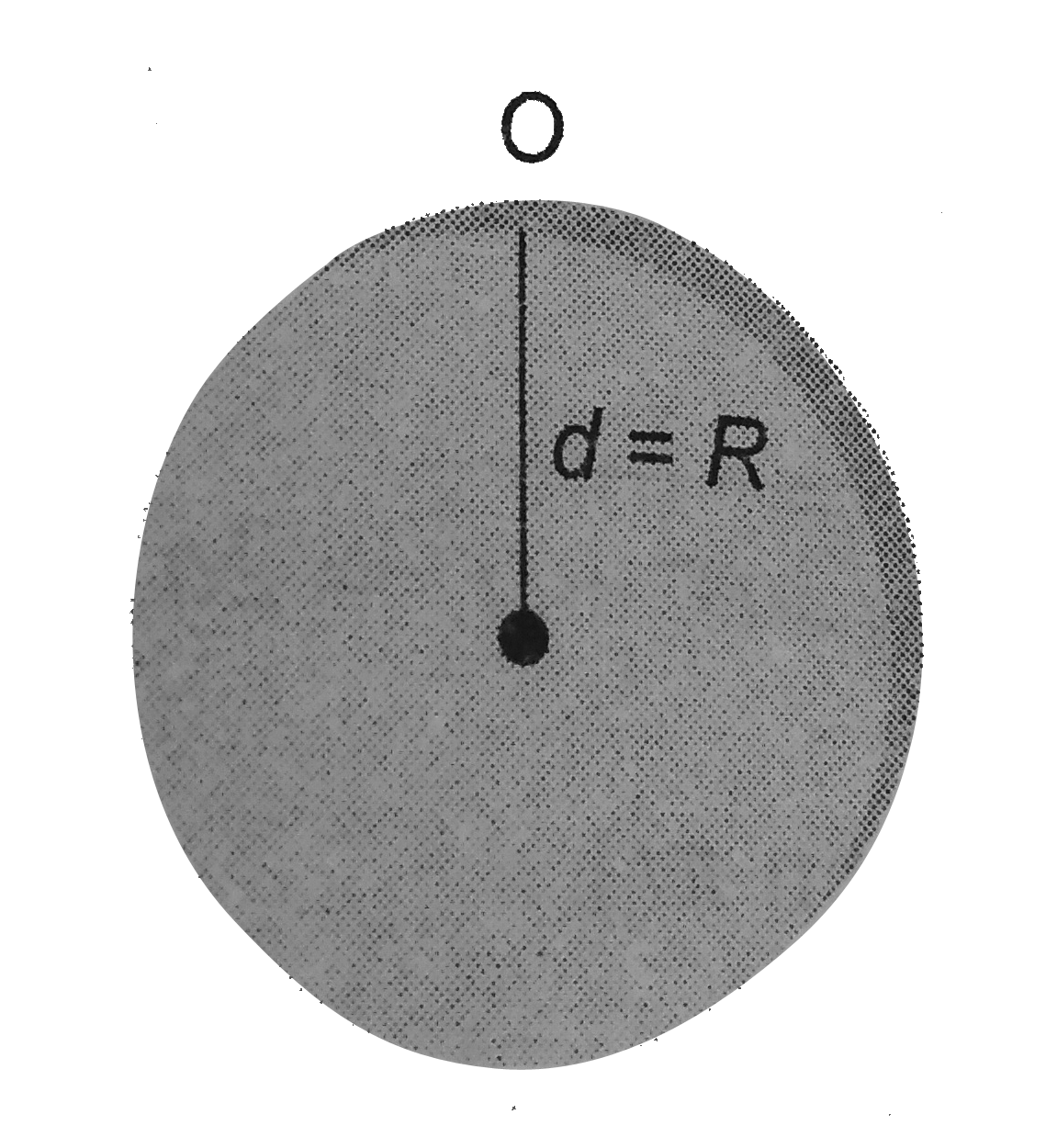
T=2π√I0mgd=2π
⎷(I0mgdmR2+mR2)mgR
=2π√3R2g ….(i)
Tsimple pendulum=2π√1g ….(ii)
Equating (i) and (ii) l=32R.
Topper's Solved these Questions
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Problems Based On Mixed Concepts24videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Assertion Reasoning22videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - OSCILLATION AND SIMPLE HARMONIC MOTIONEXERCISE - Simple Pendulum And Different Cases Of Shm25videos
OSCILLATION AND SIMPLE HARMONIC MOTION
- BOOK - A2ZCHAPTER - NEWTONS LAWS OF MOTIONEXERCISE - Chapter Test30videos
NEWTONS LAWS OF MOTION
- BOOK - A2ZCHAPTER - PROPERTIES OF MATTEREXERCISE - Chapter Test29videos
PROPERTIES OF MATTER
Similar Questions
A uniform rod of length l is suspended by end and is made to undego small oscillations. Find the length of the simple pendulum having the time period equal to that of the rod.
View SolutionA disc of radius R and mass m is mounted on a vertical axis through its centre and perendicular to its plane. A rat of same mass m runs around the rim of the disc with speed v anticlockwise relative to stationary observer. The disc rotates clockwise with angular speed omega. The rat finds a piece of bread on the rim and stops. Find the angular velocity of the disc after the rat stops.
 View Solution
View Solution
Knowledge Check
- Question 1 - Select One
A disc of radius R and mass M is pivoted at the rim and it set for small oscillations. If simple pendulum has to have the same period as that of the disc, the length of the simple pendulum should be
A5R4B2R3C3R4D3R2 - Question 2 - Select One
A disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
A2π√RgB2π√2RgC2π√2π3gD2π√3R2g - Question 3 - Select One
A disc of radius R is suspended from its circumference and made to oscillate. Its time period will be:
A2π√3R2gB2π√4RgC2π√RgD2π√2Rg
A uniform circular disc of mass M and radius R is pivoted at distance x above the centre of mass of the disc, such that the time period of the disc in the vertical plane is infinite. What is the distance between the pivoted point and centre of mass of the disc ?
View SolutionA disc of mass 'm' is suspended at a point R/2 above its centre. Find its period of oscillation.
View SolutionA circular hoop of radius R is hung over a knife edge. The period of oscillation is equal to that of a simple pendulum of length
View SolutionFrom a disc of radius R and mass M , a circular hole of diameter R , whose rim passes through the centre is cut . What is the moment of inertia of the remaining part of the disc about a perpendicular axis , passing through the centre ?
View SolutionA uniform disc of radius R is pivoted at point O on its circumstances. The time period of small oscillations about an axis passing through O and perpendicular to plane of disc will be
View Solution

