From the surface of a round wire of radius a carrying a direct current i, a positive charge q escapes with a velocity v0 perpendicular to the surface. Find what the maximum distance of the electron will ne from the axis of the wire before it turns back due to the action of the magnetic field generated by the current.
Let at any instant velocity of charge is v, then force on the charge due to magnetic field caused by wire is given by
→F=q(→v×→B)
maxˆi+mayˆj=q(vxˆi+vzˆk)×μ0I2πxˆj
maxˆi+mayˆj=qvxμ0I2πxˆk−qvzμ0I2πxˆi

⇒max=−qμ0I2πvzx
⇒ax=−qμ0I2πmvzx
["But" v20=v2x⇒vz=±√v20−v2x
Here we have to take vz=+√v20−v2x) because ax is negative]
⇒vxdvxdx=−qμ0I2πm√v20−v2xx
⇒∫0v0vxdvx√v20−v2x=qμ0I2πm∫xmaxadxx
⇒[−√v20−v2x]0v0=−qμ0I2πmln((xmaxa)
⇒v0=qμ0I2πmln((xmaxa)
⇒xmax=ae2πmv0qμ0I
when x is miximum, x component of velocity is zero.
Topper's Solved these Questions
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.1
35 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - Concept Exercise 2.2
10 Videos
- SOURCES OF MAGNETIC FIELDBOOK - CENGAGE PHYSICSCHAPTER - SOURCES OF MAGNETIC FIELDEXERCISE - single correct Ansewer type
12 Videos
- SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSBOOK - CENGAGE PHYSICSCHAPTER - SEMICONDUCTOR ELECTRONIC : MATERIALS, DEVICES AND SIMPLE CIRCUITSEXERCISE - QUESTION BANK
12 Videos
- THERMAL PROPERTIES OF MATTERBOOK - CENGAGE PHYSICSCHAPTER - THERMAL PROPERTIES OF MATTEREXERCISE - Question Bank
40 Videos
Similar Questions
From the surface of a round wire of radius a carrying a direct current I an electron escapes with a velocity v0 perpendicular to the surface. Find what will be the maximum distance of the electron from the axis of the wire before it turns back due to the action of the magnetic field generated by the current.
View SolutionA thick wire of radius r=5mm carries a steady current I=10A. An electron leaves its surface perpendicularly with velocity v0=106m/s. Find the maximum distance from the surface of the wire before it turns back.
View Solution
Knowledge Check
A wire carries current toward the north. In the region beneath this wire, What is the direction of the magnetic field due to the current ?
ANorthwardBSouthwardCEastwardDWestwardMagnetic field exist in the space and given as →B=−B0l2x2ˆk, where B0and l positive constants. A particle having positive charge 'q' and mass 'm' is pojected wit speed 'v0' along positive x axis from the origin. What is the maimum distance of the charged particle from the y-axis before it turns back due to the magnetic field. (Ignore any interaction other than magnetic field)
A(ml2v03qB0)1/3B(3ml2v02qB0)1/3C(3ml2v0qB0)1/3D(ml2v0qB0)1/3A long straight wire of radius 10mm carries a current uniformly distributed over its cross section. The ration of magnetic fields due to the wire at distance of 5mm and 15 mm, respectively from the axis of the wire is
A34B23C13D2
An electric current flows in a wire from north to south.
What will be the direction of the magnetic field due to this wire at a point.
View SolutionA semicircular wire of radius 5.0 cm carries a current of 5.0 A. A magnetic field B of magnitude 0.50 T exists along the perpendicular to the plane of the wire. Find the magnitude of the magnetic force acting on the wire.
View Solution(i) A very long wire carrying a current I is bent at right angles .Find magnetic field at a point lying on a perpendicular to the wire , drawn through the point of bending at a distance d from it
(ii) Three long wires carrying same currect are placed as shown Find magnetic field at O.
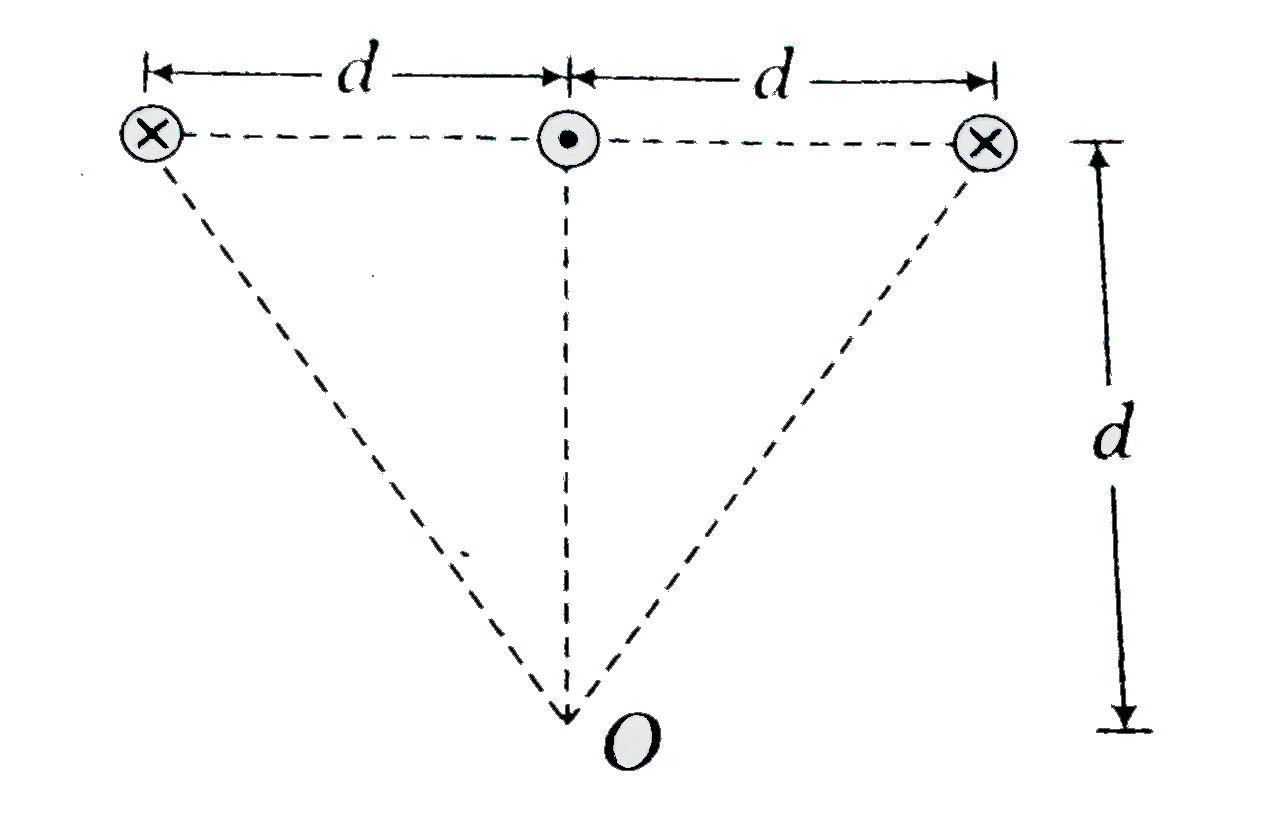
View SolutionAt a distance of 10 cm from a long straight wire carrying current the magnetic field is 0.04 T. At the distance of 20 cm, the magnetic field will be
View SolutionAn electron is ejected from the surface of a long, thick straight conductor carrying a current, initially in a direction perpendicular to the conductor. The electron will
View Solution
