Doubtnut Promotions Sticky

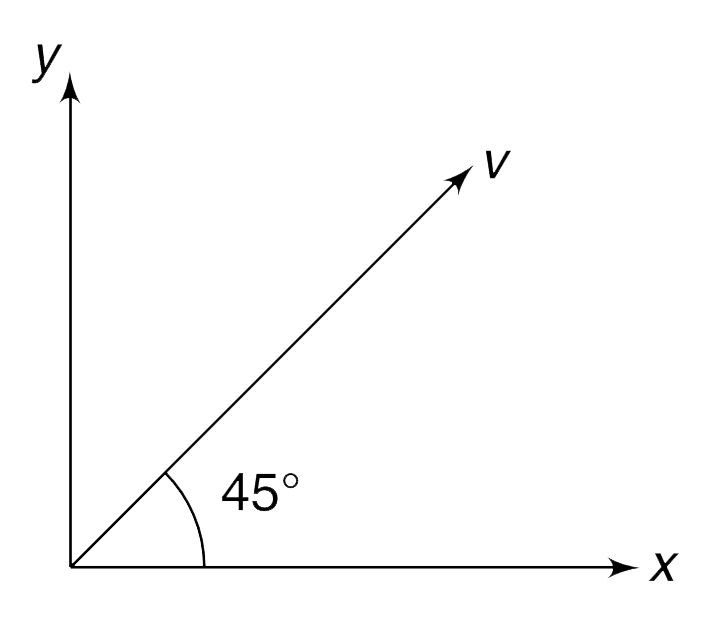
A charged particle carrying charge q=10μC moves with velocity v1=106,s−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
Find the magnetic field →B.

A

(10−3T)(ˆi+ˆj)

B

(2×10−3T)ˆi

C

(10−3T)ˆi

D

(2×10−3T)(ˆi+ˆj)

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:c

For the first case: →F=q→v×→B
⇒−5√2×10−3ˆk
=10−5×106√2(ˆi+ˆj)×(Bxˆi+Byˆj+Bzˆk)
=(10√2)[Bzˆi−Bzˆj+(By+Bx)ˆk]
⇒Bz=0,By−Bx=−10−3T....(i)
Similarly, for the second case:
F2ˆj=(10−5)(106ˆk)×[(Bxˆi+Byˆj+Bzˆk)]
F2ˆj=10(Bxˆj−Byˆi)
F2=10Bx,By=0....(ii)
Using eqs. (i) and (ii), we get Bx=10−3T
Thus, →B=(10−3T)ˆi
Also, F2=10Bx=10−2N.

|
Updated on:21/07/2023
Class 12PHYSICSMOVING CHARGES AND MAGNETISM

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Question 1 - Select One

    A charged particle carrying charge q=10μC moves with velocity v1=106,s−1 at angle 45∘ with x-axis in the xy plane and experience a force F1=5√2mN along the negative z-axis. When the same particle moves with velocity v2=106ms−1 along the z-axis, it experiences a force F2 in y-direction.
    Find the magnitude of the force F2.

    A10−2N
    B10−3N
    C10−4N
    D10−5N
  • Question 2 - Select One

    When a charge of 1C moving with velocity 1 m/s normal to a magnetic field experiences a force 1N, then the magnitude of them magnetic field is.

    A1 Gauss
    B1 Tesla
    C1 Orested
    DNone of the above
  • Question 3 - Select One

    A particle of charge −16×10−18coo–mb moving with velocity 10ms−1 along the x−aξs, and an electric field of magnitude (104)/(m) is along the negative z−ais. If the charged particle continues moving along the x−aξs , the magnitude of B is

    A(103Wb)/(m2)
    B(105Wb)/(m2)
    C(1016Wb)/(m2)
    D(10−3Wb)/(m2)