A straight wire lies along a body diagonal of an imaginary cube of side a=20cm, and carries a current of 5 A (as shown in Fig. 1.61). Find the force on it due to a uniform field →B=0.6ˆjT.

In order to use equation →F=i→l×→B, we would need to find the
angle between →land→B. We avoid this task by using unit vector
notation. Form the problem figure, we see that
→l=aˆi−aˆj+aˆk
Thus, the force is →F=I→l×→B=IaB(ˆi−ˆj+ˆk)×(ˆj)
=IaB(−ˆi+ˆk)
The force lies in the xz plane and has a magnitude
F=√2IaB=0.85N
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise1.3
14 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Subjective
10 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.1
22 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
A long, straight wire lies along the y-axis and carries a current I=8A in the y-direction (as shown in Fig) In addition to the magnetic field due to the current in the wire, a uniform magnetic field →B0 with magnitude 1.50×10−6T is in the +x direction. What is the total field (magnitude and direction ) at following points in the plane?
(a)x=0,z=1.00m
(b) x=1.00m,z=0
(c) x=0,z=−0.25m

View SolutionThe square loop in Fig. has sides of length 20 cm. It has 5 turns and carries a current of 2 A. The normal to the loop is at 37∘ to a uniform field →B=0.5ˆjT.
a. Find the magnetic moment of the loop.
b. Find the work needed to rotate the loop from its position of minimum energy to the given orientation.

View Solution
Knowledge Check
The magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1The rigid conducting thin wire frame carries an electric current I and this frame is inside a uniform magnetic field →B as shown in Fig. 1.146. Then,
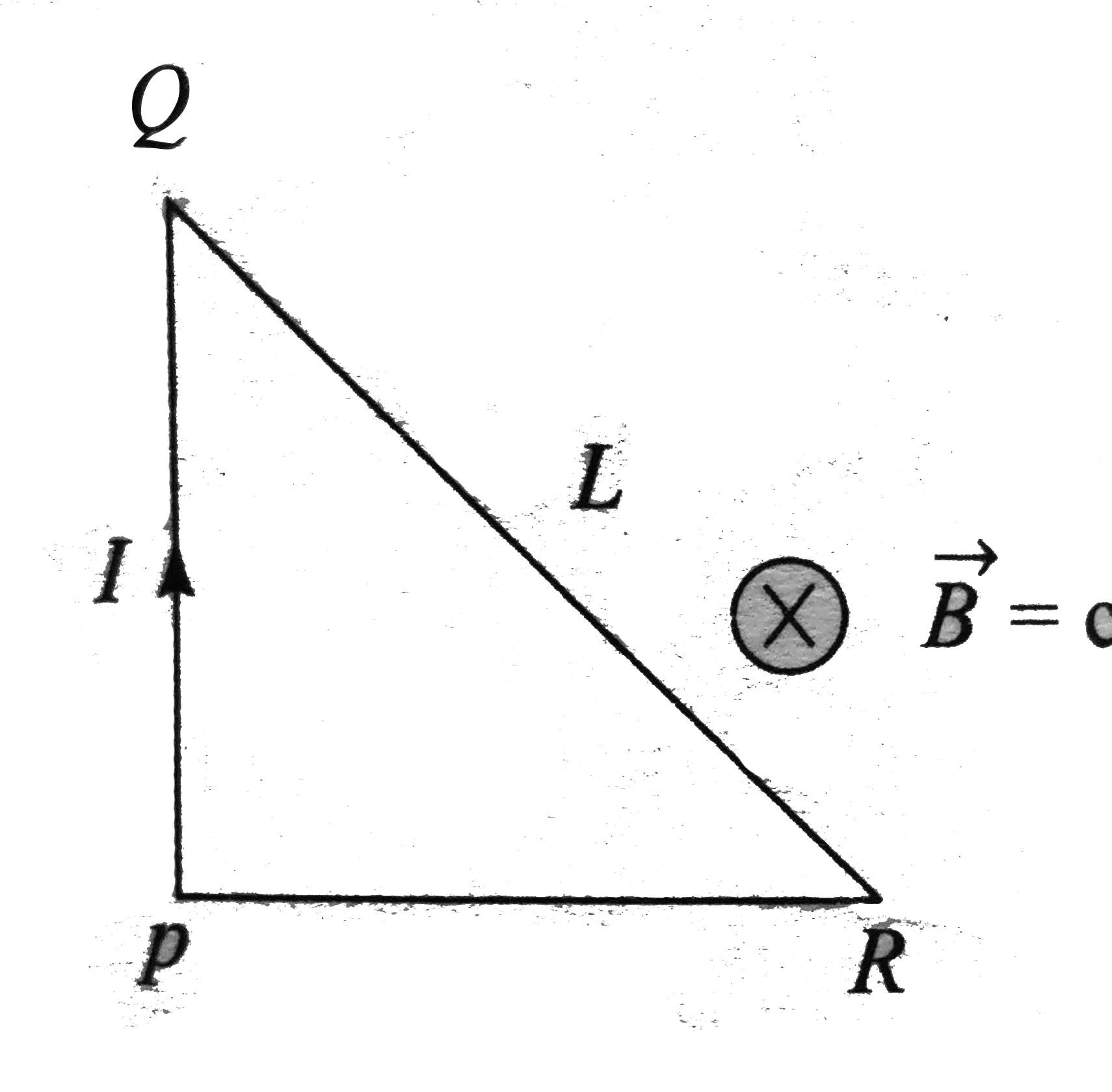 AThe net magnetic force on the frame is zero but the torque is not zeroBThe net magnetic force on the frame and the torque due to magnetic field are both zero.Cthe net magnetic force on the frame is not zero and the torque is also not zero.DNone of these
AThe net magnetic force on the frame is zero but the torque is not zeroBThe net magnetic force on the frame and the torque due to magnetic field are both zero.Cthe net magnetic force on the frame is not zero and the torque is also not zero.DNone of theseThe magnetic force per unit length on a wire carrying a current of 10 A and making an angle of 45∘ with the direction of a uniform magnetic field of 0.20 T is
A2√2Nm−1B2√2Nm−1C√22Nm−1D4√2Nm−1
A copper wire of diameter 1.6 mm carries a current of 20A. Find the maximum magnitude of the magnetic field (vec B)due to this current.
View SolutionCalculate the force on a current carrying wire in a uniform magnetic field as shown in Fig. 1.59.
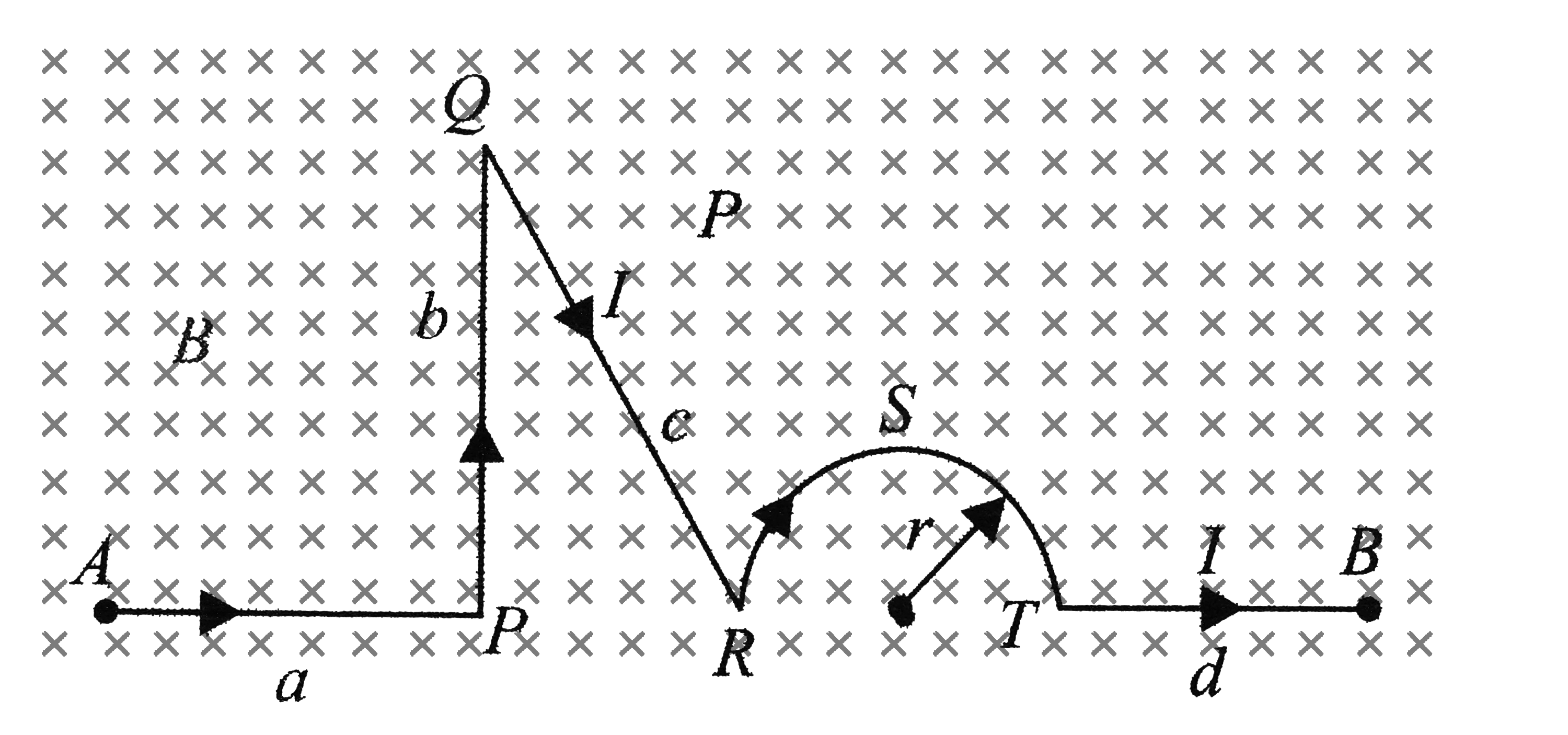
View SolutionA straight wire carrying a current of 5 A is bent into a semicircular arc of radius 2 cm as shown in the fig. Find the magnitude and direction of the magnetic field at the centre of the arc.

View SolutionA straight wire of1.4 m long carries a current of 7 A at right angles to a uniform magnetic field of 0.01 T. Calculate the mechanical force acting on the wire due to the magnetic field.
View SolutionThe bent wire shown in figure lies in a uniform magnetic field. Each straight section is 2.0 m long and makes an angle θ=60∘ with the x-axis , and the wire carries a current of 2.0A . What is the net magnetic force on the wire in unit -vector rotation if the magnetic field is given by (a) 4.0ˆkT and (b) 4.0ˆiT ?

View Solution