A circular coil with area A and N turns is free to rotate about a diameter that coincides with the x-axis. Current I is circulating in the coil. There is a uniform magnetic field →B in the the positive y direction. Calculate the magnitude and direction of the torque →τ and the value of the potential energy U, when the coil is oriented as shown in parts (a) through (d) of Fig. 1.79.




(a) ϕ=90∘:τ=NIABsin(90∘)=NIAB
Direction: ˆk׈j=−ˆi,U=−MBcosϕ=0
(b) ϕ=0∘:τ=NIABsin(0)=0 no direction,
M=NIA,U=−MBcosϕ=−NIAB
(c) ϕ=90∘:τ=NIABsin(90∘)=NIAB
Direction: −ˆk׈j=−ˆi,U=−MBcosϕ=0
(d) ϕ=180∘:τ=NIABsin(180∘)=0 no direction,
U=−MBcosϕ=−NIAB
Topper's Solved these Questions
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Subjective
10 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercises Single Correct
70 Videos
- MAGNETIC FIELD AND MAGNETIC FORCESBOOK - CENGAGE PHYSICSCHAPTER - MAGNETIC FIELD AND MAGNETIC FORCESEXERCISE - Exercise 1.2
13 Videos
- KINETIC THEORYBOOK - CENGAGE PHYSICSCHAPTER - KINETIC THEORYEXERCISE - Question Bank
31 Videos
- Magnetism and MatterBOOK - CENGAGE PHYSICSCHAPTER - Magnetism and MatterEXERCISE - Question Bank
50 Videos
Similar Questions
A square 12-turn coil with sides of length 40 cm carries a current of 3 A. It lies in the x-y plane as shown in Fig. in a uniform magnetic field.
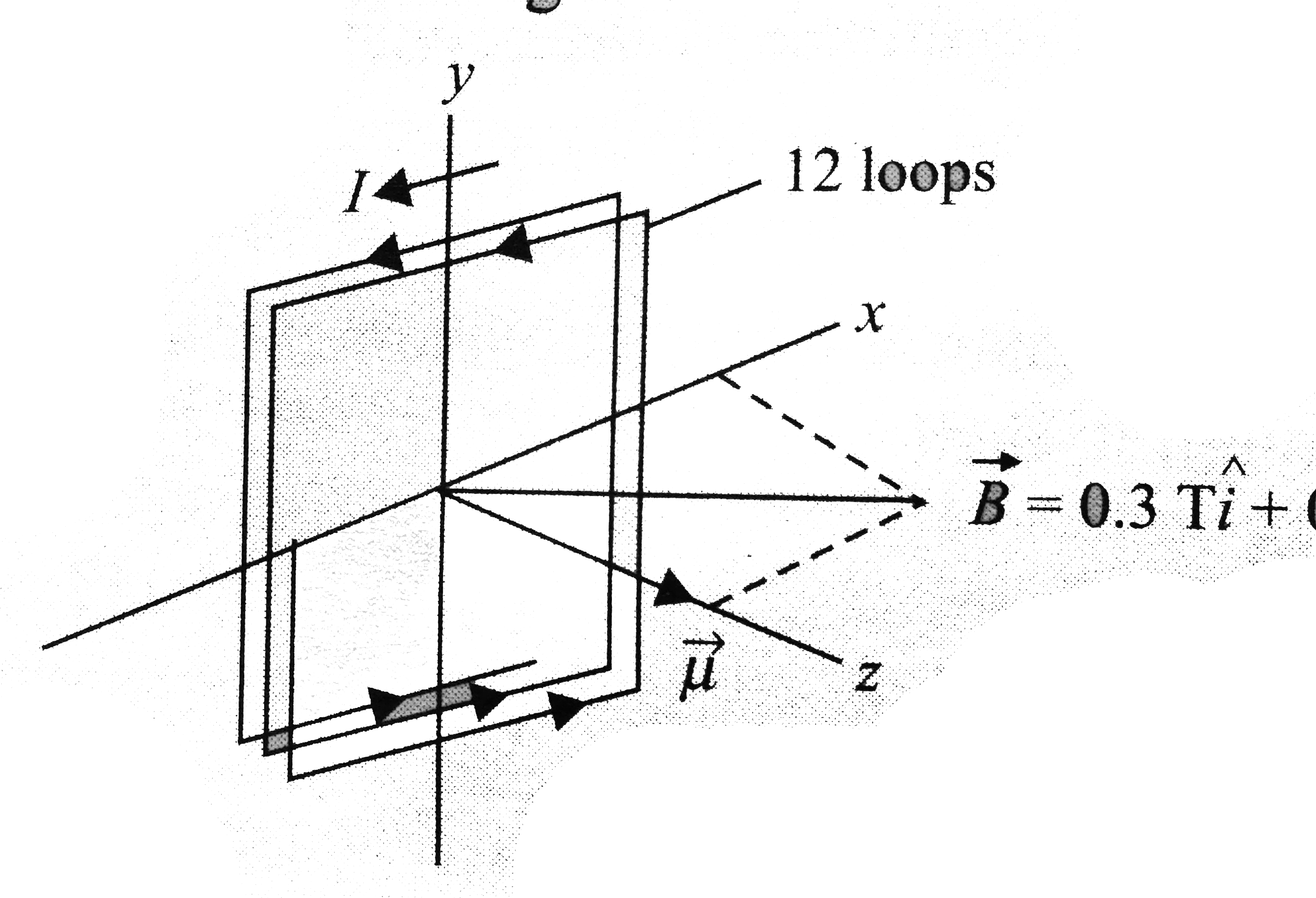
(a) Find the magnetic moment of the coil.
(b) Find the torque exerted on the coil.
(c) Find the potential energy of the coil.
View SolutionA circular coil of cross-sectional area 200cm2 and 20 turns is rotated about the vertical diameter with angular speed of 50rad/s in a uniform magnetic of 3.0×10−2T. Calculate the maximum value of current in the coil.
View Solution
Knowledge Check
A current i flows in a circular coil of radius r. If the coil is placed in a uniform magnetic field B with its plane parallel to the field, magnitude of the torque that acts on the coil is
AZeroB2πriBCπr2iBD2πr2iBA current i flows in a circular coil of radius r. If the coil is placed in a uniform magnetic field B with its plane parallel to the field, magnitude of the torque that acts on the coil is
AZeroB2πriBCπr2iBD2πr2BA flat coil of n turns, area A and carrying a current I is placed in a uniform magnetic field of magnitude B. The plane of the coil makes an angle θ with the direction of the field. The torque acting on the coil is
ABInAsinθBnAIBsinθCBInAcosθDBIn2Acosθ
A current carrying circular coil, suspended freely in a uniform external magnetic field orients to a position of stable equilibrium. In this state :
View SolutionThere is a flat circular current coil placed in a uniform magnetic field in such a manner that its magnetic moment is opposite to the direction of the magnetic field. The coil is
View SolutionA current carrying rectangular coil is placed in a uniform magnetic field. In which orientation, the coil will not tend to rotate
View SolutionA planar coil having 12 turns carries 15 A current. The coil is oriented with respect to the uniform magnetic field →B=0.2ˆi T such that its directed area is →A=−0.04ˆi m. The potential energy of the coil in the given orientation
View SolutionA rectangular coil of area→Aand number of turns n is placed in a uniform magnetic field vecB. If the coil carries a current I, then the torque acting on the coil is given by
View Solution