In the arrangement shown in figure, light of wavelength 6000Å is incident on slits S1 and S2 have been opened such that S3 is the position of first maximum above the central maximum and S4 is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S1 and S2 and O' is equidistant from S3 and S4. Then intensity of inciden light is I0
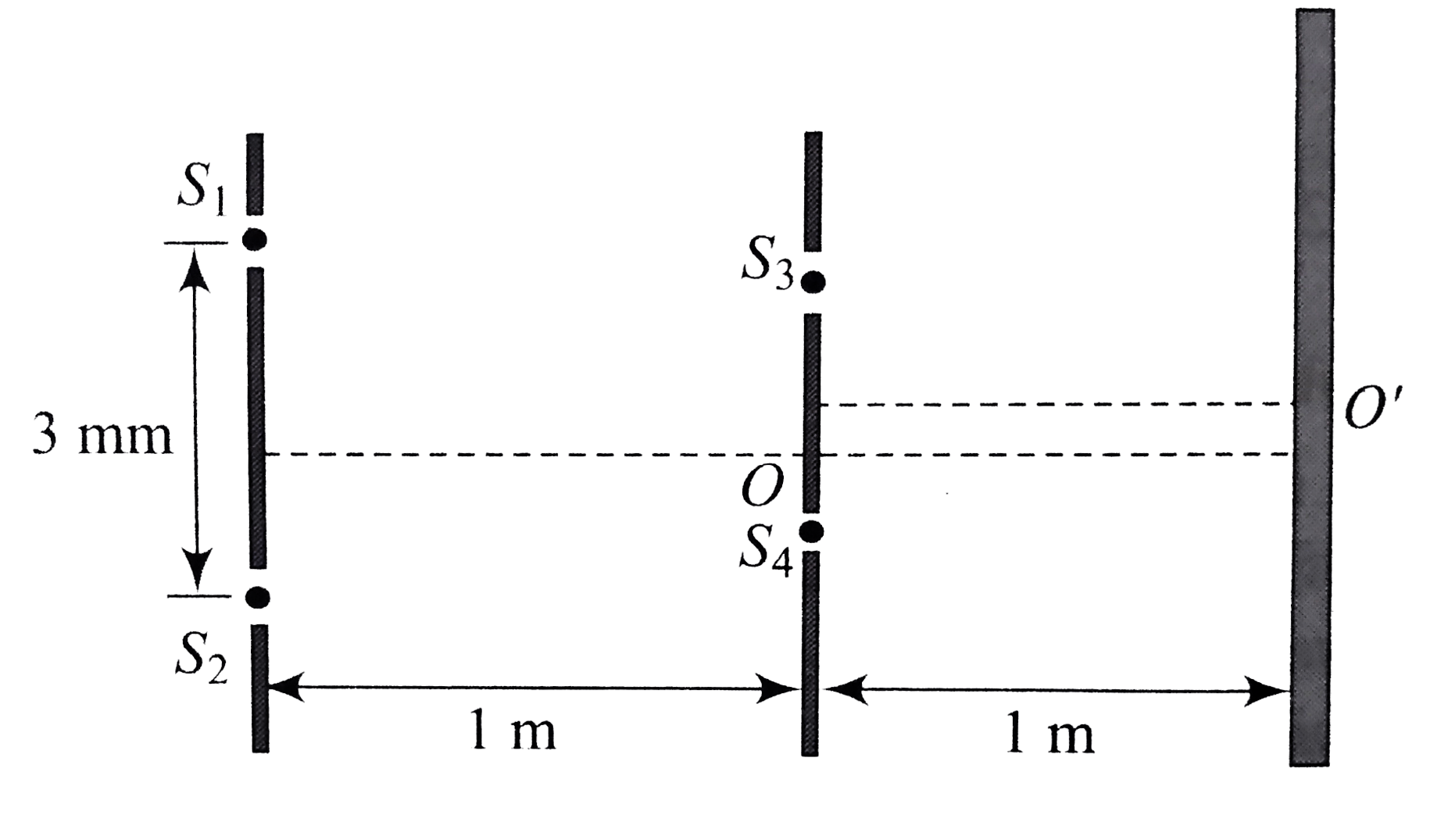
Find the intensity at O' (on the screen).
4.5I0
3I0
2I0
5I0
The correct Answer is:b
From the given condition,
OS3=Dλd=1×6×10−73×10−3=2×10−4m
Let the reaching from S1 and S2 to S4 and phase difference ϕ and intensity of incident light is I0. Resultant intensity at S4 is
(i) I=4I0cos2(ϕ2)
As I=I0, hence
I04I0=cos2(ϕ2)
⇒cos(ϕ2)=12=cos60∘
(ii) ϕ=2π3,ϕ=2π3Δx⇒Δx=λ3
(iii) As Δx=λ3 , using Δxd=yD
y=ΔxDd⇒OS4=Dλ3d
Therefore, S3S4=OS3+OS4=43dλd=83×10−4m
Now, resultant wave coming out of S3 has intensity 4I0 and waves coming out of S4 have intensity I0.
Phase difference at S3=2π
Phase difference at S4=2π/3
These phase diffference ara ralative to the ligth incident on slit S1 and S2.
Now, S3 and S4 are secondary sources of ligth.
Phase difference at O' =4π/3, equal to inital phase difference between the ligth reaching at O', i.e.,
2π2π3=4π3
Let intensity at O' be I'. Then
I'=I0+4I0+2√I0√4I0cos(4π3)
=5I0+4I0cos(π+π3)=3I0
For the brightest fringe, phase difference =2np,n=0,±1,±2,...
Let I'' be the intensity of the brightest fringe.
I''=I0+4I0+2√I0√4I0cosϕ (where cosϕ=1).
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Integer
3 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Archives
4 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning
13 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
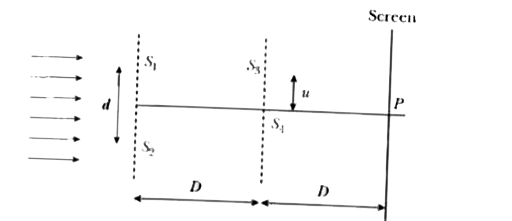
In the arrangement shown in the following figure, the wavelength of light used is λ. The distance between slits S1 and S2 is d (« D). The distance between S3 and S4 is u=λD/3d. If the ratio of maximum to minimum intensity observed on screen is k. Find k.
View SolutionIn an interference experiment, the two slits S1andS2 are not equidistant from the source S. then the central fringe at 0 will be
View Solution
Knowledge Check
In the arrangement shown in figure, light of wavelength 6000Å is incident on slits S1 and S2 have been opened such that S3 is the position of first maximum above the central maximum and S4 is the closest position where intensity is same as that of the ligth used, below the central maximum Point O is equidistant from S1 and S2 and O' is equidistant from S3 and S4. Then intensity of inciden light is I0
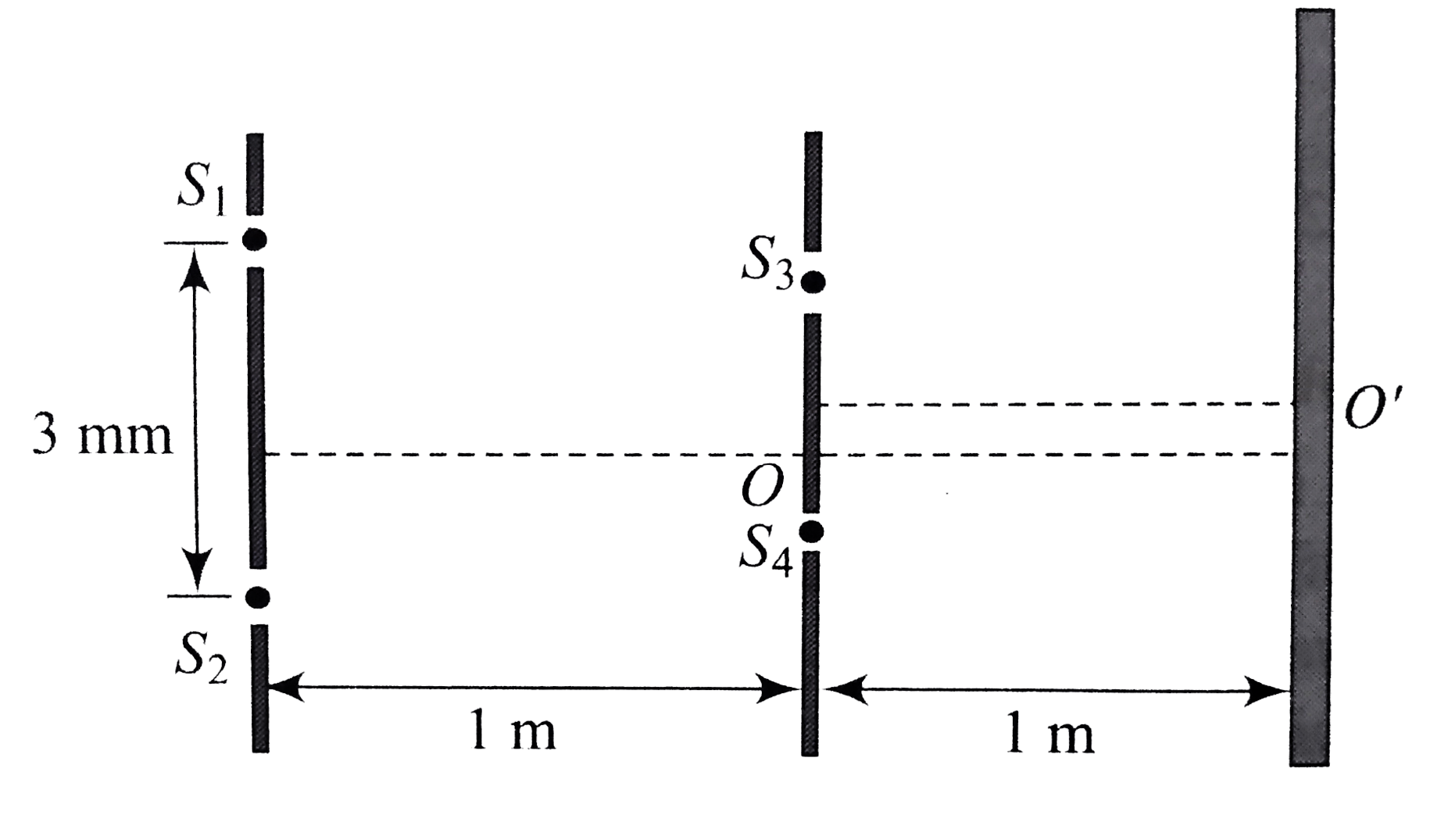
Find the intensity of the brightest fringe.A9I0B5I0C3I0D7I0In YDSE monochromatic light of wavelength 600 nm incident of slits as shown in figure.
_PHY_E01_002_Q01.png)
If S1S2=3mm,OP=11mm thenAIf α=0.36π degree then destructive interface at point PBIf α=0.36π degree then constructive interfaces at point OCIf α=0 then constructive interface at ODFringe width depends an αIn a Young's double-slit experiment set up, source S of wavelength 6000Å illuminates two slits S1 and S2 which act two coherent sources. The sources S oscillates about its shown position according to the eqation y=1+cosπt, where y is in millimeter and t in second.
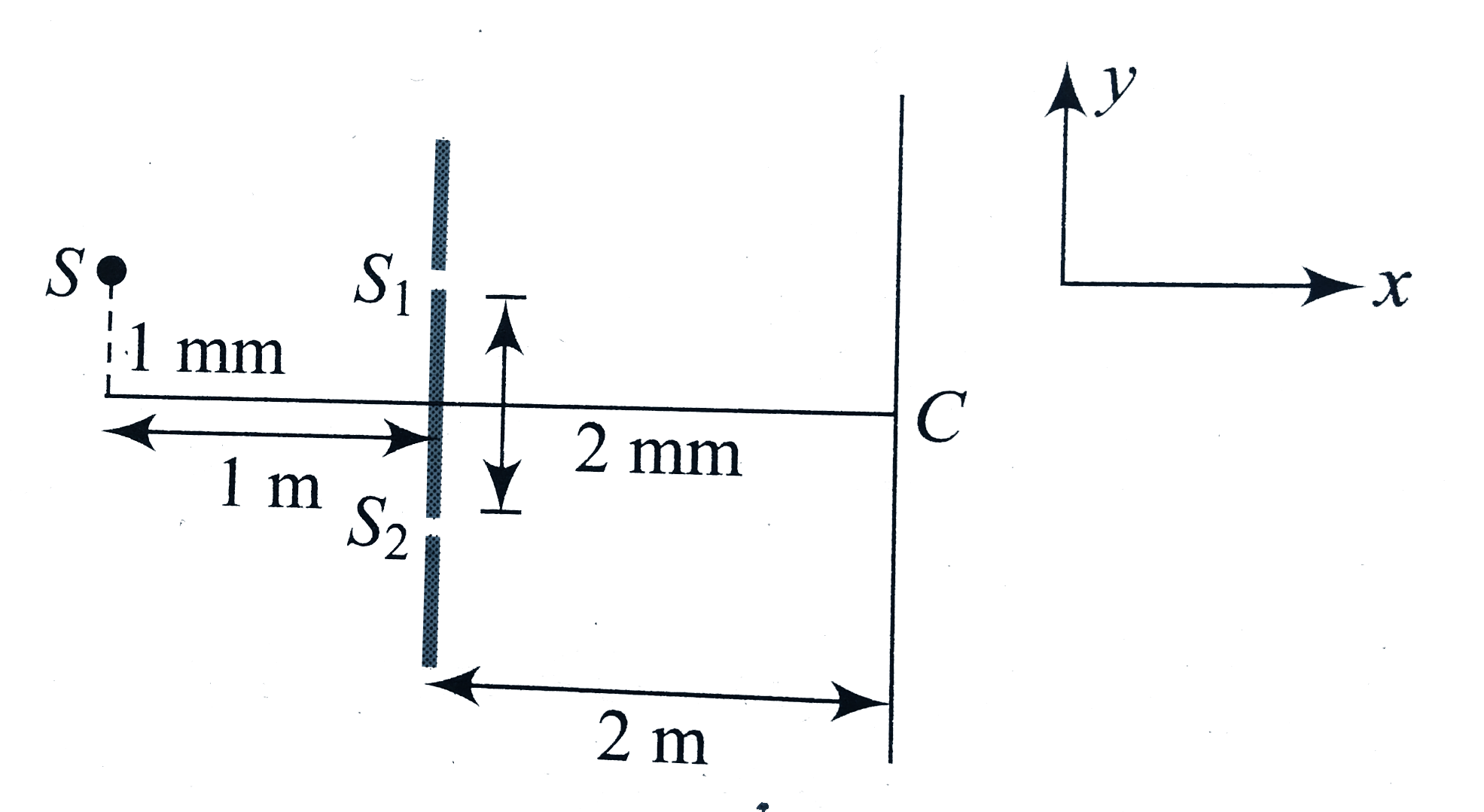
At t=2 s, the position of central maxima isA2 mm above CB2 mm below CC4 mm above CD4 mm below C
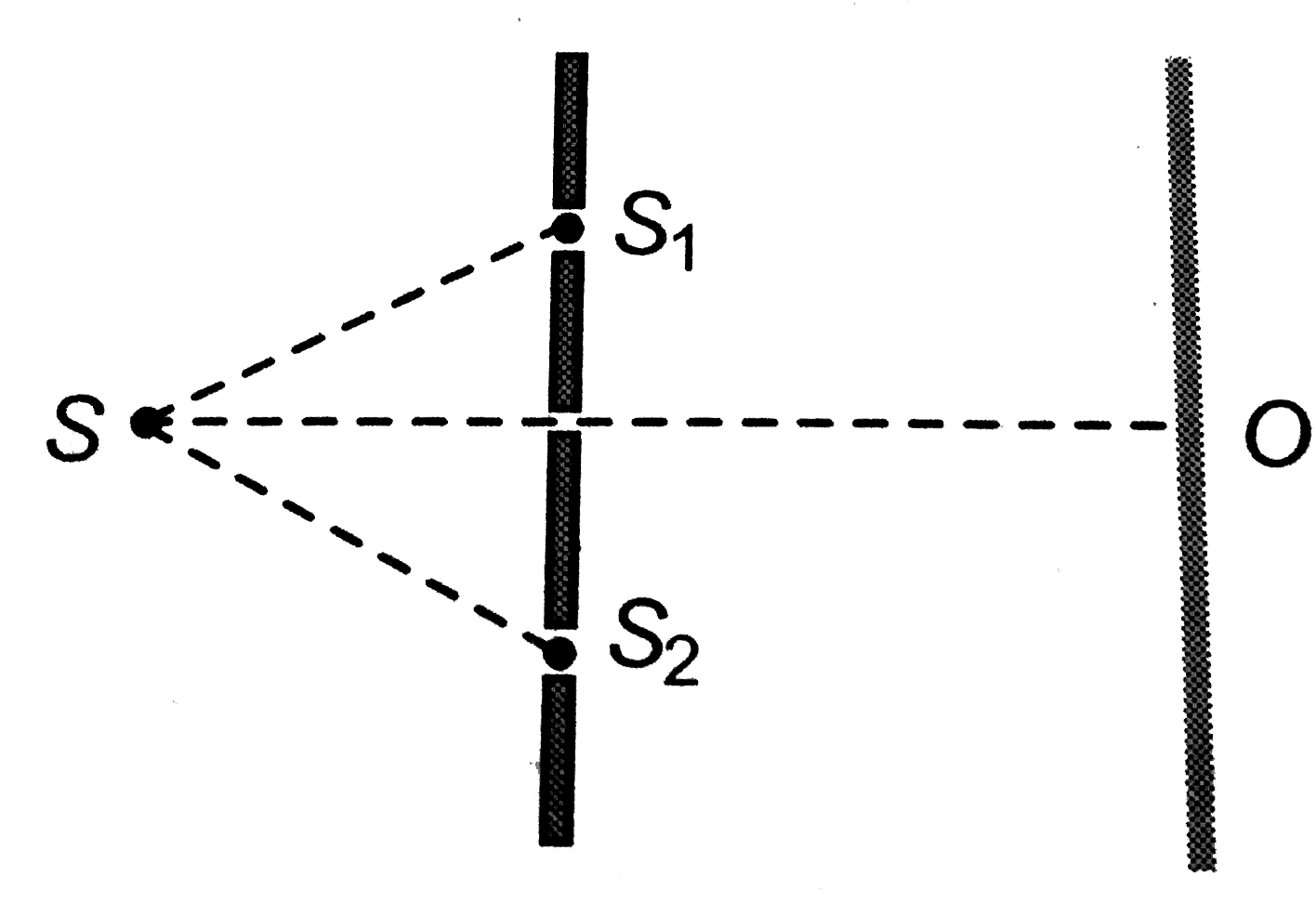
In the set up shown in figure, the two slits S1 and S2 are not equidistant from the slit S. The central fringe at O is then
View SolutionLight of wavelength 6000Å is normally incident on a slit. Angular position of second minimum from central maximum is 30∘. Width of the slit should be –
View SolutionA coherent light is incident on two paralle slits S1andS2. At a point P1 the frings will be dark if the phase difference between the rays coming from S1andS2 is
View SolutionIn fig., light of wavelength λ=5000Å is incident on the slits (in a horizontally fixed place).
Here, d=1mm and D=1m
Take origin at O and XY plane as shown in the figure. The screen is released from rest from the initial position as shown
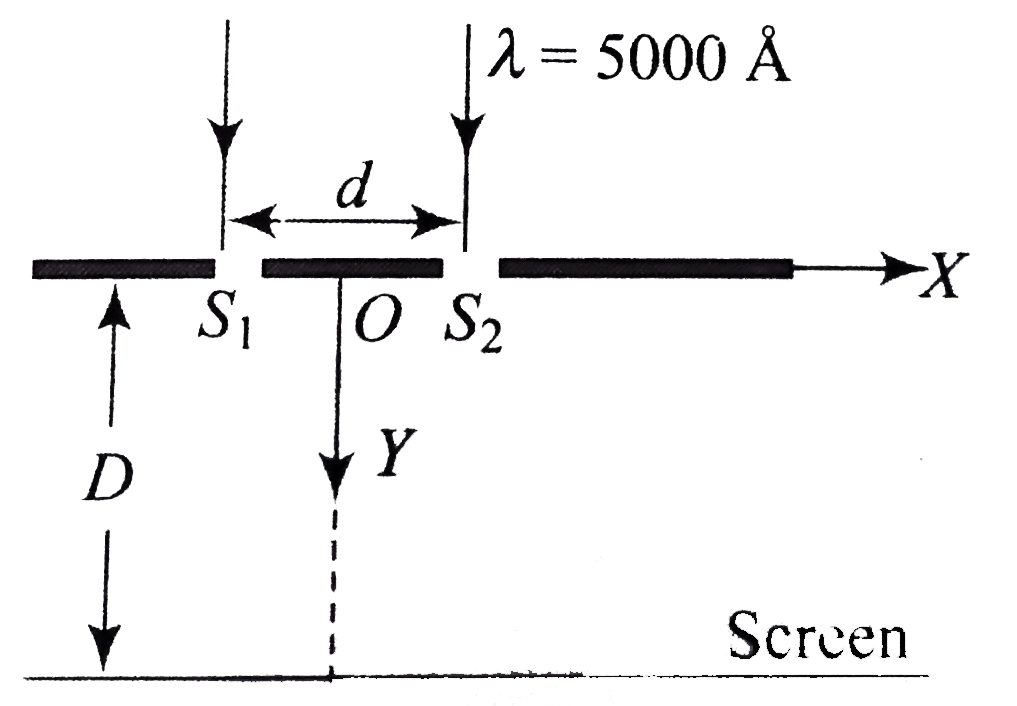
The velocity of central maxima at t=5s is
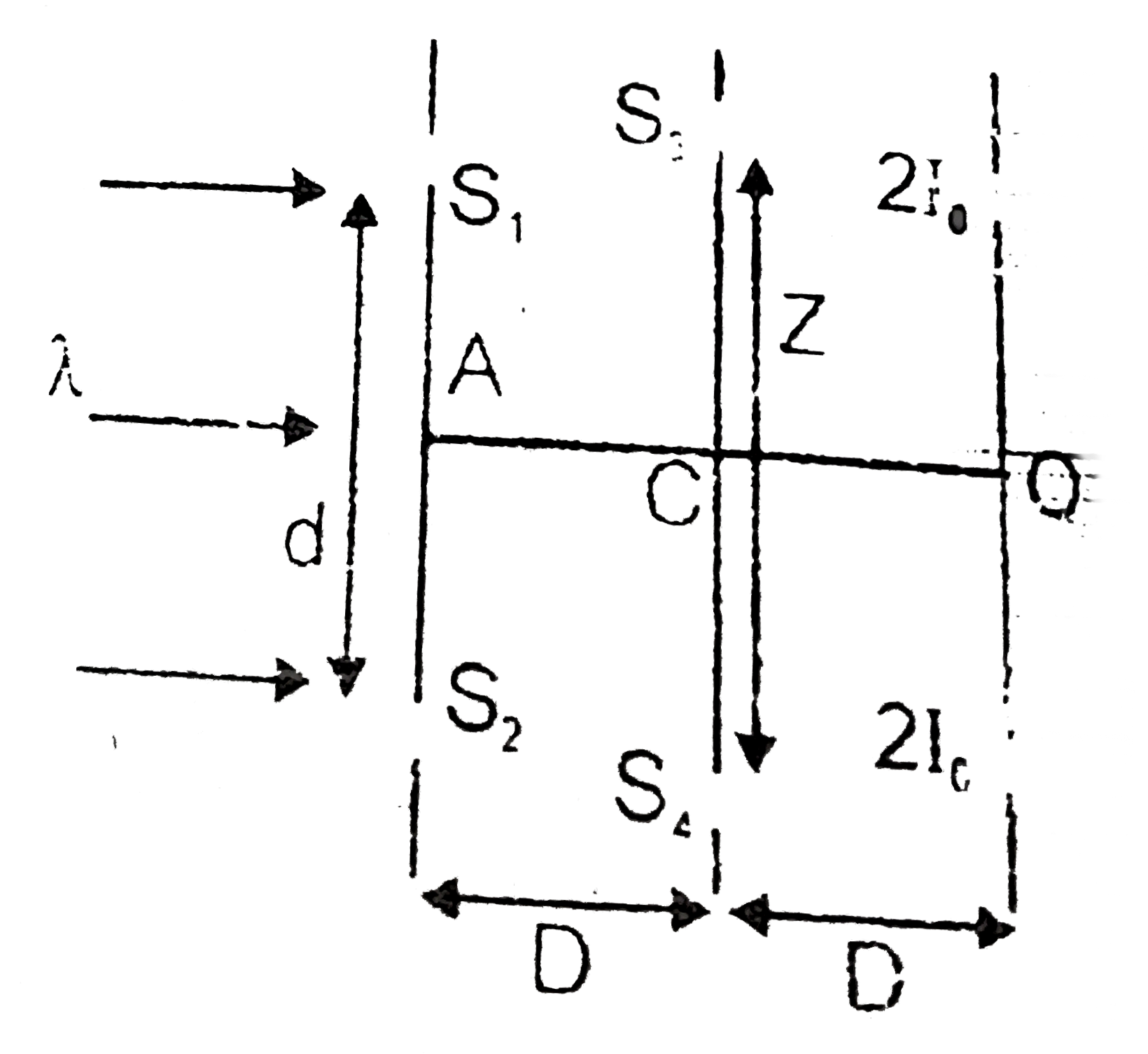
View SolutionIn arrangement shown in figure, plane wavefront of monochromatic light of wavelength λ is incident on identical slits S1 and S2. There is another pair of indentical slits S3 and S4 which are having separation Z=λD2d Point O is on the screen at the common perpendicular bisector of S1S2 and S3S4.I1 is the intensity at point O Now the board having slits S3S4 is moved upward parallel to itself and perpendicular to line AO till slit S4 is on line AO and it is observed that now intensity at point O is I2 then I2I1 is :
View Solution
