In figure, a screen is placed normal to the line joining the two point coherent sources S1 and S2. The interference pattern consists of concentric circles.
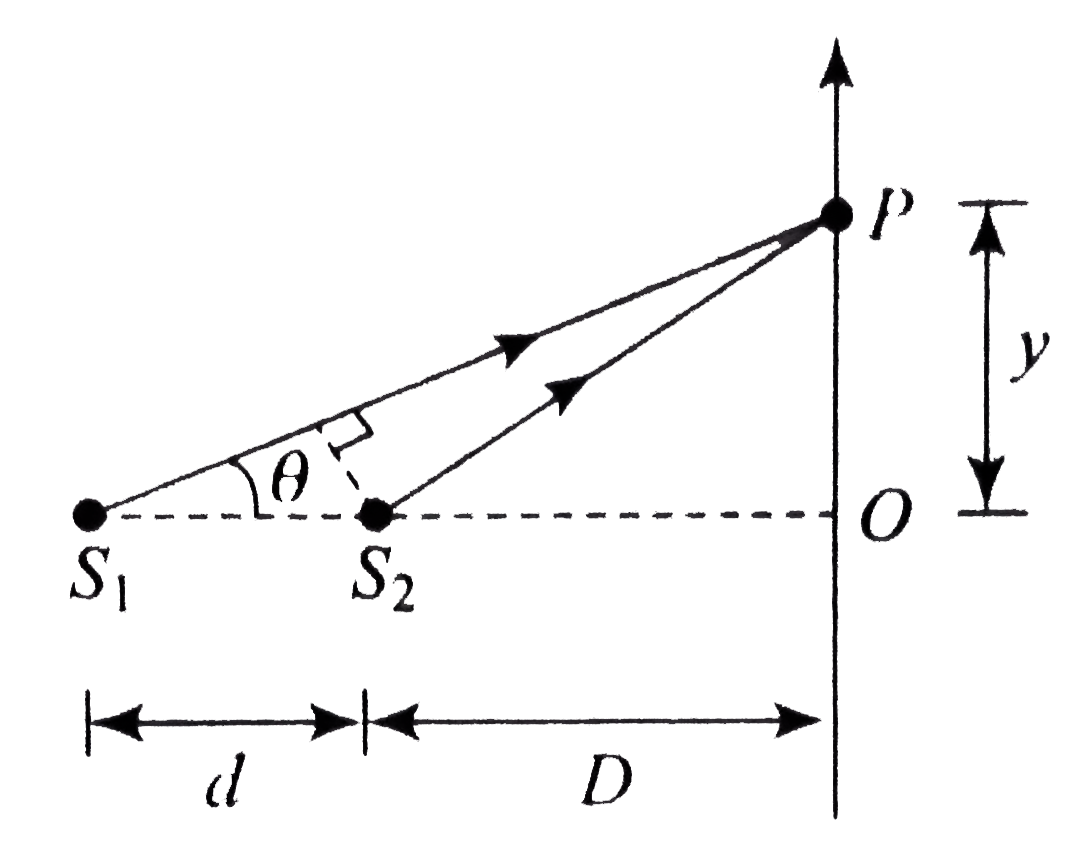
If d=0.5mm,λ=5000Å and D−10cm, find the value of n for the closest second bright ring.
888
830
914
998
The correct Answer is:d
The optical path difference at P is
Δx=S1P−S2P=cosθ
∵cosθ=1−θ22 for small θ
∴Δx=d(1−θ22)
=d[1−y22D2], where D+d=D
For nth maxima,
⇒Δx=nλ
d[1−y22D2]=nλ
y = radius of the nth brigth ring
=D√2(1−nλd)
At the central maxima, θ=0
Δx=d=nλ
⇒n=dλ=0.50.5×10−3=1000
Hence, for the closest second brigth ring, n=998.
Putting values, r=6.32cm.
Topper's Solved these Questions
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Integer
3 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Archives
4 Videos
- WAVE OPTICSBOOK - CENGAGE PHYSICSCHAPTER - WAVE OPTICSEXERCISE - Assertion- Reasoning
13 Videos
- THERMODYNAMICSBOOK - CENGAGE PHYSICSCHAPTER - THERMODYNAMICSEXERCISE - QUESTION BANK
34 Videos
- WAVESBOOK - CENGAGE PHYSICSCHAPTER - WAVESEXERCISE - QUESTION BANK
38 Videos
Similar Questions
In the figure shown, a screen is placed normal to the line joining the two point coherent sources S1andS2. The interference pattern consists of concentric circles.
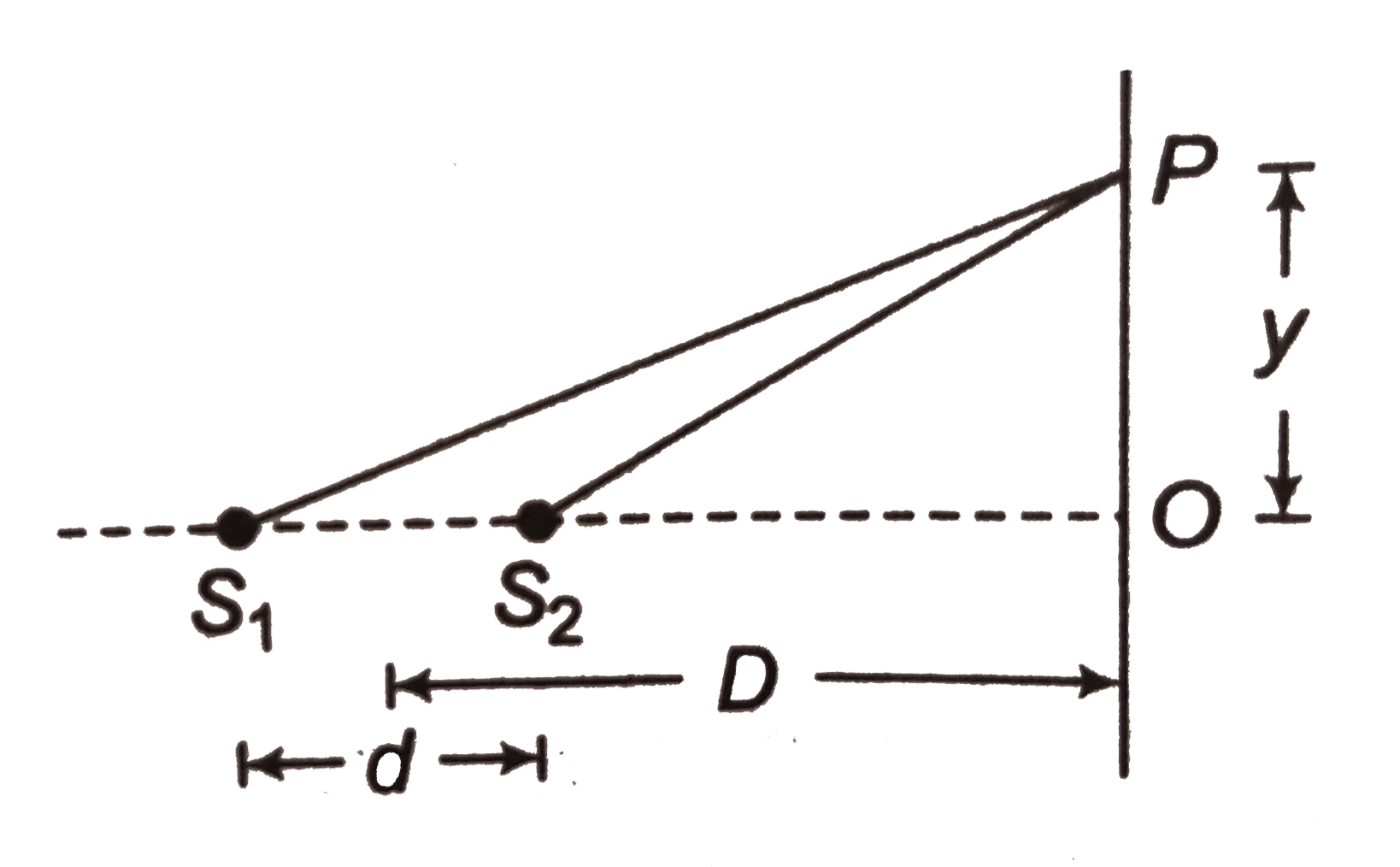
(a)Find the radius of the nth bright ring.
(b) If d=0.5 mm, λ=5000Å and D=100 cm, find the radius of the closest second bright ring.
(c) Also, find the value of n for this ring.
View SolutionIn Young's double-slit experiment,, monochromatic light of wavelength 630 nm illuminates the pair of slits and produces an interference pattern in which two consecutive bright fringes are separated by 8.1mm. Another source of monochromatic light produces the interference pattern in which the two consecutive bright fringes are separated by7.2 mm. find the wavelength of light from the second source.
What is the effect on the interference fringes if the monochromatic source is replaced by a source of white light ?
View Solution
Knowledge Check
In figure, a screen is placed normaol to the line joining the two point coherent sources S1 and S2. The interference pattern consists of concentric circles.
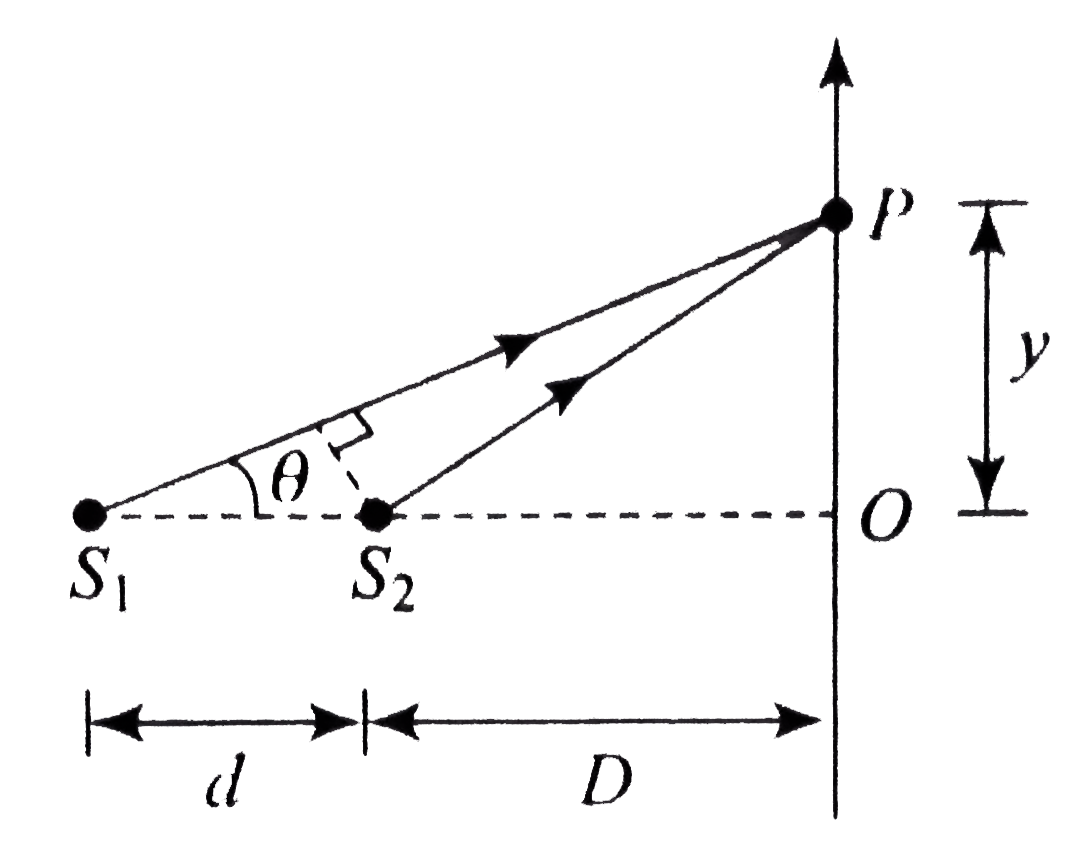
Find the radius of the nth bright ringAD√1(1−nλd)BD√2(1−nλd)C2D√2(1−nλd)DD√2(1−nλ2d)In figure, a screen is placed normaol to the line joining the two point coherent sources S1 and S2. The interference pattern consists of concentric circles.
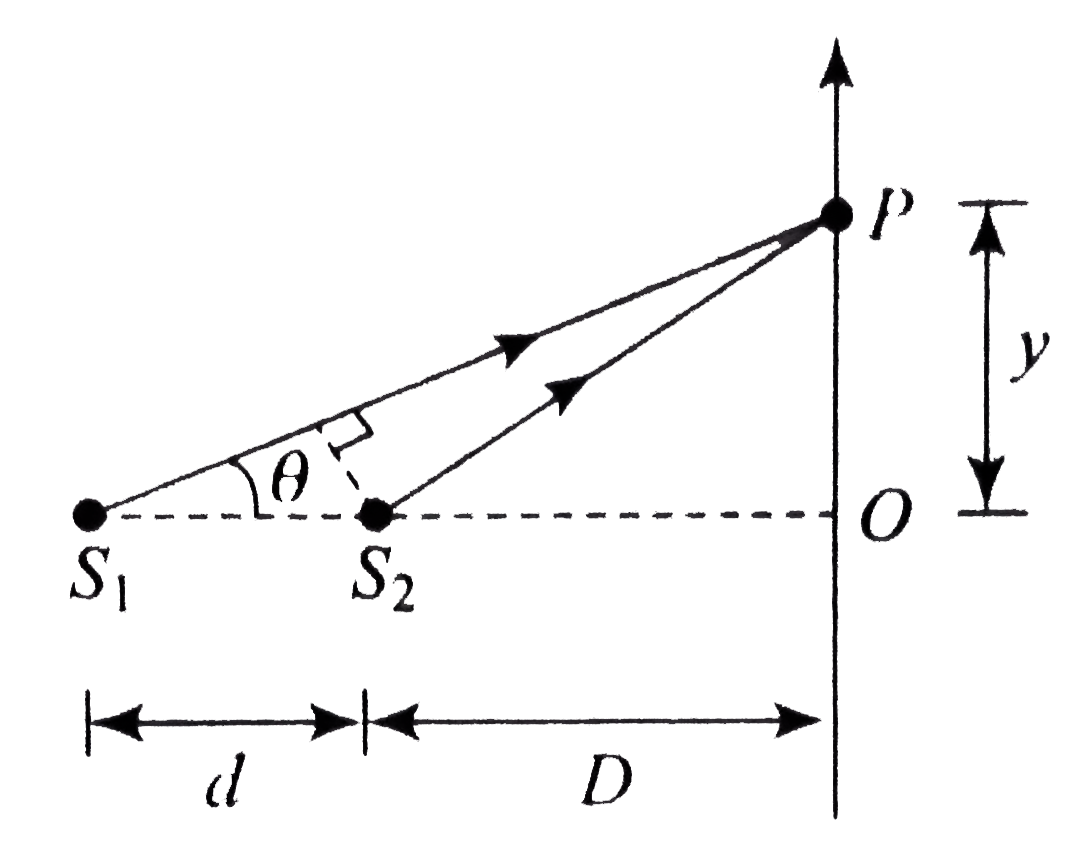
Also, find the value of radius for this ring.A6.32 cmB5.52 cmC4.7 cmD3.25 cmIn the figure , the intensity of waves arriving at D from two coherent soucrces s1ands2isI0. The wavelength of the wave is λ=4m . Resultant intensity at D will be
 A4l0Bl0C2l0Dzero
A4l0Bl0C2l0Dzero
In Young's double-slit experiment, monochromatic light of wavelength 630 nm illuminates the pair of slits prodcues an interference pattern in which two consecutive bright fringes are separated by 8.1 mm. Another source of monochromatic light produces the interference pattern in which the two consecutive bright fringes are separated by 7.2 mm. Find the wavelength of light for the second source.
What is the effect on the interference fringes if the monochromatic source is replaced by a source of white light?
View SolutionTwo coherent point sources S1andS2 are separated by a small distance 'd' as shown. The fringes obtained on the screen will be :

View SolutionTwo coherent point sources S1 and S2 are separated by a small distance d as shown. The fringes obtained on the screen will be

View SolutionTwo waves of equal amplitude a from two coherent sources (S1&S2) interfere at a point R such that S2R−S1R=1.5λThe intensity at point R Would be
View SolutionIn a biprism experiment, the distance between the two virtual sources is 0.1 mm and the screen is placed at 100 cm from the slits. If the wavelength of light used is 5000 Å, then the distance of the 4th bright band from the central bright band will be
View Solution
