Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
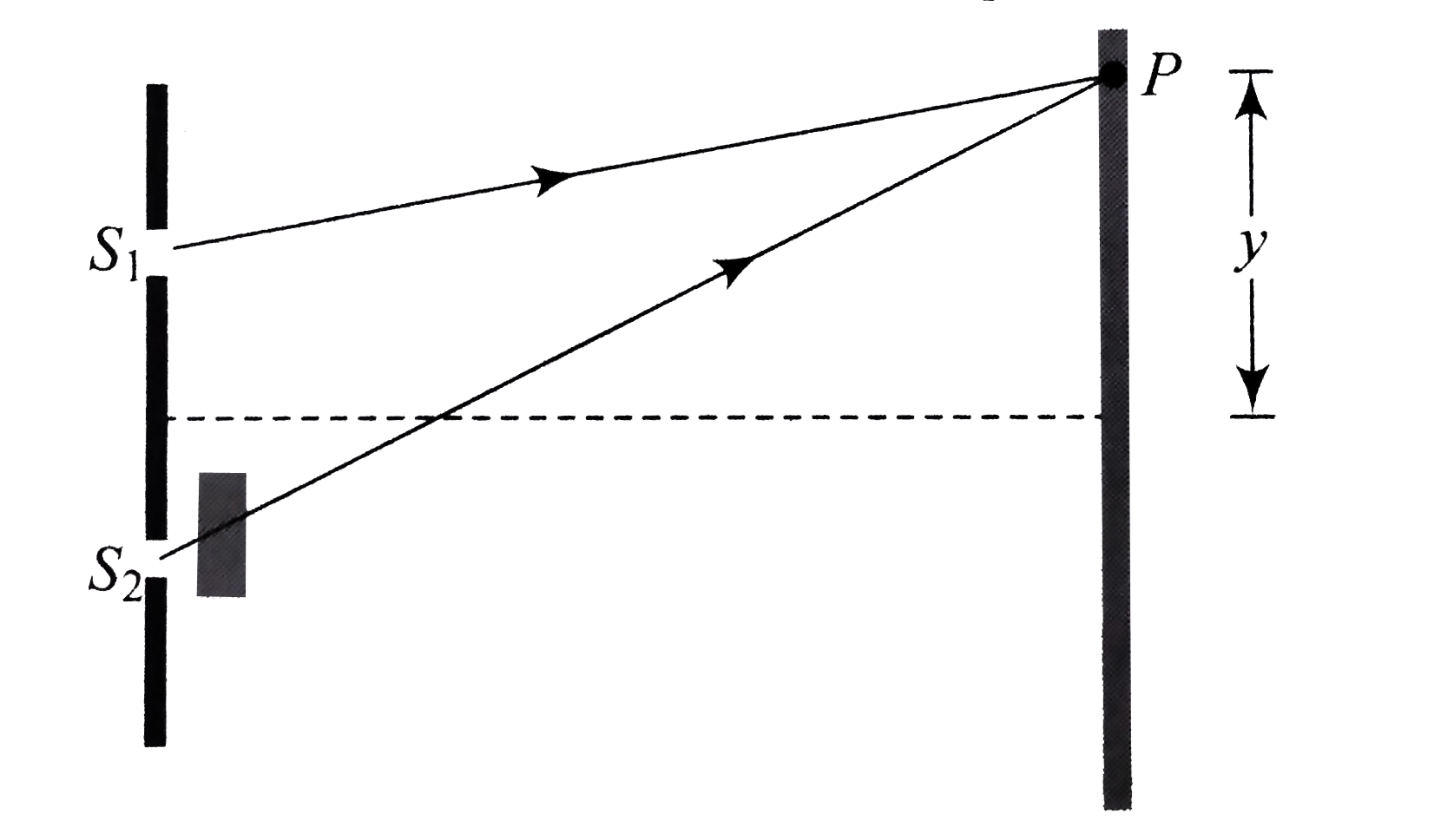
Young's double-slit experiment setup with ligth of wavelength lambda = 6000 Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction eta of the incident light to pass through) and thickness 8000 Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change. The value of eta is
The value of η is

A

0.21

B

0.42

C

0.12

D

0.5

Video Solution
Doubtnut Promotions Banner
Text Solution
Verified by Experts

The correct Answer is:a

Without inserting the slab, path difference at P,
Δx=ydD=0.15×10−3×2×10−32=1.5×10−7m
Corresponding phase difference at P,
ϕ=(2πλ)(Δx)
=(2π6000×10−10)(1.5×10−7)=π2
ϕ2=π4
Therefore, intensity at P,
I=4I0cos2(ϕ/2)=2I0
Phase difference after placing to glass sheet,
ϕ'=ϕ+2πλ(μ−1)t
=π2+2π6000×10−10(1.5−1)(8000×10−19)
=11π6
Now, the intensity at P is,
I'=I0+ηI0+2√ηI20cos.11π6=2I0 (given)
solving this equation, we get η=0.21.
ocr_image

Updated on:21/07/2023
|
Class 12PHYSICSWAVE OPTICS

Topper's Solved these Questions

Similar Questions

Knowledge Check

  • Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
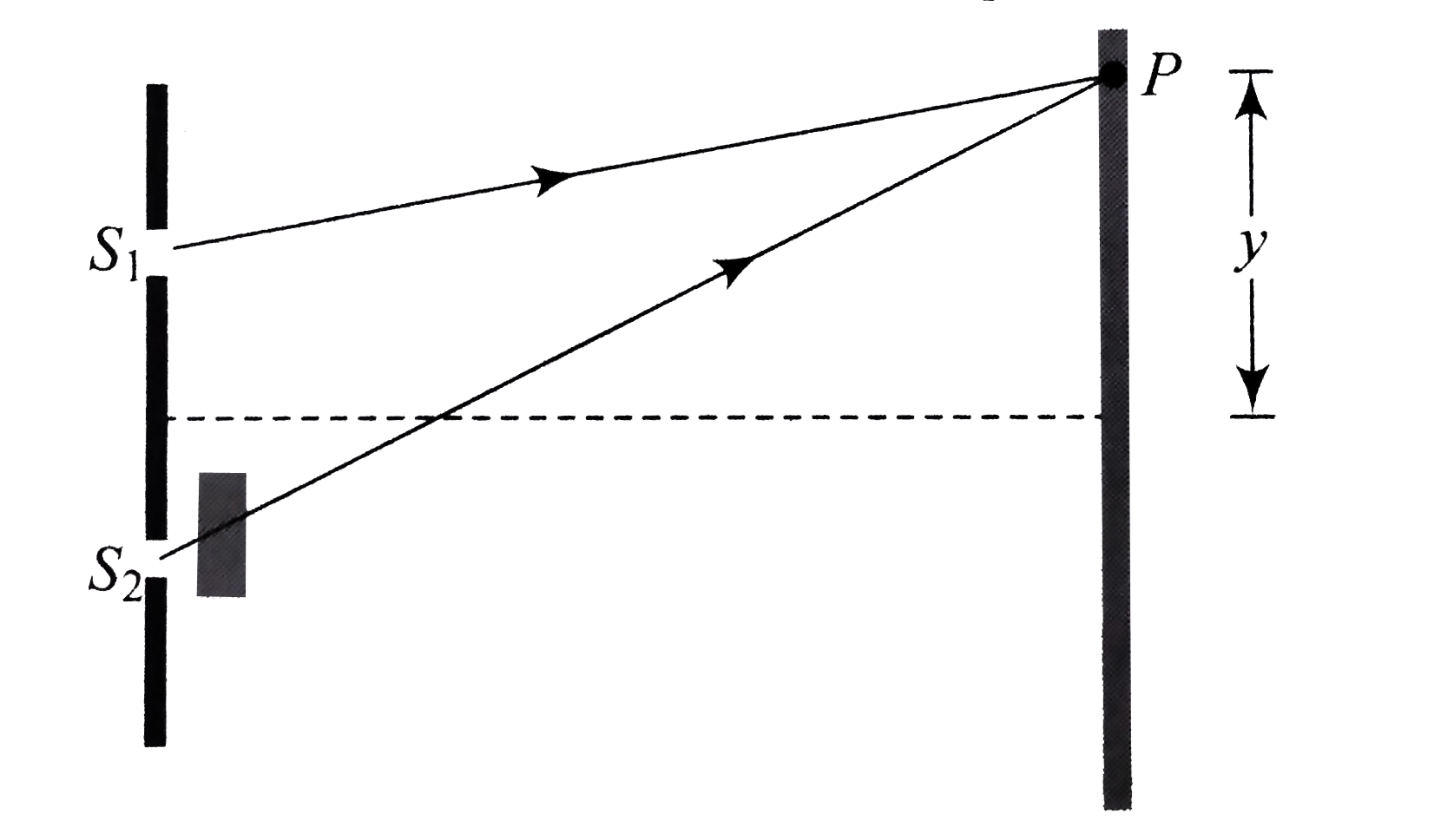
    ocr_image
    The phase difference at point P without inserting the slab is

    A3π/4
    Bπ/4
    Cπ/2
    Dπ/3

  • Young's double-slit experiment setup with ligth of wavelength λ=6000Å, distance between two slit in 2 mm and distance between the plane of slits and the screen. Is 2 m. The slits are of equal intensity. When a sheet of glass of refractive index 1.5 (which permtis only a fraction η of the incident light to pass through) and thickness 8000Å is placed in front of the lower slit, it is observed that the intensity at a point P, 0.15 mm above the central maxima, does not change.
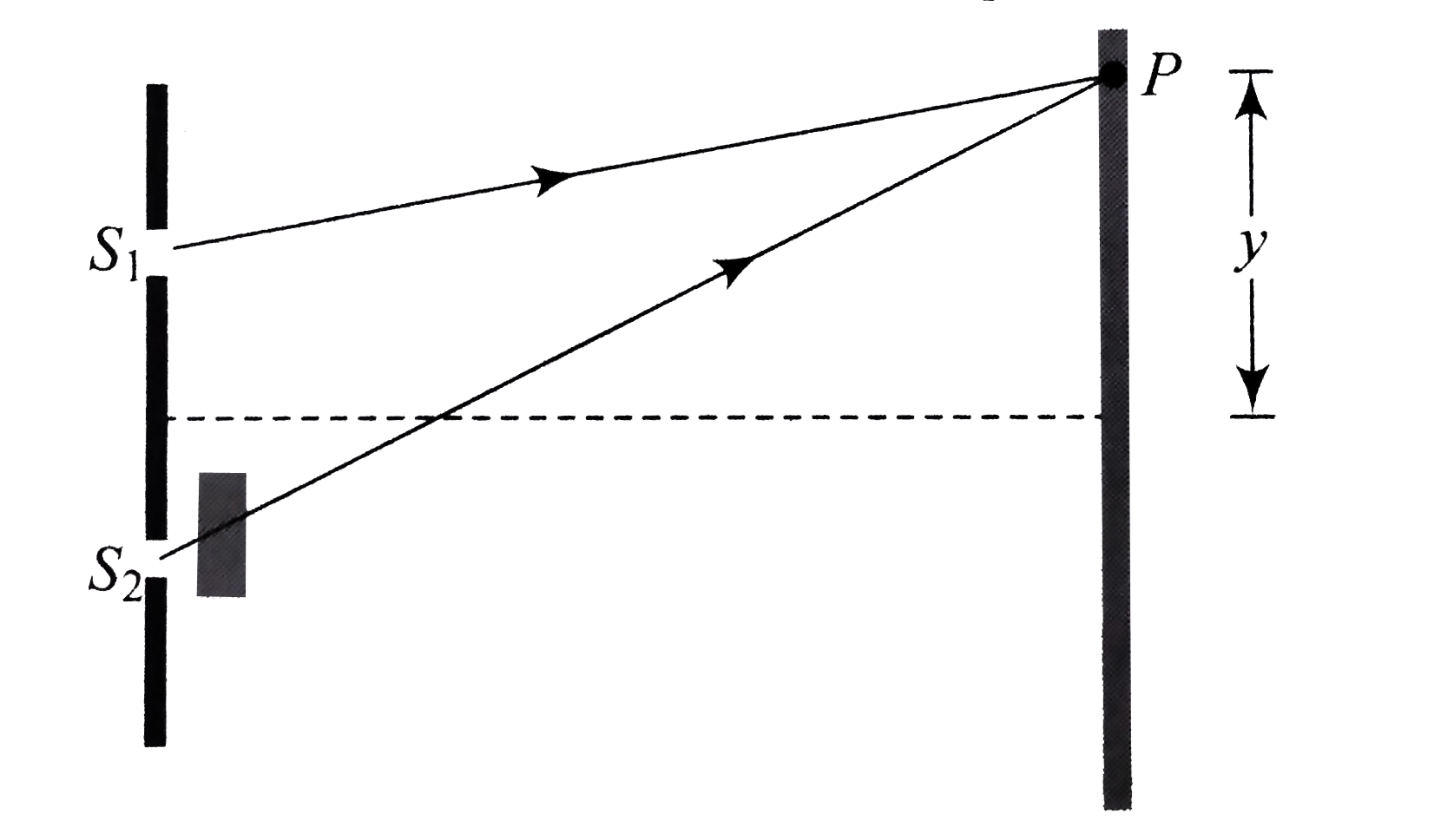
    ocr_image
    Intensity at point P is

    A3I0
    BI0
    C2I0
    D8I0

  • In a Young's double slit experiment, bi-chromatic light of wavelengths 400 nm and 560 nm are used. The distance between the slits is 0.1 mm and the distance between the plane of the slits and the screen is 1m. The minimum distance between two successive regions of complete darkness is

    A4 mm
    B5.6 mm
    C14 mm
    D28 mm