A very small sphere of mass 80g having a charge q is held at a height of 9m vertically above the center of a fixed conducting sphere of radius 1m, carrying an equal charge q. When released, it falls until it is repelled back just before it comes in contact with the shpere as shown in Fig. 47. Calculate the charge q.[g=10ms−2].
To solve the problem, we need to analyze the forces acting on the small sphere and use the concept of energy conservation. We will calculate the charge q on the small sphere.
Step 1: Understand the Forces Acting on the Sphere
When the small sphere is released, it experiences two forces:
1. Gravitational force acting downward, Fg=mg
2. Electrostatic force due to the repulsion from the conducting sphere.
Step 2: Calculate the Gravitational Force
Given:
- Mass m=80g=0.08kg
- Acceleration due to gravity g=10m/s2
The gravitational force is:
Fg=mg=0.08kg×10m/s2=0.8N
Step 3: Determine the Electrostatic Force
The electrostatic force Fe between two charges q at a distance r is given by Coulomb's law:
Fe=k⋅q2r2
where k=14πϵ0.
At the point just before the small sphere touches the conducting sphere, the distance r between the centers of the two spheres is 9m−1m=8m.
Thus, the electrostatic force becomes:
Fe=k⋅q2(8m)2=k⋅q264
Step 4: Set Up the Equation of Motion
At the point just before it is repelled, the gravitational force equals the electrostatic force:
Fg=Fe
0.8=k⋅q264
Step 5: Solve for Charge q
Rearranging the equation gives:
k⋅q2=0.8⋅64
k⋅q2=51.2
Now, substituting k=14πϵ0 (where ϵ0≈8.85×10−12C2/N⋅m2):
q24πϵ0=51.2
q2=51.2⋅4πϵ0
q2=51.2⋅4⋅π⋅8.85×10−12
Calculating this gives:
q2≈51.2⋅4⋅3.14⋅8.85×10−12≈5.7×10−10
Taking the square root:
q≈√5.7×10−10≈2.39×10−5C
Final Answer
The charge q on the small sphere is approximately:
q≈2.39×10−5C
When the small sphere is released, it experiences two forces:
1. Gravitational force acting downward, Fg=mg
2. Electrostatic force due to the repulsion from the conducting sphere.
Given:
- Mass m=80g=0.08kg
- Acceleration due to gravity g=10m/s2
Fg=mg=0.08kg×10m/s2=0.8N
The electrostatic force Fe between two charges q at a distance r is given by Coulomb's law:
Fe=k⋅q2r2
where k=14πϵ0.
Fe=k⋅q2(8m)2=k⋅q264
At the point just before it is repelled, the gravitational force equals the electrostatic force:
Fg=Fe
0.8=k⋅q264
Rearranging the equation gives:
k⋅q2=0.8⋅64
k⋅q2=51.2
Now, substituting k=14πϵ0 (where ϵ0≈8.85×10−12C2/N⋅m2):
q24πϵ0=51.2
q2=51.2⋅4πϵ0
q2=51.2⋅4⋅π⋅8.85×10−12
Calculating this gives:
q2≈51.2⋅4⋅3.14⋅8.85×10−12≈5.7×10−10
Taking the square root:
q≈√5.7×10−10≈2.39×10−5C
The charge q on the small sphere is approximately:
q≈2.39×10−5C
Topper's Solved these Questions
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - Examples
9 Videos
- ELECTRIC POTENTIALBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC POTENTIALEXERCISE - Exercise 3.1
23 Videos
- ELECTRIC FLUX AND GAUSS LAWBOOK - CENGAGE PHYSICSCHAPTER - ELECTRIC FLUX AND GAUSS LAWEXERCISE - MCQ s
38 Videos
- ELECTRICAL MEASURING INSTRUMENTSBOOK - CENGAGE PHYSICSCHAPTER - ELECTRICAL MEASURING INSTRUMENTSEXERCISE - M.C.Q
2 Videos
Similar Questions
A small sphere A of radius r and carrying a charge q is enclosed by a concentric spherical shell B of radius 2r carrying a charge 29. When A and B are connected by a conducting wire, the charge will flow from __________ to __________ .
View SolutionTwo identical charges repel each other with a force equal to 1mg wt when they are 0.6m apart in air.The value of each charge is (g=10ms−2 )
View Solution
Knowledge Check
Calculate amount of charge flow, when a conducting sphere of radius R and carrying a charge Q, is joined to an uncharged conducting sphere of radius 2R.
AQ4BQ3CQ2D2Q3An isolated conduction sphere sphere whose radius R=1 m has a charge q=19nC. The energy density at the surface of the sphere is
Aε02J/m3Bε0J/m3C2ε0J/m3Dε0/3Jm3If a small sphere of mass m and charge q is hung from a silk thread at an angle θ with the surface of a vertical charged conducting plate, then for equilibrium of sphere, the surface charge density of the plate is
Aε0(mgq)tanθBε0(2mgq)tanθCε0(mgq)tanθDε0(mg3q)tanθ
When a small uncharged conducting ball of radius a=1cm and mass m=50g is dropped from a height h above the center of another large conducting sphere of radius b(=1m) having charge Q(=(100μC). It rises to a height h1(=2m) after the collision. Find the value of h. Assume that during the impact there is no dissipation of energy.
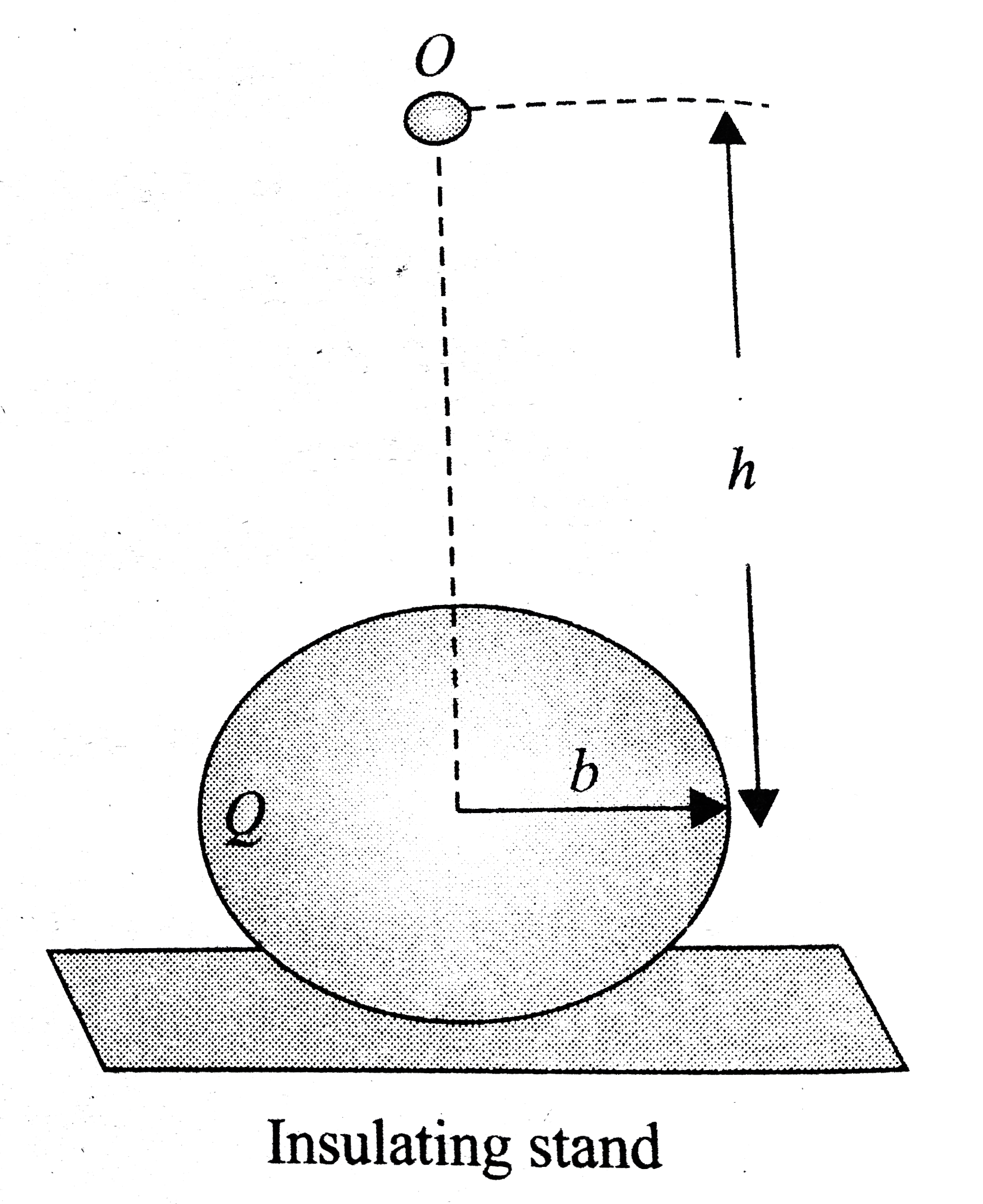 .
.
View SolutionTwo particles having positive charge +Q and +2Q are fixed at equal distance x from centre of a conducting sphere having zero net charge and radius r as shown . Initially the switch S is open. After the switch S is closed, the net charge flowing out of sphere is (P−5)Qrx find P.
View SolutionA drop of water of mass m falls away from the bottom of charged conducting sphere of radius R, carrying with it a charge q1 and leaving the sphere a uniformly distributed charge q2. The kinetic energy of the drop after it has fallen height h is -
View SolutionA particle of mass 2 g and charge 1μC is held at rest on a frictionless surface at a distance of 1m from a fixed charge of 1 mC. If the particle is released it will be repelled. The speed of the particle when it is at distance of 10 m from fixed charge is :
View SolutionA small conducting sphere of radius a, carrying a charge +Q, is placed inside an equal and oppositely charged conducting shell of radius b such that their centers coincide. Determine the potential at a point which is at a distance c from center such that a<c<b.
View Solution