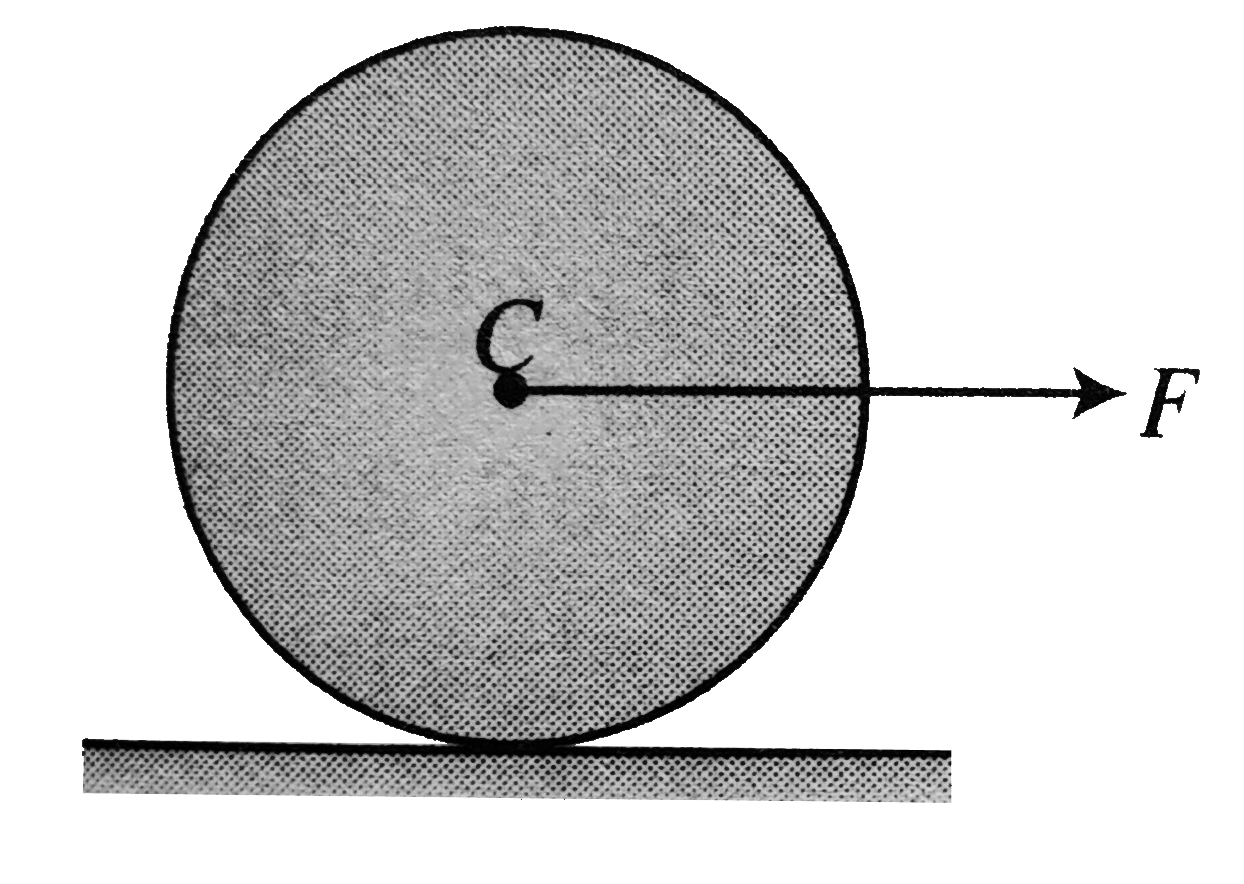
A horizontal force $F = 14 N$ acts at the centre of mass of a sphere of mass $m = 1 k g$ . If the sphere rolls without sliding, find the frictional force (in $N$ )

Video Solution

Text Solution

Verified by Experts

The correct Answer is:4

Updated on:21/07/2023

A force $F$ acts tangentially at the highest point of a disc of mass $m$ kept on a rough horizontal plane. If the disc rolls without slipping, the acceleration of centre of the disc is:

A $\frac{2 F}{3 m}$
B $\frac{10 F}{7 m}$
Czero
D $\frac{4 F}{3 m}$
A homogeneous cylinder of mass Mand radius r is pulled on a horizontal plane by a horizontal force F acting through its centre of mass. Assuming rolling without slipping, find the angular acceleration of the cylinder,
A $\frac{2 F}{3 M r}$
B $\frac{3 F}{2 M r}$
C $\frac{F}{3 M r}$
D $\frac{F}{2 M r}$
A horizontal force $F$ acts on the block of mass $m$ and the block remains stationary, thr value of friction force is.

A $μ m g$
B $μ m g - F$
CF
Dzero