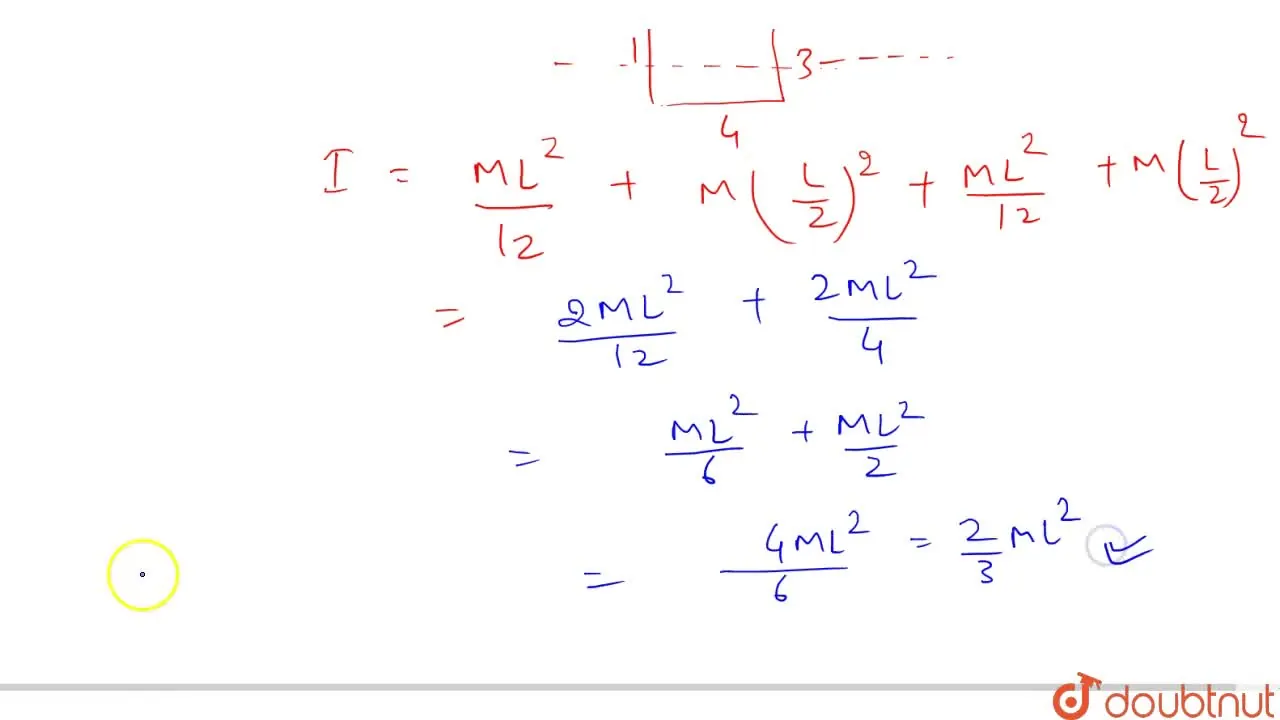
Four identical rods are joined end to end to form a square. The mass of each rod is . The moment of inertia of the square about the median line is
none of these
The correct Answer is:D
Related Playlists
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct9videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Linked Comprehension28videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Subjective13videos
RIGID BODY DYNAMICS 1
- BOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE2videos
PROPERTIES OF SOLIDS AND FLUIDS
- BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger2videos
RIGID BODY DYNAMICS 2
Similar Questions
Two identical rods are joined to form an 'X'. The smaller angle between the rods is θ. The moment of inertia of the system about an axis passing through point of intersection of the rods and perpendicular to their plane is proportional to:
View SolutionTwo identical iniform rods are joined to from a symmetrical 'X'. The smaller angle between the rods is θ. The moment of inertia of the system about an axis passing through point of intersection of the rods and perpendcular to their plane is proportional to :
View SolutionFour thin uniform rods each of length L and mass m are joined to form a square . The moment of inertia of square about an axis along its one diagonal is
View SolutionFour thin metal rods, each of mass M and length L, are welded to form a square ABCD as shown in figure. The moment of inertia of composite structure about a line which bisects rods AB and CD is
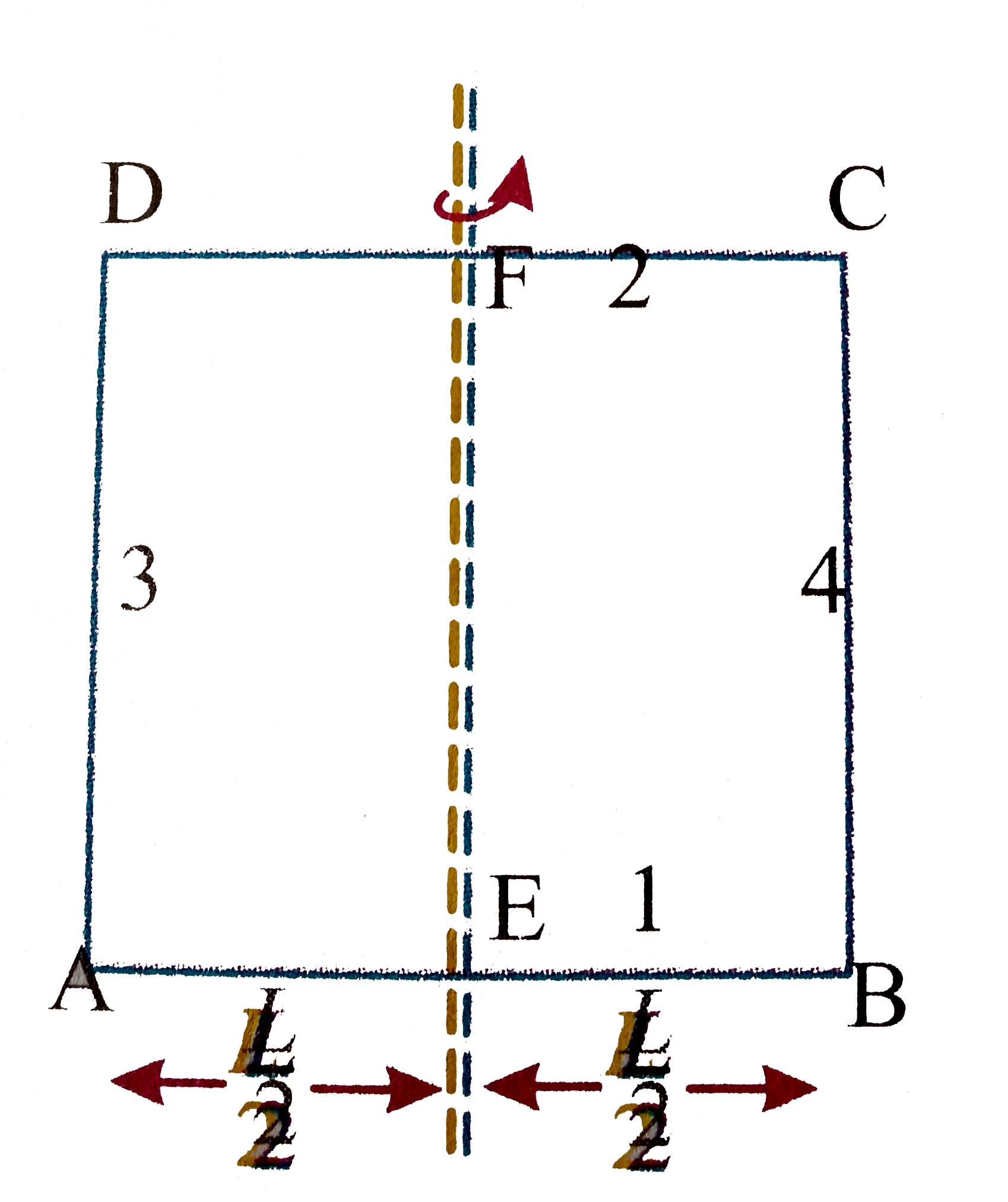 View Solution
View SolutionFour identical rods of same material are joined end to end to form a square. If the temperature difference between the ends of a diagonal is 100∘C, then the temperature difference between the ends of other diagonal will be
View SolutionTwo uniform thin identical rods AB and CD each of mass M and length L are joined so as to form a cross as shown. The moment of inertia of the cross about a bisector line EF is :-
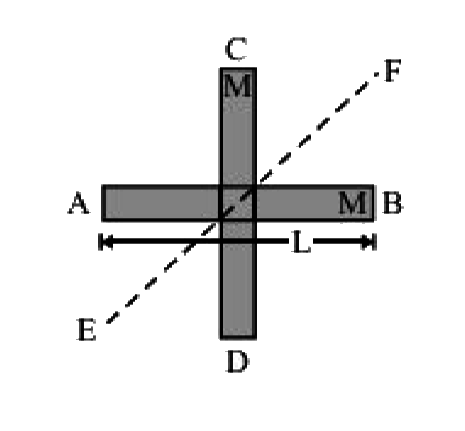 View Solution
View SolutionFour identical thin rods each of mass M and length l3, form a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is---
View SolutionFour identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
View SolutionAB and CD are two identical rods each of length l and mass m joined to form a cross. The moment of inertia of these two rods about a bisector (xy) of the angle between the rods is:
 View Solution
View SolutionThree thin rods, each of length 2 m and mass 3 kg are placed along x, y and z axes, such that one end of each rod is at the origin. The moment of inertia of this system about the x axis is
View Solution