Four identical thin rods each of mass M and length l3, form a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is---
13ml2
43Ml2
23Ml2
133Ml2
The correct Answer is:A
To find the moment of inertia of a square frame made of four identical thin rods, we can follow these steps:
1. Identify the Components:
- Each rod has a mass M and length l.
- There are four rods forming a square frame.
2. Moment of Inertia of a Single Rod:
- The moment of inertia I of a thin rod about an axis through its center and perpendicular to its length is given by:
Icenter=112Ml2
3. Using the Perpendicular Axis Theorem:
- For a rod positioned horizontally, we want to find the moment of inertia about an axis through the center of the square and perpendicular to its plane.
- The distance from the center of the square to the center of the rod is l2.
- We can use the Parallel Axis Theorem to find the moment of inertia about this new axis:
I=Icenter+Md2
- Here, d=l2, so:
I=112Ml2+M(l2)2
- Simplifying this gives:
I=112Ml2+Ml24=112Ml2+312Ml2=412Ml2=13Ml2
4. Total Moment of Inertia for the Square Frame:
- Since there are four identical rods, the total moment of inertia Itotal is:
Itotal=4⋅13Ml2=43Ml2
Final Result:
The moment of inertia of the square frame about an axis through the center and perpendicular to its plane is:
Itotal=43Ml2
- Each rod has a mass M and length l.
- There are four rods forming a square frame.
- The moment of inertia I of a thin rod about an axis through its center and perpendicular to its length is given by:
Icenter=112Ml2
- For a rod positioned horizontally, we want to find the moment of inertia about an axis through the center of the square and perpendicular to its plane.
- The distance from the center of the square to the center of the rod is l2.
- We can use the Parallel Axis Theorem to find the moment of inertia about this new axis:
I=Icenter+Md2
- Here, d=l2, so:
I=112Ml2+M(l2)2
- Simplifying this gives:
I=112Ml2+Ml24=112Ml2+312Ml2=412Ml2=13Ml2
- Since there are four identical rods, the total moment of inertia Itotal is:
Itotal=4⋅13Ml2=43Ml2
The moment of inertia of the square frame about an axis through the center and perpendicular to its plane is:
Itotal=43Ml2
Topper's Solved these Questions
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 3 ( Section-B )
24 Videos
- ROTATIONAL MOTION BOOK - MOTIONCHAPTER - ROTATIONAL MOTION EXERCISE - Exercise - 2
40 Videos
- RAY OPTICSBOOK - MOTIONCHAPTER - RAY OPTICSEXERCISE - Exercise-4
38 Videos
- SEMI CONDUCTOR AND LOGIC GATESBOOK - MOTIONCHAPTER - SEMI CONDUCTOR AND LOGIC GATESEXERCISE - EXERCISE 3
34 Videos
Similar Questions
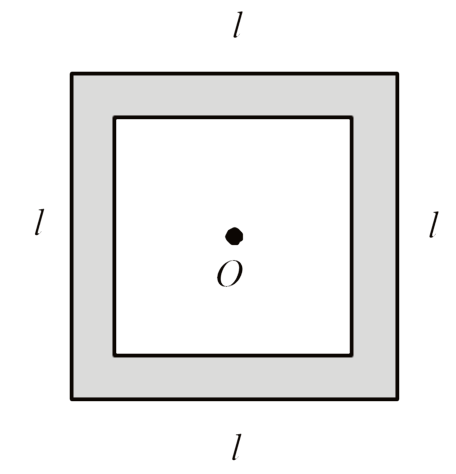
Four thin rods of same mass M and same length l, form a square as shown in figure. Moment of inertia of this system about an axis through centre O and perpendicular to its plane is
View Solutionfour thin rods each of mass m and length l are joined to make a square. Find moment of inertia of all the four rods about any side of the square.
View Solution
Knowledge Check
Four identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
A13Ml2B43Ml2C23Ml2D133Ml2Four thin rods of same mass M and same length l, form a square as shown in figure. Moment of inertia of this system about an axis through centre O and perpendicular to its plane is
 A43Ml2BMl23CMl26D23Ml2
A43Ml2BMl23CMl26D23Ml2Four thin rods same mass M and same length l, from a square as shown in figure. Moment of inertia of this system about an axis passing through one corner of the square and perpendicular to its plane is
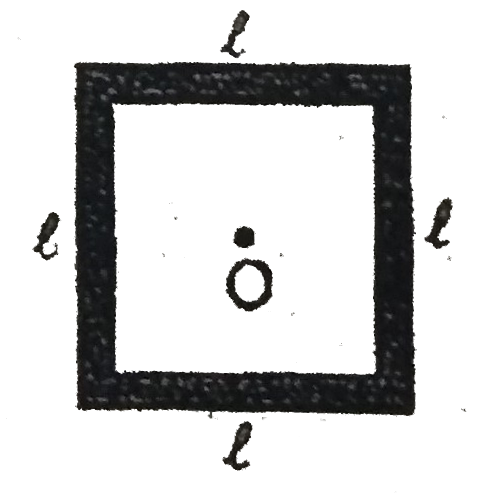 A103Ml2BMl23CMl26D23Ml2
A103Ml2BMl23CMl26D23Ml2
Three identical rods, each of mass m and length l, form an equaliteral triangle. Moment of inertia about one of the sides is

View SolutionFour thin rods of same mass m and same length L form a square as shown in the figure. M.I. of this system about an axis through center O and perpendicular to its plane is
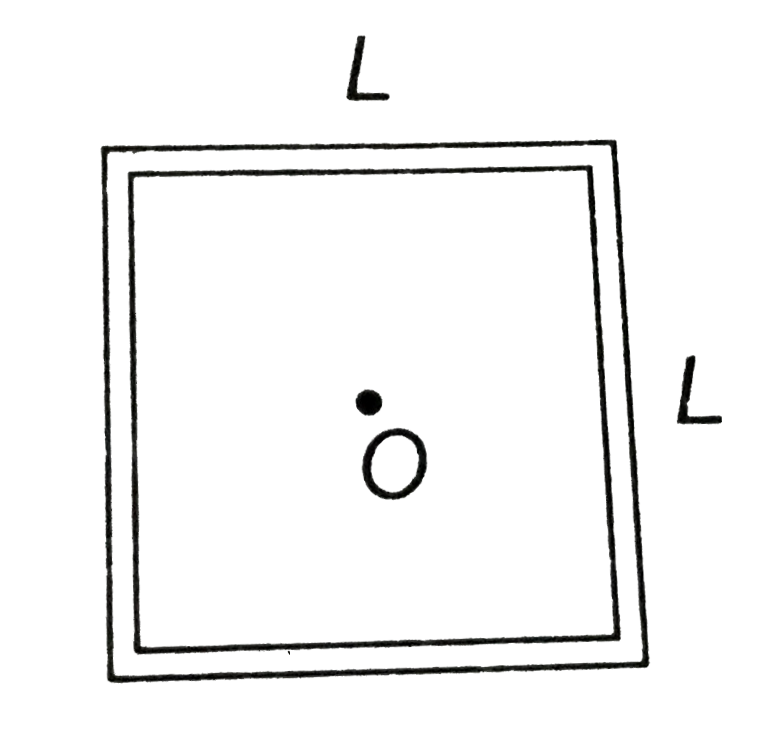
View SolutionTwo rods each of mass m and length l are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them is :
View SolutionTwo rods each of mass m and length l are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them is :
View SolutionTwo rods each of mass m and length 1 are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them, is :
View Solution
