four thin rods each of mass m and length l are joined to make a square. Find moment of inertia of all the four rods about any side of the square.
To find the moment of inertia of a square made of four thin rods about any one side of the square, we can follow these steps:
Step 1: Identify the Configuration
We have a square formed by four thin rods, each of mass m and length l. We need to calculate the moment of inertia about one of the sides of the square.
Step 2: Understand the Moment of Inertia
The moment of inertia I of a rod about an axis through one end and perpendicular to its length is given by the formula:
I=13ml2
For a rod along the axis, the moment of inertia is zero because the distance from the axis to the mass is zero.
Step 3: Calculate the Moment of Inertia for Each Rod
1. Rod along the axis (let's call it Rod 4):
- Since this rod is along the axis AA', its moment of inertia I4=0.
2. Rod perpendicular to the axis (Rod 1 and Rod 3):
- Both Rod 1 and Rod 3 are perpendicular to the axis AA'. Their moments of inertia can be calculated using the formula mentioned above:
I1=I3=13ml2
3. Rod parallel to the axis (Rod 2):
- For Rod 2, we will use the parallel axis theorem. The moment of inertia about the center of mass (which is along the length of the rod) is:
IBB′=0(since it is along the rod)
- The distance X from the center of mass of Rod 2 to the axis AA' is l (the length of the rod). Using the parallel axis theorem:
IAA′=IBB′+m⋅X2=0+m⋅l2=ml2
Step 4: Sum the Moments of Inertia
Now we can sum the moments of inertia of all four rods about the axis AA':
IAA′=I1+I2+I3+I4
Substituting the values we calculated:
IAA′=13ml2+ml2+13ml2+0
IAA′=13ml2+13ml2+ml2=23ml2+ml2=53ml2
Final Result
Thus, the moment of inertia of the square about any one side is:
IAA′=53ml2
We have a square formed by four thin rods, each of mass m and length l. We need to calculate the moment of inertia about one of the sides of the square.
The moment of inertia I of a rod about an axis through one end and perpendicular to its length is given by the formula:
I=13ml2
For a rod along the axis, the moment of inertia is zero because the distance from the axis to the mass is zero.
1. Rod along the axis (let's call it Rod 4):
- Since this rod is along the axis AA', its moment of inertia I4=0.
- Both Rod 1 and Rod 3 are perpendicular to the axis AA'. Their moments of inertia can be calculated using the formula mentioned above:
I1=I3=13ml2
- For Rod 2, we will use the parallel axis theorem. The moment of inertia about the center of mass (which is along the length of the rod) is:
IBB′=0(since it is along the rod)
- The distance X from the center of mass of Rod 2 to the axis AA' is l (the length of the rod). Using the parallel axis theorem:
IAA′=IBB′+m⋅X2=0+m⋅l2=ml2
Now we can sum the moments of inertia of all four rods about the axis AA':
IAA′=I1+I2+I3+I4
Substituting the values we calculated:
IAA′=13ml2+ml2+13ml2+0
IAA′=13ml2+13ml2+ml2=23ml2+ml2=53ml2
Thus, the moment of inertia of the square about any one side is:
IAA′=53ml2
Topper's Solved these Questions
- ROTATIONAL MECHANICSBook:DC PANDEYChapter:ROTATIONAL MECHANICSExercise:Exercise 12.2
Explore 4 Videos
- ROTATIONAL MECHANICSBook:DC PANDEYChapter:ROTATIONAL MECHANICSExercise:Exercise 12.3
Explore 4 Videos
- ROTATIONAL MECHANICSBook:DC PANDEYChapter:ROTATIONAL MECHANICSExercise:Solved Example
Explore 1 Video
- ROTATIONBook:DC PANDEYChapter:ROTATIONExercise:(C) Chapter Exercises
Explore 39 Videos
- ROTATIONAL MOTIONBook:DC PANDEYChapter:ROTATIONAL MOTIONExercise:Integer Type Questions
Explore 17 Videos
Similar Questions
Three identical thin rods, each of mass m and length l, are joined to form an equilateral triangular frame. Find the moment of inertia of the frame about an axis parallel to tis one side and passing through the opposite vertex. Also find its radius of gyration about the given axis.
View SolutionAB and CD are two identical rods each of length l and mass m joined to form a cross. The moment of inertia of these two rods about a bisector (xy) of the angle between the rods is:

View Solution
Knowledge Check
Four identical thin rods each of mass M and length l, from a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is
A13Ml2B43Ml2C23Ml2D133Ml2Four identical thin rods each of mass M and length l3, form a square frame. Moment of inertia of this frame about an axis through the centre of the square and perpendicular to its plane is---
A13ml2B43Ml2C23Ml2D133Ml2Two rods each of mass m and length l are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them is :
Aml2/12Bml2/6Cml2/3Dml2/2
Two identical rods each of mass M and length L are kept according to figure. Find the moment of inertia of rods about an axis passing through O and perpendicular to the plane of rods.

View SolutionTwo rods each of mass m and length l are joined at the centre to form a cross. The moment of inertia of this cross about an axis passing through the common centre of the rods and perpendicular to the plane formed by them is :
View SolutionTwo uniform, thin identical rods each of mass M and length l are joined together to form a cross. What will be the moment of inertia of the cross about an axis passing through the point at which the two rods are joined and perpendicular to the plane of the cross ?
View SolutionTwo uniform thin identical rods AB and CD each of mass M and length L are joined so as to form a cross as shown. The moment of inertia of the cross about a bisector line EF is :-
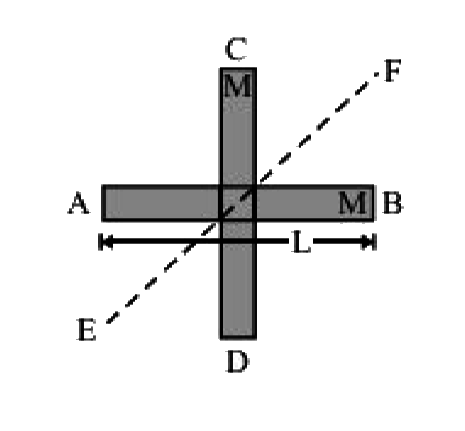
View SolutionFour thin uniform rods each of length L and mass m are joined to form a square . The moment of inertia of square about an axis along its one diagonal is
View Solution