A cylinder is rolling without sliding over two horizontal planks (surfaces) 1 and 2. If the velocities of the surfaces A and B are −vˆi and 2vˆi respectively, find the:
a. Position of instantaneous axis of rotation.
b. Angular velocity of the cylinder.
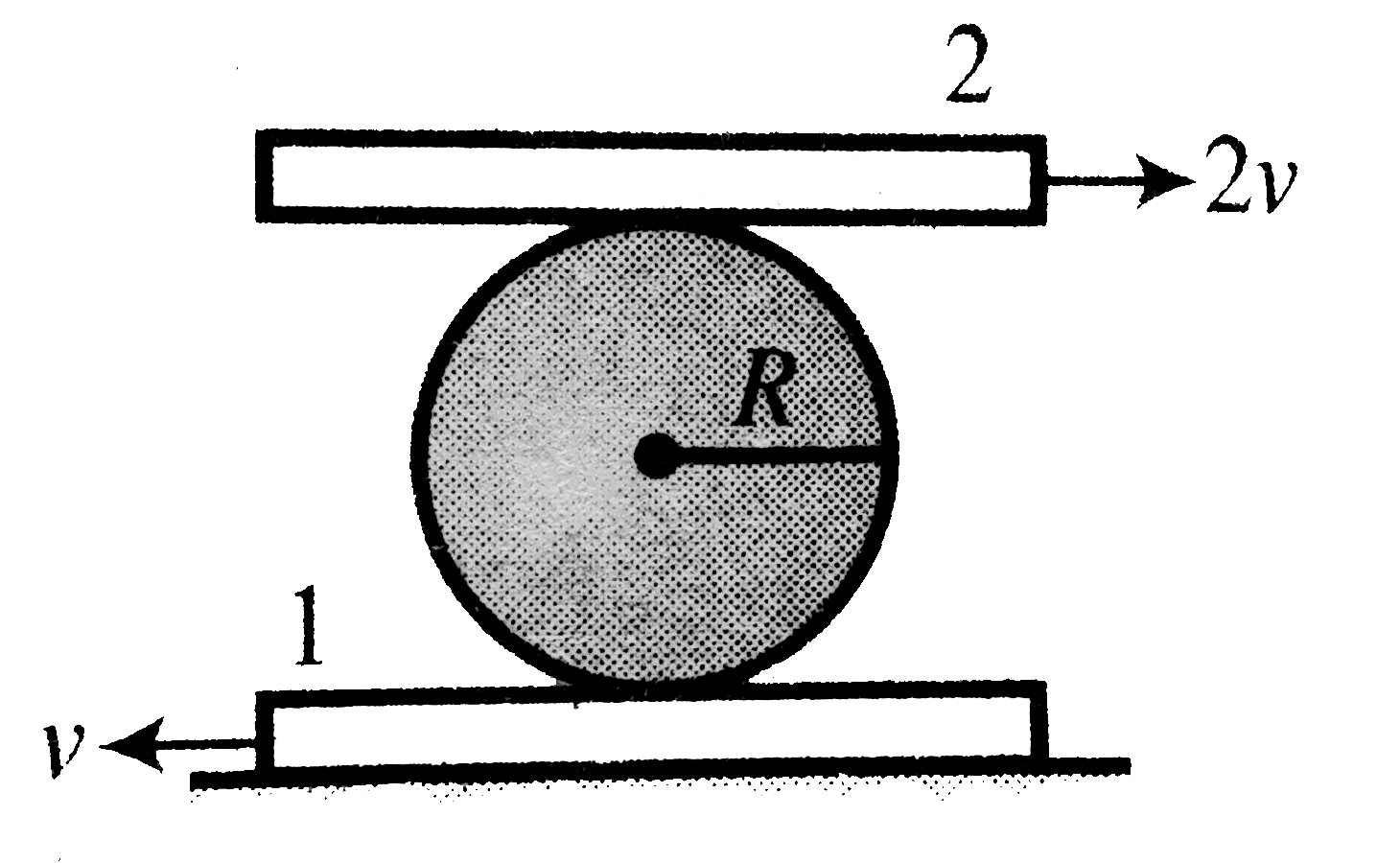
The correct Answer is:3v/2R
a. Since there is no relative sliding between the cylinder and the planks 1 and 2, the points, A and B of the disc will move with velocities equal to the velocities of the respective surface.
→vA=−vˆi and →vB=−2vˆi
respectively. Joining A and B and A' and B' we find the point P as IAR. Then we have the similar triangles PAA' and PBB'. Using the properties of similar triangles we have

ω=BB'AA'=BPAP
AA'=vA=v,BB'=vB=2v,AP=2R−x and BP=x.
Then we have 2vv=x2R−x
This gives, x=3R3
hence IAR is located at a distance of 4R/3 below the top of the disc.
b. As we know ω=vABAB
vAB=(→vA−→vB|=|−→vˆi−2vˆi∣=3v and AB=2R
This gives ω=3v2R clockwise
Topper's Solved these Questions
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Single Correct
97 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Multiple Correct
9 Videos
- RIGID BODY DYNAMICS 1BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 1EXERCISE - Exercise 2.4
11 Videos
- PROPERTIES OF SOLIDS AND FLUIDSBOOK - CENGAGE PHYSICSCHAPTER - PROPERTIES OF SOLIDS AND FLUIDSEXERCISE - INTEGER_TYPE
2 Videos
- RIGID BODY DYNAMICS 2BOOK - CENGAGE PHYSICSCHAPTER - RIGID BODY DYNAMICS 2EXERCISE - Interger
2 Videos
Similar Questions
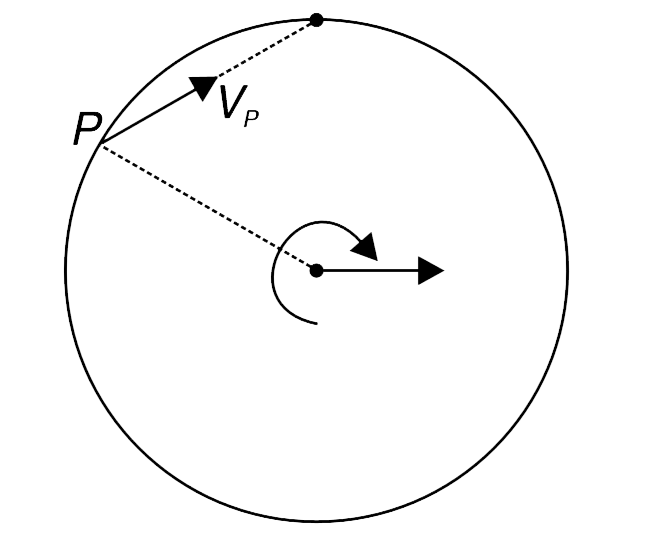
A wheel is rolling without sliding on a horizontal surface. Prove that velocities of all points on the circumference of the wheel are directed towards the top most point of the wheel.
View SolutionA disc rolls without slipping on a horizontal surface such that its velocity of center of the mass is v. Find the velocity of points A,B,C and D.
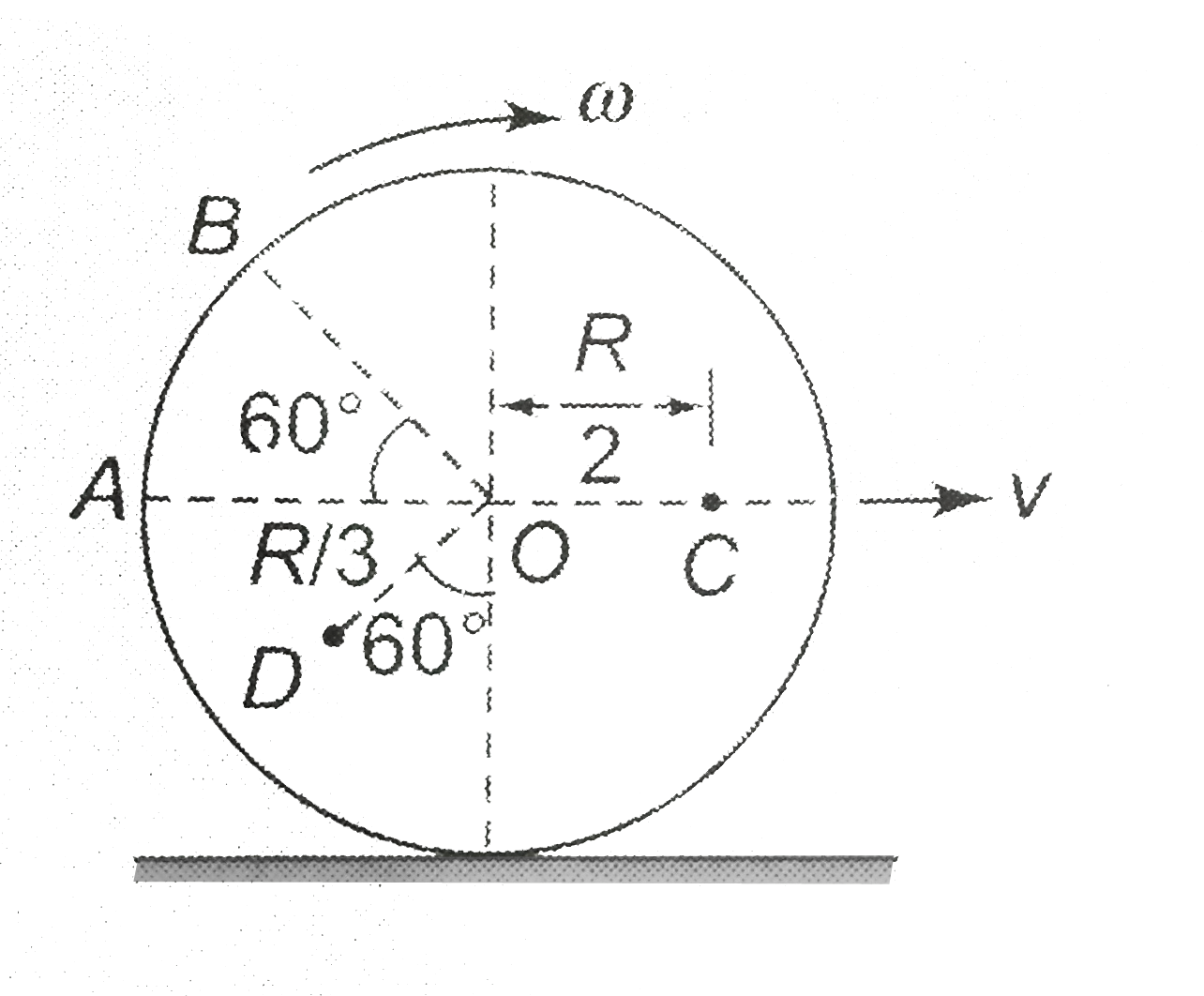
View Solution
Knowledge Check
A disc is rolling without sliding on a horizontal surface. Velocity of the centre of the disc is V. Then the maximum relative sped of any point on the circumference of the disc with respect to point P is.
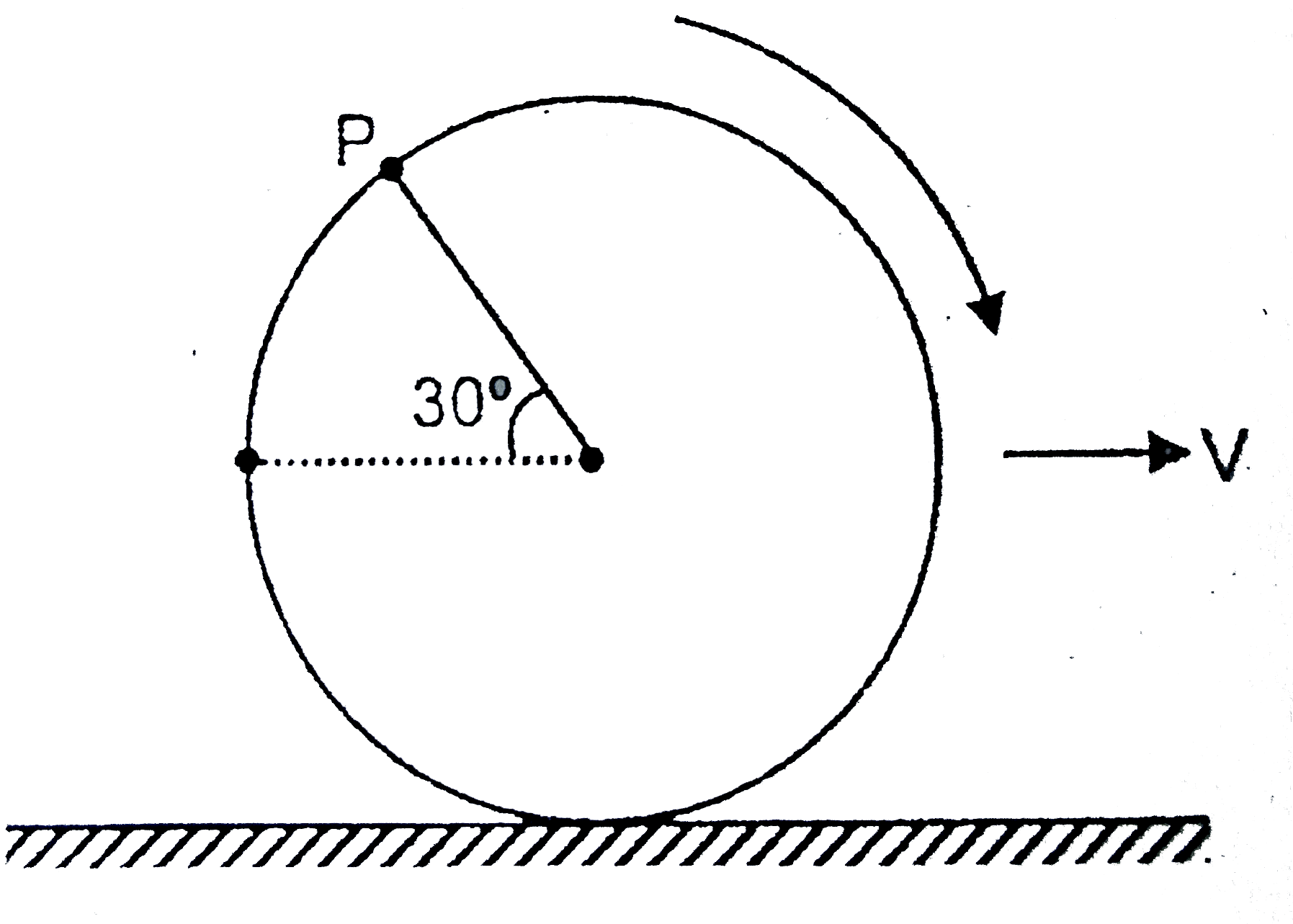 AVB2VC3VD2V3
AVB2VC3VD2V3
A cylinder A rolls without slipping on a plank B.The velocities of center of the cylinder and that of the plank are 4m/s and 2m/s respectively in same direction,with respect to the ground.Find the angular velocity ( in rad /sec) of the cylinder if its radius is 1m .
View SolutionA cylinder rolls without slipping over a horizontal plane. The radius of the cylinder is equal to r. Find the curvature radii of trajectories traced out by the points A and B(see figure)

View SolutionIn figure (a) there is a uniform cylinder of mass M and radius R. Length of the cylinder is L=√3R. The cylinder is rolling without sliding on a horizontal surface with its centre moving at speed V. In figure (b) the same cylinder is moving on a horizontal surface with its centre moving at speed V and the cylinder rotating about a vertical axis passing through its centre. [Place your pencil on the table and give a sharp blow at its end. Look at the motion of the pencil. This is how the cylinder is moving]. The angular speed is ω=VR. Write the kinetic energy of the cylinder in two cases. In which case, the kinetic energy would have been higher if length of the cylinder were doubled (=2√3R).
(a)
View SolutionA uniform disc of radius R rolls perfectly over two horizontal plank A and B moving with velocities v and 2v, respectively. Find the
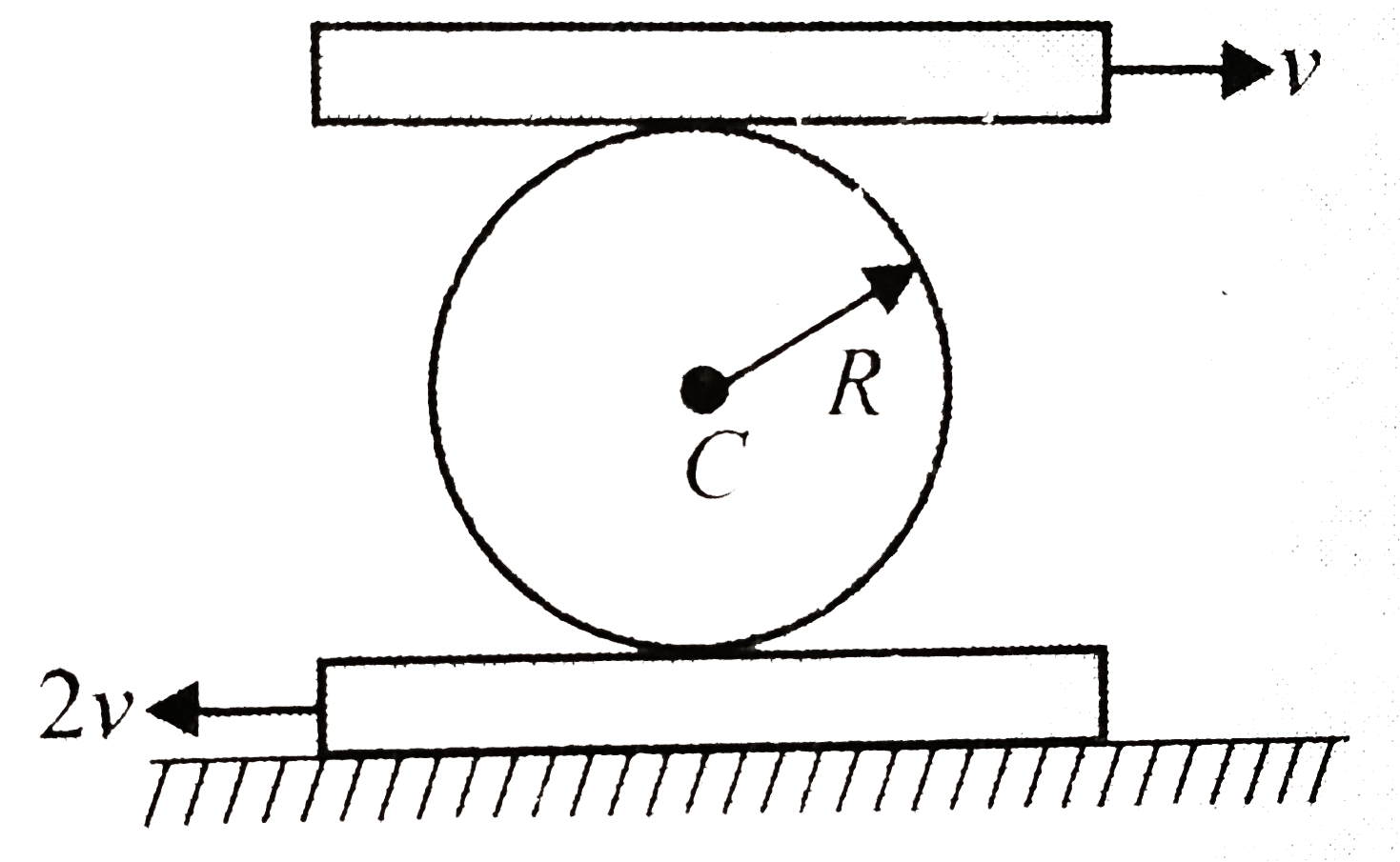
a. velocity of CM of the disc.
b. angular velocity of the disc.
View SolutionA bobbin rolls without slipping over a horizontal surface so that the velocity v of the end of the thread (point A) is directed along the horizontal . A board hinged at point B leans against the bobbin (Fig.) The inner and outer radii of the bobbin are r and R respectively. Determine the angular velocity ω of teh board as a function of an angle α.

View Solution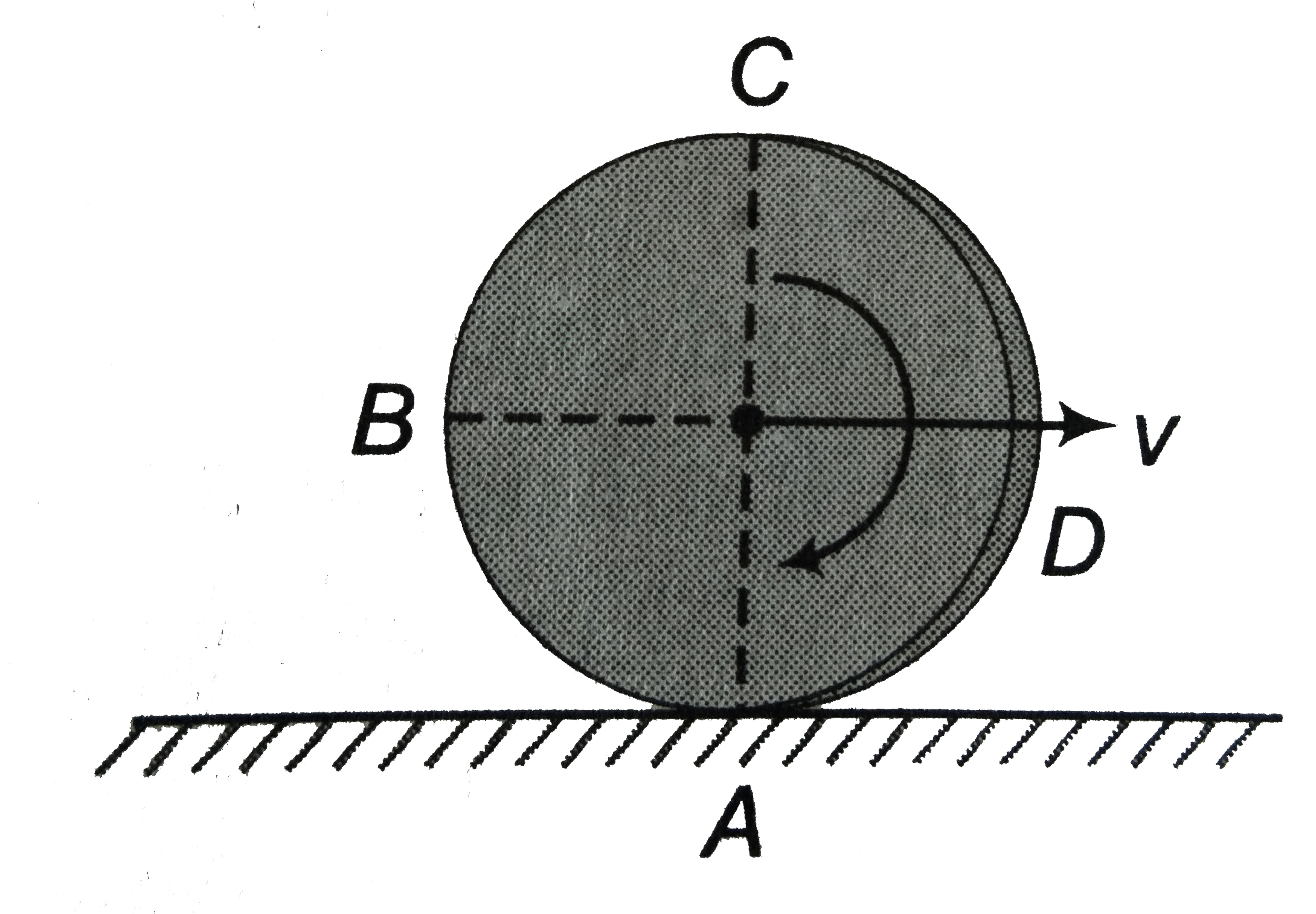
A disc is rolling without slipping with linear velocity v as shown in figure. With the concept of instantaneous axis of rotation, find velocities of point A, B, C and D.
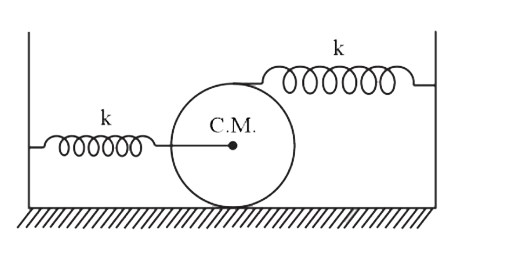
View SolutionA uniform solid cylinder can roll without sliding on a horizontal surface as shown in the figure. The mass of cylinder is 10 kg and the spring constant for both the ideal springs is 300Nm−1 . What is the angular frequency (in rad s−1 ) of small oscillations of the centre of the cylinder?
View Solution
