A uniform solid cylinder can roll without sliding on a horizontal surface as shown in the figure. The mass of cylinder is 10 kg and the spring constant for both the ideal springs is 300Nm−1 . What is the angular frequency (in rad s−1 ) of small oscillations of the centre of the cylinder?
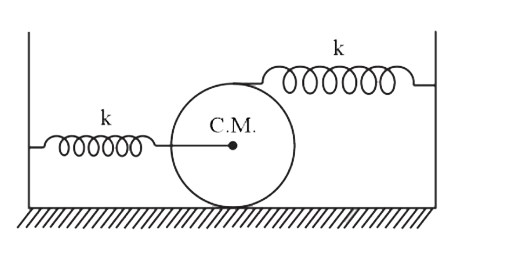
The correct Answer is:10
Step by step video, text & image solution for A uniform solid cylinder can roll without sliding on a horizontal surface as shown in the figure. The mass of cylinder is 10 kg and the spring constant for both the ideal springs is 300 Nm^(-1) . What is the angular frequency (in rad s^(-1) ) of small oscillations of the centre of the cylinder? by Physics experts to help you in doubts & scoring excellent marks in Class 12 exams.
Updated on:21/07/2023Topper's Solved these Questions
Similar Questions
Knowledge Check
A solid cylinder of mass M and radius R pure rolls on a rough surface as shown in the figure. Choose the correct alternative (s).
 AThe acceleration of the centre of mass is FMBThe acceleration of the centre of mass is 23FMCThe friction force on the cylinder acts backwardDThe magnitude of the friction force is F3
AThe acceleration of the centre of mass is FMBThe acceleration of the centre of mass is 23FMCThe friction force on the cylinder acts backwardDThe magnitude of the friction force is F3A solid cylinder is attached to a horizonatal massless spring as shwn in figure.If the cyclinder rolls without slipping, the time period of oscillation of the cyclinder is
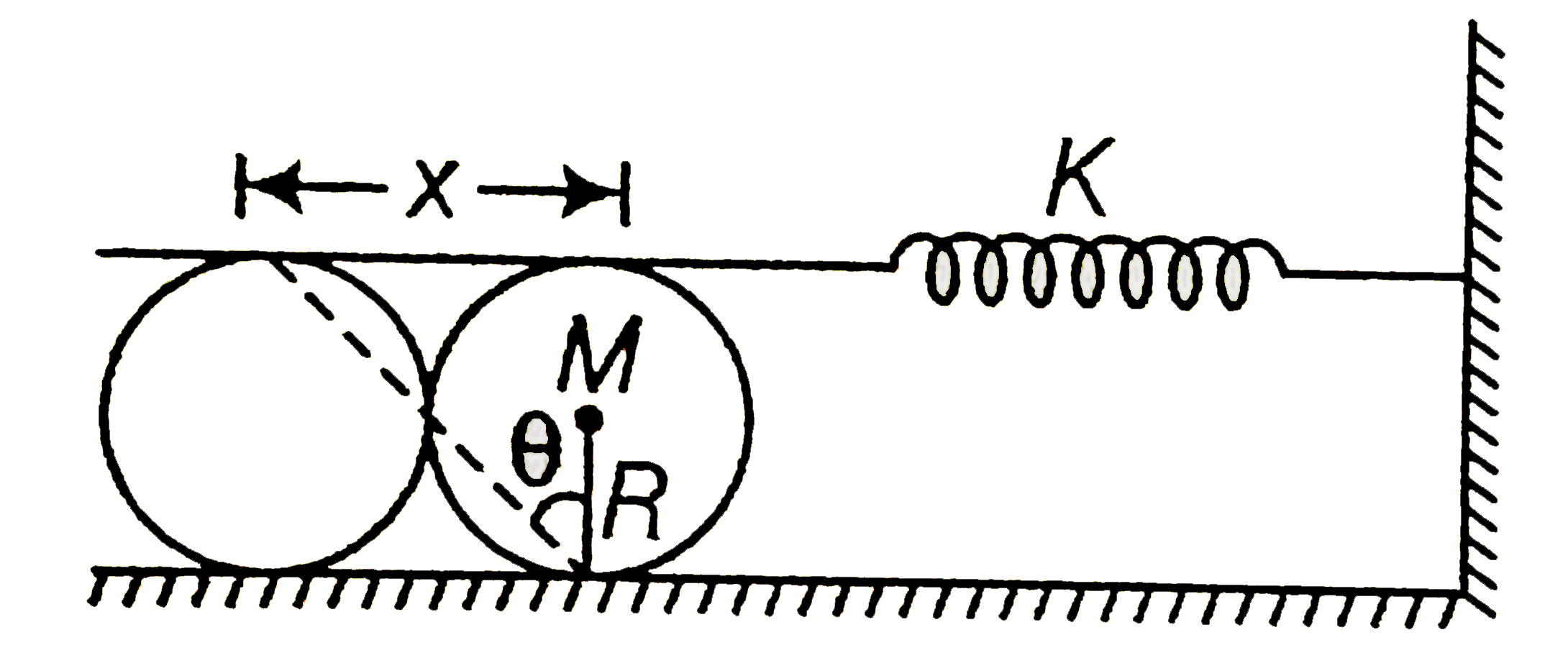 A2π√xgB2π√2M3KC2π√3M8KD2π√3M2K
A2π√xgB2π√2M3KC2π√3M8KD2π√3M2KA mass M=5 kg is attached to a string as shown in the figure and held in position so that the spring remains unstretched. The spring constant is 200Nm−1 . The mass M is then released and begins to undergo small oscillations. The amplitude of oscillation is
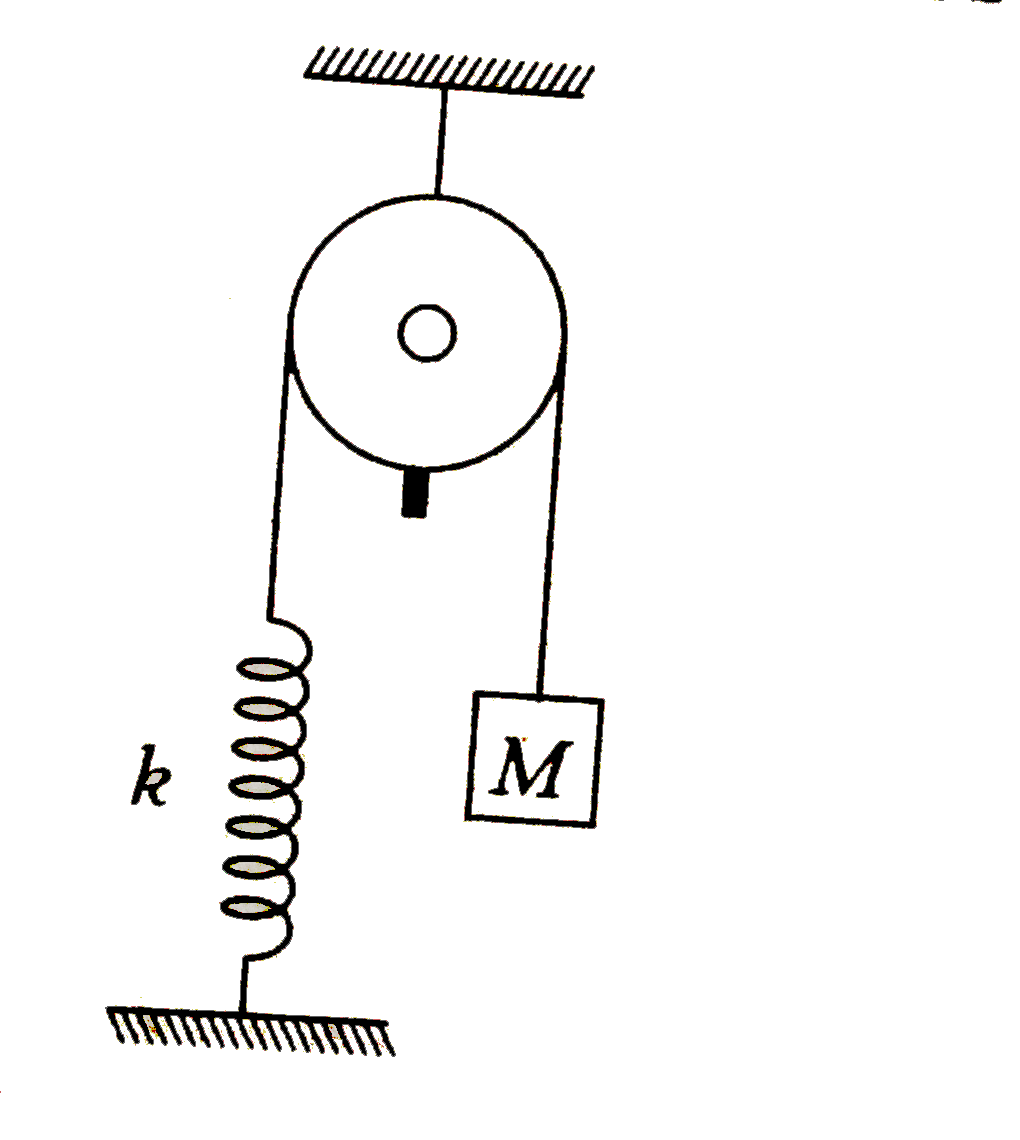 A0.5 mB0.25 mC0.2 mD0.1 m
A0.5 mB0.25 mC0.2 mD0.1 m
A cylinder rolls on a horizontal polane surface. If the speed of the centre is 25 m/s, what is the speed of the highest point?
View SolutionA solid cylinder of mass 3kg is rolling on a horizontal surface with velocity 4ms−1. It collides with a horizontal spring of force constant 200Nm−1. The maximum compression producec in the spring will be :
View SolutionA solid cylinder of mass 3 kg is rolling on a horizontal surface with velocity 4ms−1. It collides with a horizontal spring of force constant 200Nm−1. The maximum compression produced in the spring will be
View SolutionA hoop and a solid cylinder have the same mass and radius. They both roll,without slipping, on horizontal surface. If their kinetic energies are equal -
View SolutionA uniform solid cylindrical roller of mass 'm' is being pulled on a horizontal surface with force F parallel to the surface and applied at its centre. If the acceleration of the cylinder is 'a' and it is rolling without slipping, then the value of 'F' is :−
View Solution

