The potential energy of a particle is determined by the expression U=α(x2+y2), where α is a positive constant. The particle begins to move from a point with coordinates (3,3), only under the action of potential field force. Then its kinetic energy T at the instant when the particle is at a point with the coordinates (1,1) is
(a) 8α
(b) 24α
(c) 16α
(d) Zero
The correct Answer is:C
To solve the problem, we will use the principle of conservation of mechanical energy. The total mechanical energy of the system remains constant when only conservative forces are acting on the particle.
1. Identify the Potential Energy Function:
The potential energy U of the particle is given by:
U=α(x2+y2)
where α is a positive constant.
2. Calculate Initial Potential Energy:
The particle starts from the point (3,3). We can calculate the initial potential energy Ui:
Ui=α(32+32)=α(9+9)=18α
3. Calculate Final Potential Energy:
The particle moves to the point (1,1). We will calculate the final potential energy Uf:
Uf=α(12+12)=α(1+1)=2α
4. Apply Conservation of Mechanical Energy:
According to the conservation of mechanical energy:
ΔT+ΔU=0
This implies:
ΔT=−ΔU
where ΔU=Uf−Ui.
5. Calculate Change in Potential Energy:
Now we can find ΔU:
ΔU=Uf−Ui=2α−18α=−16α
6. Calculate Change in Kinetic Energy:
Substituting ΔU into the equation for ΔT:
ΔT=−(−16α)=16α
7. Conclusion:
The kinetic energy T at the point (1,1) is:
T=16α
Final Answer:
The kinetic energy T at the instant when the particle is at the point (1,1) is 16α.
---
The potential energy U of the particle is given by:
U=α(x2+y2)
where α is a positive constant.
The particle starts from the point (3,3). We can calculate the initial potential energy Ui:
Ui=α(32+32)=α(9+9)=18α
The particle moves to the point (1,1). We will calculate the final potential energy Uf:
Uf=α(12+12)=α(1+1)=2α
According to the conservation of mechanical energy:
ΔT+ΔU=0
This implies:
ΔT=−ΔU
where ΔU=Uf−Ui.
Now we can find ΔU:
ΔU=Uf−Ui=2α−18α=−16α
Substituting ΔU into the equation for ΔT:
ΔT=−(−16α)=16α
The kinetic energy T at the point (1,1) is:
T=16α
The kinetic energy T at the instant when the particle is at the point (1,1) is 16α.
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Multiple Correct
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Linked Comprehension
55 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Subjective
23 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
The potential energy of a particle is determined by the expression U=α(x2+y2), where α is a positive constant. The particle begins to move from a point with the co-ordinates (3, 3) only under the action of potential fields force. When it reaches the point (1, 1) its kinetic energy is 4Kα. Find the value of K.
View SolutionThe potential energy of a particle under a conservative force is given by U(x)=(x2−3x) J. The equilibrium position of the particle is at
View Solution
Knowledge Check
The potential energy function of a particle in the x-y plane is given by U=k(x+y), where (k) is a constant. The work done by the conservative force in moving a particlae from (1,1) to (2,3) is .
A−3kB+3kCkDNone of theseThe potential energy of a point particle is given by the expression V(x)=−αx+βsin(x/γ). A dimensionless combination of the constant α,β and γ is–
Aα/βγBα2/βγCγ/αβDαγ/βThe potential energy of a particle in a force field is:
U=Ar2−Br,. Where A and B are positive
constants and r is the distance of particle from the centre of the field. For stable equilibrium the distance of the particle isAabBbaC2abD2ba
The potential energy of a particle in a conservative field is U=ar3−br2 where a and b are positive constants and r is the distance of particle from the centre of field. For equilibrium, the value of r is
View SolutionThe potential energy of a particle under a conservative force is given by U(x)=(x2−3x)J. The equilibrium position of the particle is at x m. The value of 10 x will be
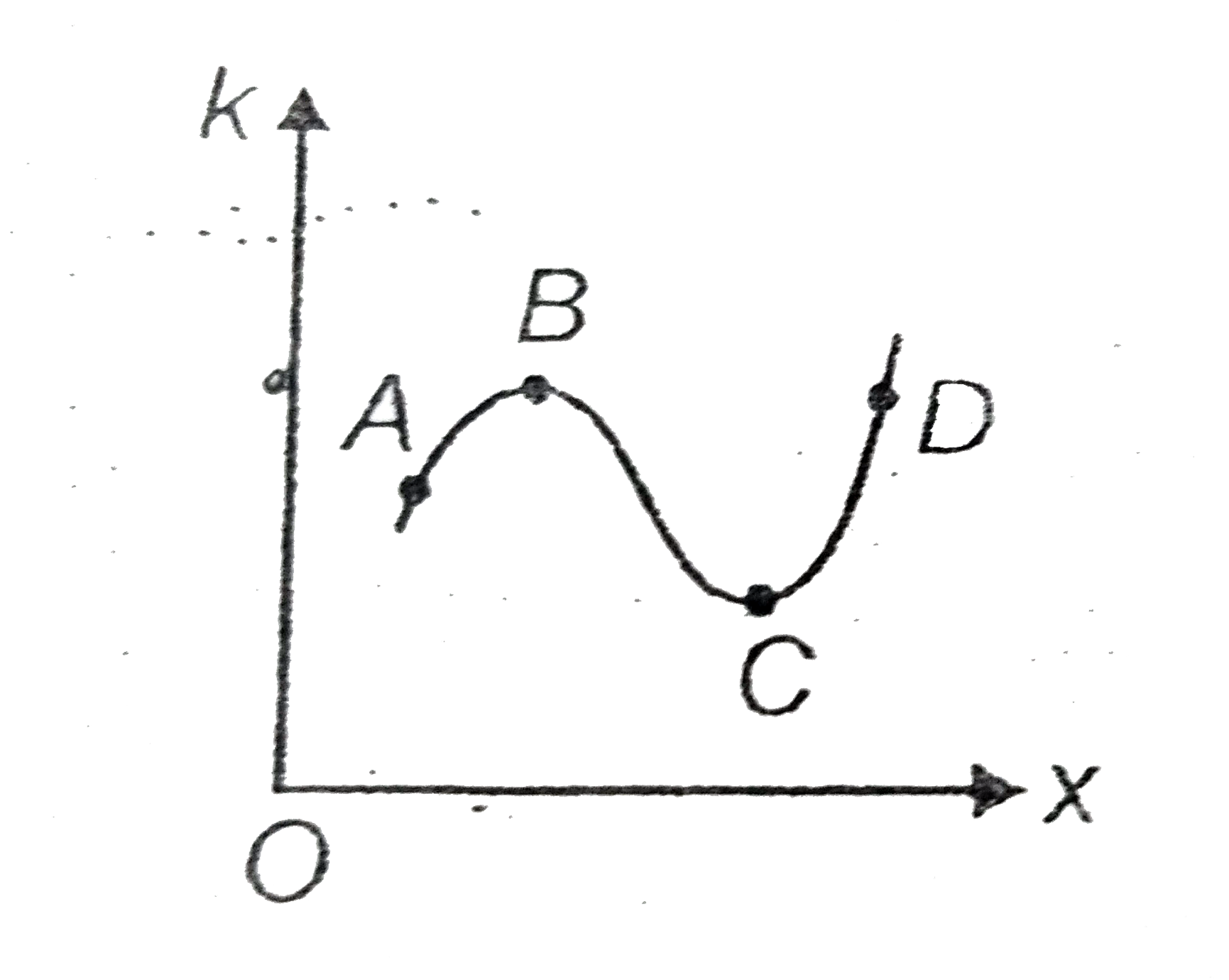
View SolutionFor a particle moving under the action of a variable force, kinetic energy (k) varsus position (x) graph is given, then
View SolutionThe potential energy of a particle in a certain field has the form U=a/r2−b/r, where a and b are positive constants, r is the distance from the centre of the field. Find:
(a) the value of r0 corresponding to the equilibrium position of the particle, examine where this position is steady,
(b) the maximum magnitude of the attraction force, draw the plots U(r) and Fr(r) (the projections of the force on the radius vector r).
View SolutionPotential energy of a particle at position x is given by U=x2−5x. Which of the following is equilibrium position of the particle?
View Solution
