In the above question, the average power delivered by gravity is

In the above question, the maximum power delivered by the agent in pulling up the rope is
A $λ / g v$
B(b) $λ / g v + \frac{v^{3} λ}{2}$
C(c) $λ / g v + v^{3} λ$
D(d) $\frac{l l g v}{2} + \frac{λ v^{3}}{2}$
In the given circuit the average power developed is

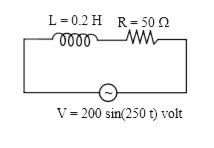A $50 \sqrt{2}$ watt
B200 watt
C $150 \sqrt{2}$ watt
D $200 \sqrt{2}$ watt
In the given circuit the average power developed is:

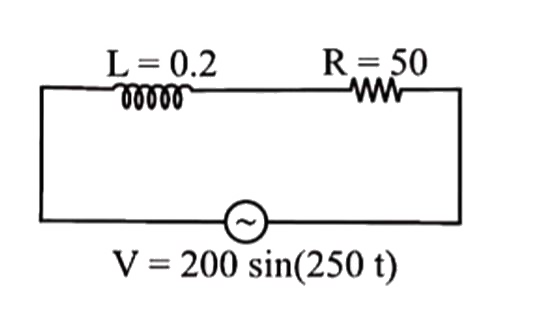A $50 \sqrt{2}$ watt
B200 watt
C $150 \sqrt{2}$ watt
D $200 \sqrt{2}$ watt

The correct Answer is:D