A uniform chain of mass m and length l is at the verge of sliding under the effect of gravity of the hanging part. Find the
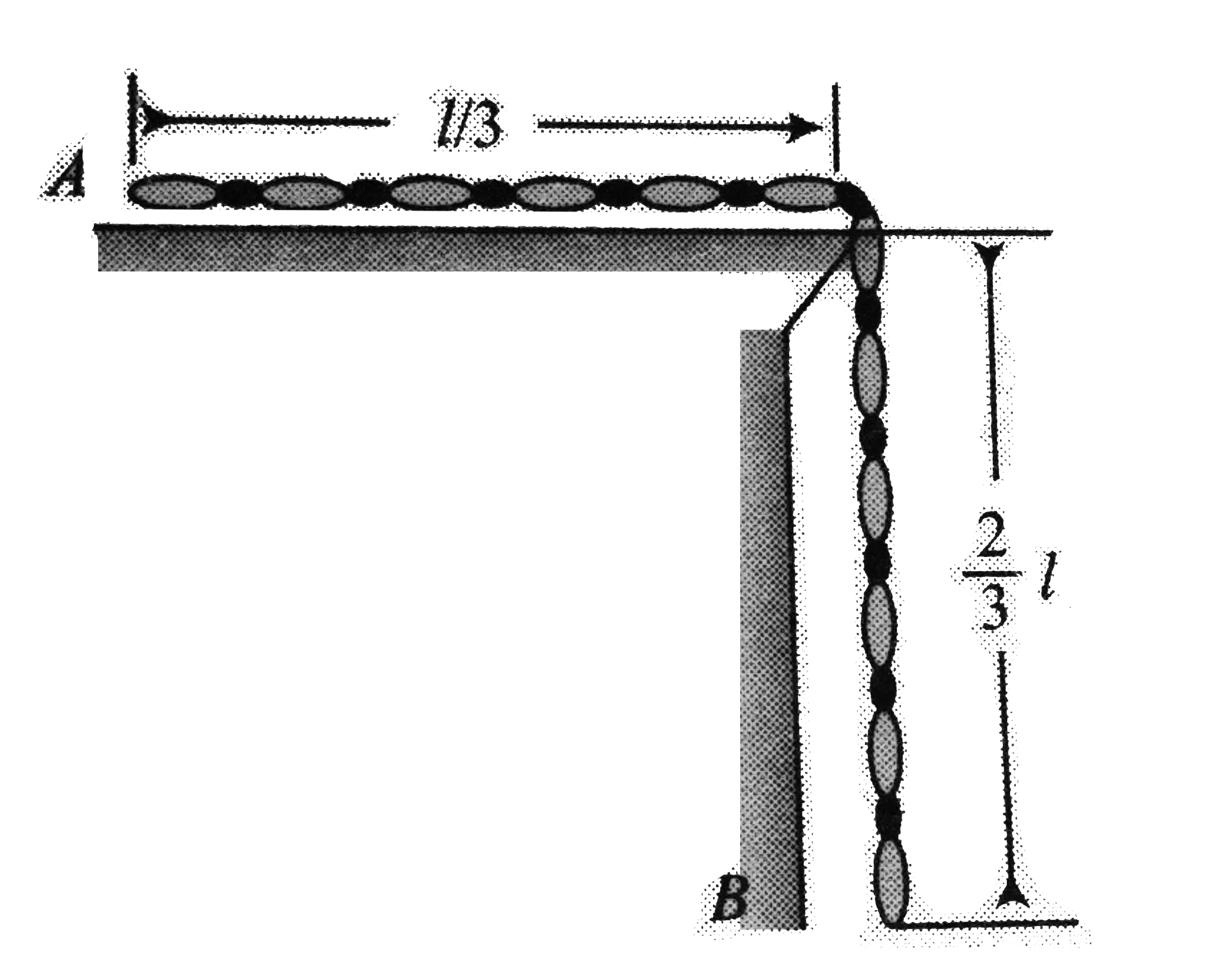
a. coefficient of friction, between chain and table.
b. work done by friction and gravity till the chain leaves the table if the hanging part is pulled gently and released.
c. speed of the chain at the time of leaving the table in part (b) .
d. work done by the external force acting at the end A of the chain in slowly pulling the chain completely onto the table.
a. Limiting friction acting on the upper part of chain will balance the weight of overhanging part.
μ(m3)g=2m3g⇒μ=2
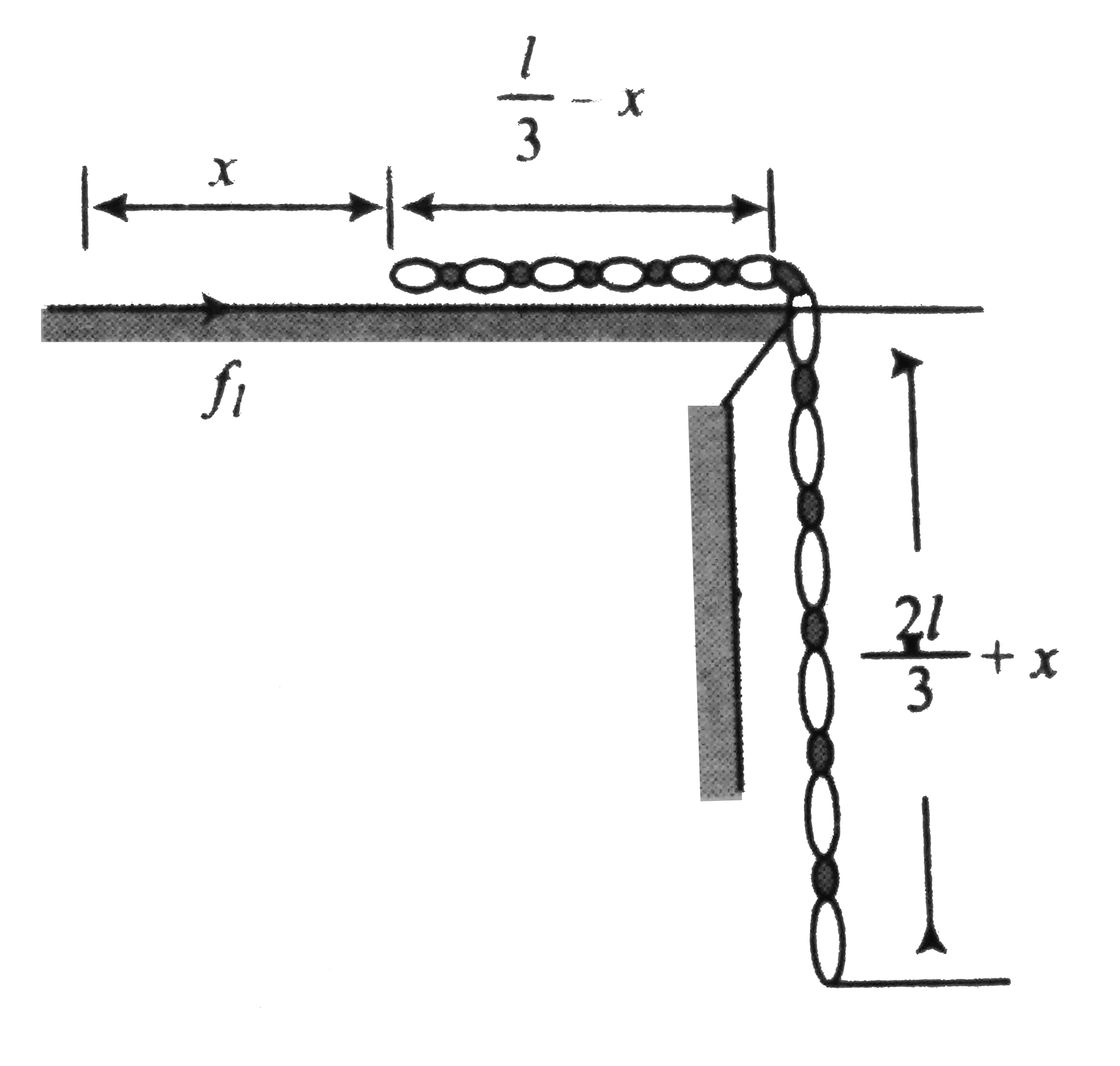
b. Let at any time, chain slides down by a distance x. Then
fl=μ[(l2−x)ml]g
Wf=−∫fldx=−μmgll/3∫0(l3−x)dx
⇒Wf=−μmgl18−2mgl18=−mgl9
⇒Wgravity=2m3gl3+m3gl6=5mgl18
c. Wgravity+Wf=12mv2
⇒5mgl18−mgl9=12mv2⇒v=√gl3
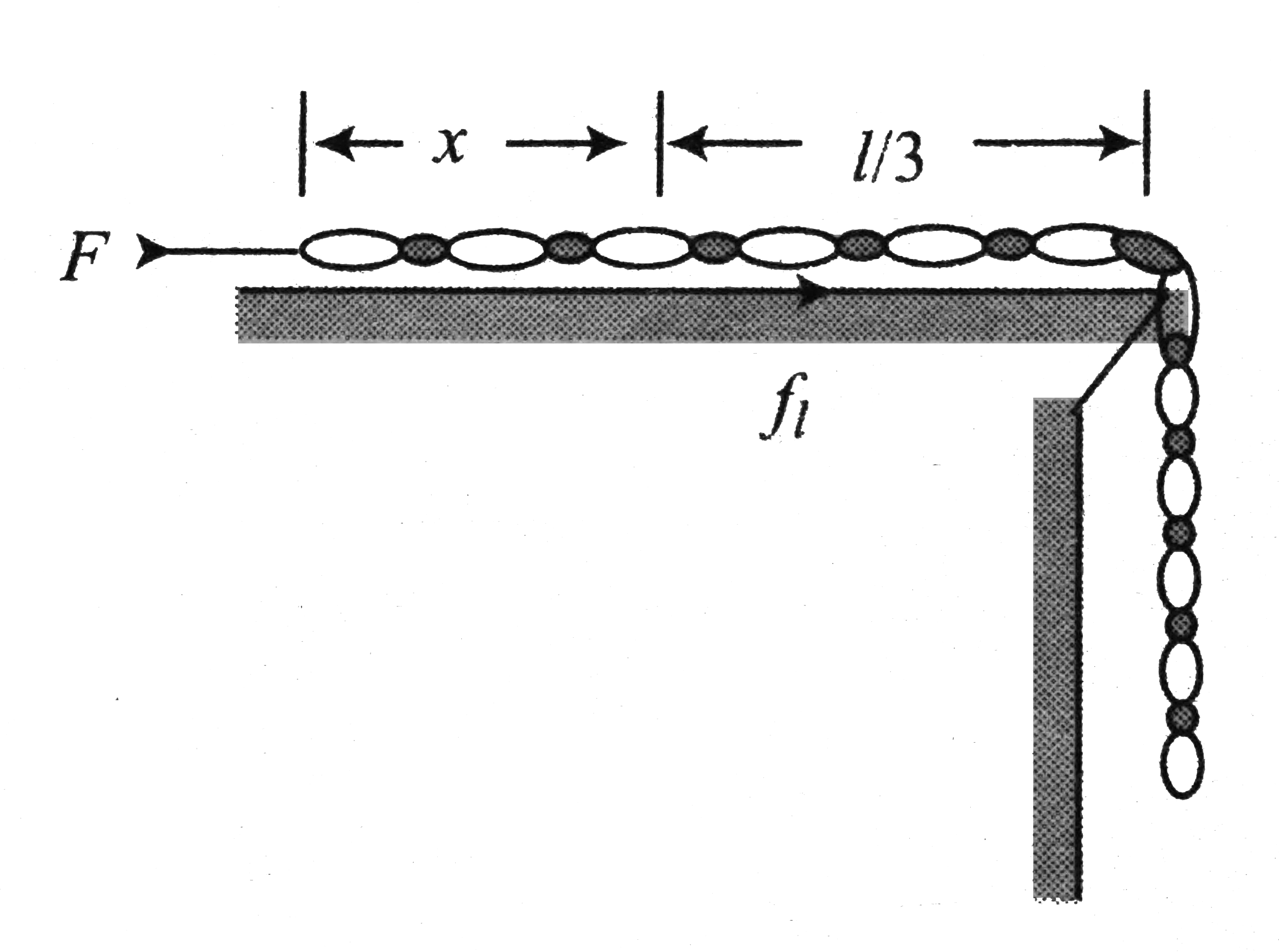
d. fl=μ(13+x)mlg
Wf=−∫fldx
=−μmgl2l/3∫0(l3+x)dx
=−4μmgl9=−8mgl9
Wgravity=−2m3gl3=−2mgl9
Wext+Wf+Wgravity=0
⇒Wext=8mgl9+2mgl9=10mgl9
Topper's Solved these Questions
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.1
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Exercise 8.2
25 Videos
- WORK, POWER & ENERGYBOOK - CENGAGE PHYSICSCHAPTER - WORK, POWER & ENERGYEXERCISE - Archives (integer)
4 Videos
- VECTORSBOOK - CENGAGE PHYSICSCHAPTER - VECTORSEXERCISE - Exercise Multiple Correct
5 Videos
Similar Questions
AS uniform chain of length L and mass M overhangs a horizontal table with its two third part n the table. The friction coefficient between the tabe and the chian is μ. Find the work done by the friction during the period the chain slips off the table.
View SolutionA uniform chain of length L and mass M overhangs a horizontal table with its two third part on the table. The friction coefficient between the table and the chain is µ. The work done by the friction during the period the chain slips off the table is [−2kμMgL]. Find the value of k.
View Solution
Knowledge Check
A uniform rope of total length l is at rest on a table with fraction f of its length hanging (see figure). If the coefficient of friction between the table and the chain is μ then
 Af=μBf=1/(1+μ)Cf=1/(1+1/μ)Df=1/(μ+1/μ)
Af=μBf=1/(1+μ)Cf=1/(1+1/μ)Df=1/(μ+1/μ)A chain is placed on a rough table, partially hanging, as shown in the figure. The coefficient of static friction between the chain and the table is μ = 0.4. If the maximum possible length of the hanging part is x and the length of the chain is L, then what is the value of Lx?
 AμL1+μBμL1−μC(1−μ)L1+μDμL
AμL1+μBμL1−μC(1−μ)L1+μDμLA uniform chain of mass m and length I is kept on the table with a part of it overhanging (see figure). If the coefficient of friction between the table and the chain is 1/3 then find the maximum length of the chain that can overhang such that the chain remain in equilibrium.
 AL/3BL/2CL/4D2L/3
AL/3BL/2CL/4D2L/3
A uniform chain of mass m and length l is lying on a horizontal table with one-third of its length hanging over the edge of the table.

If the chain is limiting equlibrium, what is the coefficient of friction for the contact between table and chain?
View SolutionA uniform chain of length L is lying partly on a table, the remaining part hanging down from the edge of the table. If the coefficient of friction between the chain and the table is 0.5 what is the minimum length of the chain that should lie on the table, to prevent the chain from slipping down to the ground.
View SolutionA chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
View SolutionA chain of mass m and length l is placed on a table with one-sixth of it hanging freely from the table edge. The amount of work. done to pull the chain on the table is
View SolutionA homogoneous chain of length L lies on a table. The coefficient of friction between the chain and the table is μ. The maximum length which can hang over the table in equilibrium is
View Solution