A particle moving with uniform acceleration along a straight line ABC rosses point A at t=0 with a velocity 12ms−1. is 40m away from A andC is 64m away from A. The particle passes B at t=4 s.<br>a.Afterwˆtimewillthepartic≤beatC?<br>b.WˆisitsvelocityatC?<br>c.Wˆdoesthepartic≤reachAaga∈?<br>d.Locatethep∮wherethepartic≤reversesitsdirectionofmotion.<br>e.F∈dthedistancecoveredbythepartic≤∈thefirst15 s.
Let the acceleratuib of the oartucke be (a)
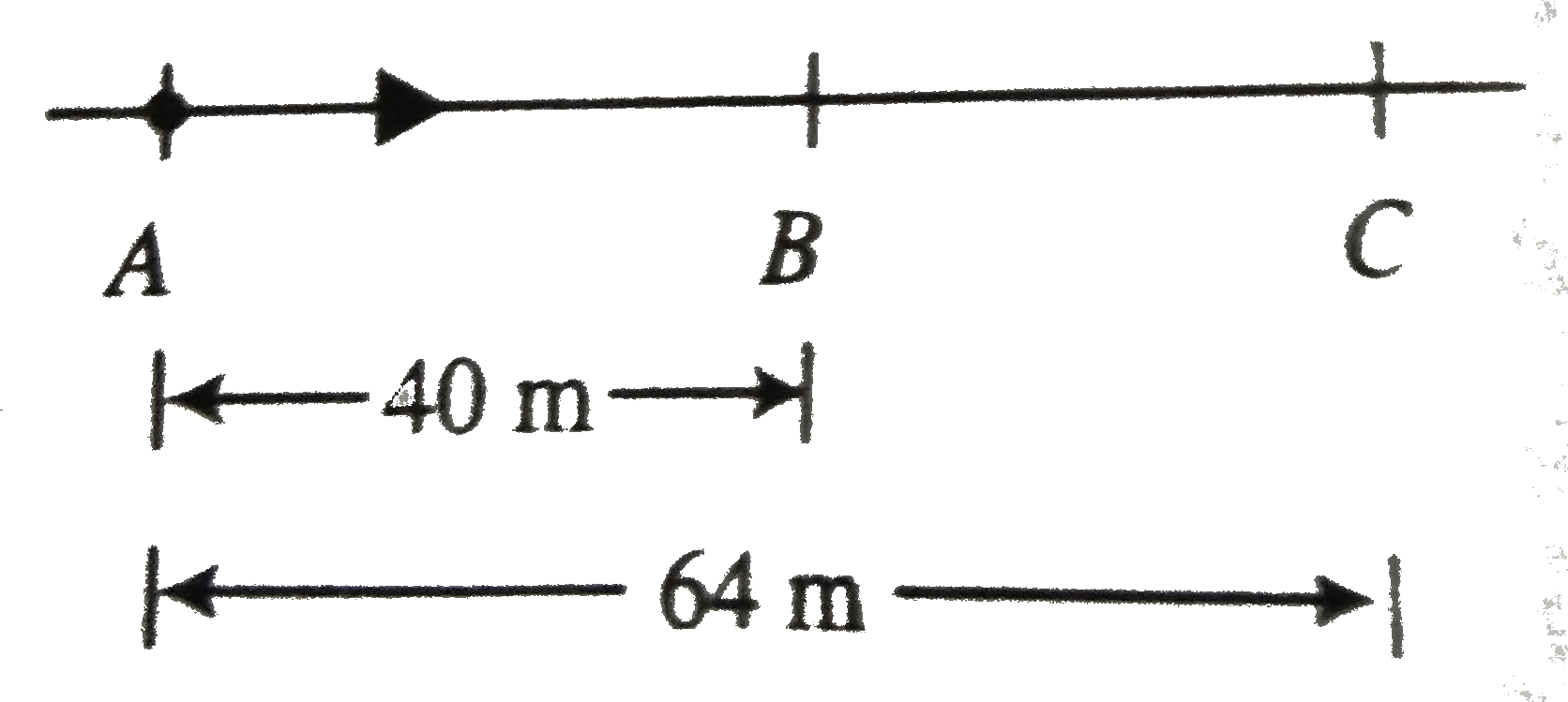 .
.
For motion between A and B
u=12ms−1,s=40 m, t=4 s<br>s=ut+1//2 at^(2)<br>rArr 40=12xx4+(1)/(2)xxaxx(4)^(4) rArra=-1 m s^(-2)<br>Formotionberween A and C<br>64=12t+(1)/(2)(-1)t^(2)<br>rArrt^(2)-24 t+128=0<br>rArr (t-8)(t-16)=0<br> rArr t=8 s, 16 s<br>a.Thepartic≤willbeatCtwo,att=8 sand1=16 s.<br>b.Velocityofthepartic≤atc<br>Att=8 s,velocityofthepartic≤v=12+(-1)xx8<br>=4 m s^(-1)<br>Att=16 s,velpcotyofthepartic≤v=12+(-1) xx16<br>=-4 m s^(-1)<br>Astheae≤rationofthepartic≤is¬ative,itwillrηrdasitmovesalonABC.Atap∮beyondCi.e.,atD,thepartic≤willcome→restmomentarily,andthenitwillmovebackwardwith∈creasingspeed.Throughoutthemotion,thepartic≤dece≤rates,itsvelocitydecrasescont∈uously,velocityvariesas(12,11,10,...,2,1,0,−1,−2,...,−10,−11,−12,)etc.OnlyomA→Dthemotionisrηrded,dur∈gwhichspeedvariesas(12,11,10,...,2,1,0.)<br><imga≤ocr_imageload∈g=lazysrc=https://d10lpgp6xz60nq.cloudfront.net/physics_images/BMS_V01_C04_S01_058_S02.pngwh=80%mx-autosty≤=background:white;>.<br>Subsequently,thepartic≤speedsup,speedchang∈gas(1,2,3,....,)etc.<br>Noticethed⇔erncebetweendece≤rationandrηrda−tion.<br>c.Whenthepartic≤reachesAaga∈,itsdisplacement=0
becuase0=12×t+12(−1)t2
⇒t=0,24s
At t=0, Velocity at A=12ms−1,
At t=24s, velocity at A is
12+(−1)×24=−12ms−1.
d. The particle reveses the direction of motion at D. For the motion between A and D
 .
.
u=12ms−1,v=0,a=−1ms−2. If AD=s, From the equation
v2=u2+2as
(0)2^(2)+2×(−1)×s
s=12×22=72m
You can also calculate the time taken by the particle to reach D from 0=12+(−1)tD and then
from s=12×tD+12(−1)t2D, you can find s.
But then again you must be intersted in optimising the steps of calculation and must not proceed so
c. After 12s the particle comes to rest momentarily at D after covering a distance of 72m.
 .
.
Distance in subsequent 3s
=0×3+12×(−1)×(3)2=4.5m
becuased=72m+4.5m=76.5m.
Topper's Solved these Questions
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.1
17 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Exercise 4.2
12 Videos
- KINEMATICS-1BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-1EXERCISE - Integer
9 Videos
- GRAVITATIONBOOK - CENGAGE PHYSICSCHAPTER - GRAVITATIONEXERCISE - INTEGER_TYPE
1 Video
- KINEMATICS-2BOOK - CENGAGE PHYSICSCHAPTER - KINEMATICS-2EXERCISE - Exercise Integer
9 Videos
Similar Questions
A particle, moving with uniform acceleration along a straight line ABC, crosses point A at t = 0 with a velocity 12 m/s. B is 40 m from A and C is 64 m from A. The particle passes B at t = 4 s.
(a) At what time, will the particle be C?
(b) What is its velocity at C?
View SolutionA particle is moving with uniform acceleration along a straight line "ABC" .Its velocity at A and B are 6m/s" and "9m/s respectively. If AB:BC=5:16" then its velocity at C is
View Solution
Knowledge Check
A particle is moving with uniform acceleration along a straight line ABC. Its velocity at A and B are 6 m/s and 9 m/s respectively if AB:BC=5:16 then its velocity at C is
A9.6m/s2B12m/sC15m/sD21.5m/sA particle is moving with uniform acceleration along a straight line ABC, where AB= BC. The average velocity of the particle from A to B is 10 m/s and from B to C is 15 m/s. The average velocity for the whole journey from A to C in m/s is
A12B12.5C13D13.5A particle moves with uniform acceleration along a straight line AB. Its velocities at AandB are 2m/sand14m/s respectively. M is the mid-point of AB. The particle takes t1 seconds to go from A to M and t2 seconds to go from M to B. Then t2:t1 is.
A1:1B2:1C1:2D3:1
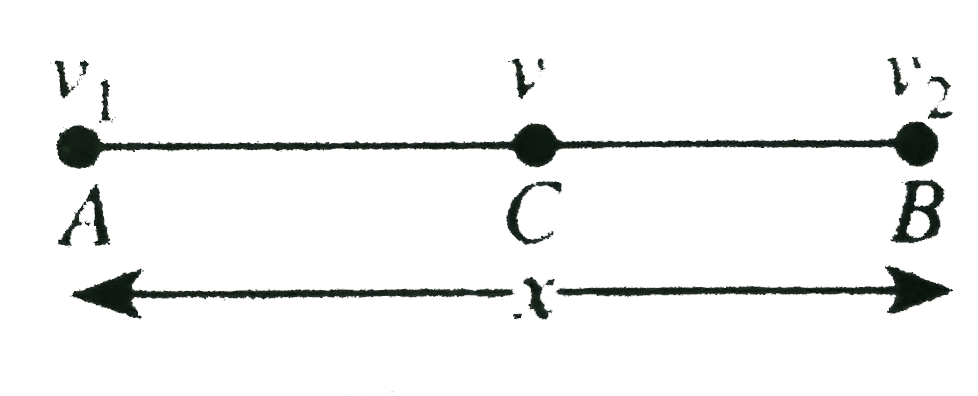
A particle moving with uniform acceleration from A to B along a straight line has velcities v1 and v2 at A and B respectively. If C is the mid-point between A and B then determine the velocity of the particle at C .
 .
.
View SolutionA particle is moving with uniform acceleration along a straight line. The average velocity of the particle from P to Q is 8ms–1 and that Q to S is 12ms–1. If QS = PQ, then the average velocity from P to S is

View SolutionA particle moves with a uniform velocity of 50 m/s for 20 min. What is the velocity of particle at t = 2 min ?
View SolutionA particle is moving with initial velocity 1m/s and acceleration a=(4t+3)ms2. Find velocity of particle at t=2sec.
View Solutiona particle moving along a straight line with uniform acceleration has velocities 7m/s at A and 17m/s at C. B is the mid point of AC. Then :-
View Solution