When a choke coil carrying a steady current is short circuited, the cu

A coil carrying a steady current is short-circuited. The current in it decreases $α$ times in time $t_{0}$ . The time constant of the circuit is
A $τ = t_{0}$ In $α$
B $τ = \frac{t_{0}}{I n α}$
C $τ = \frac{t_{0}}{α}$
D $τ = \frac{t_{0}}{α - 1}$
Consider the circuit shown below. When switch $S_{1}$ is closed, let I be the current at time t, then applying Kirchhoff's law
$E - i R - L \frac{d i}{d t} = 0 or \int_{0}^{i} \frac{d i}{E - i R} = \frac{1}{L} \int_{0}^{1} d t$
$i = \frac{E}{R} (1 - e^{- \frac{R}{l} \cdot t})$
$\frac{L}{R}$ = time constant of circuit
When current reaches its steady value $(= i_{0}$ , open $S_{1}$ and close $S_{2}$ , the current does reach to zero finally but decays expnentially. The decay equation is given as $i = i_{0} e^{- \frac{R}{L} \cdot T}$ ).

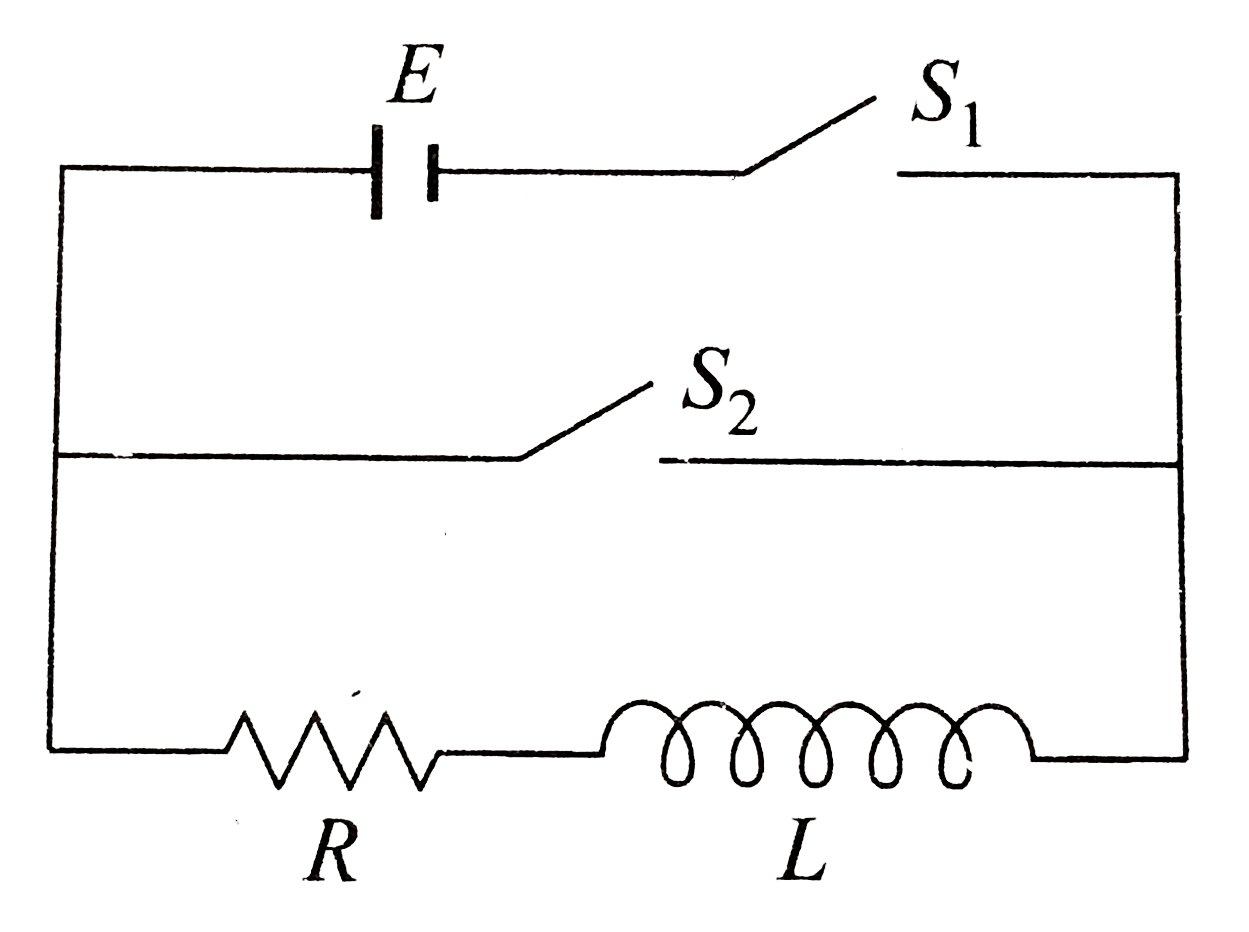
When a coil carrying a steady current is short circuited, the current decreases in it $η$ times in time $t_{0}$ . The time constant of the circuit is
A $t_{0} 1 n η$
B $\frac{t_{0}}{1 n η}$
C $\frac{t_{0}}{η}$
D $\frac{t_{0}}{η} - 1$
At the time of short circuit, the current in the circuit
Areduces substantially
Bdoes not change
Cincreases heavily
Dvaries continuously

When a choke coil carrying a steady current is short circuited, the current in it decreases to $β (< 1)$ times its initial value in a time $T$ . The time constant of the choke coil is