A long cylindrical conductor of radius a has two cylindrical cavities of diameter a through its entire length as shown in cross-section in figure. A current I is directed out of the page and is uniform throughout the cross-section of the conductor. Find the magnitude and direction of the magnetic field in terms of μ0,I,r and a.
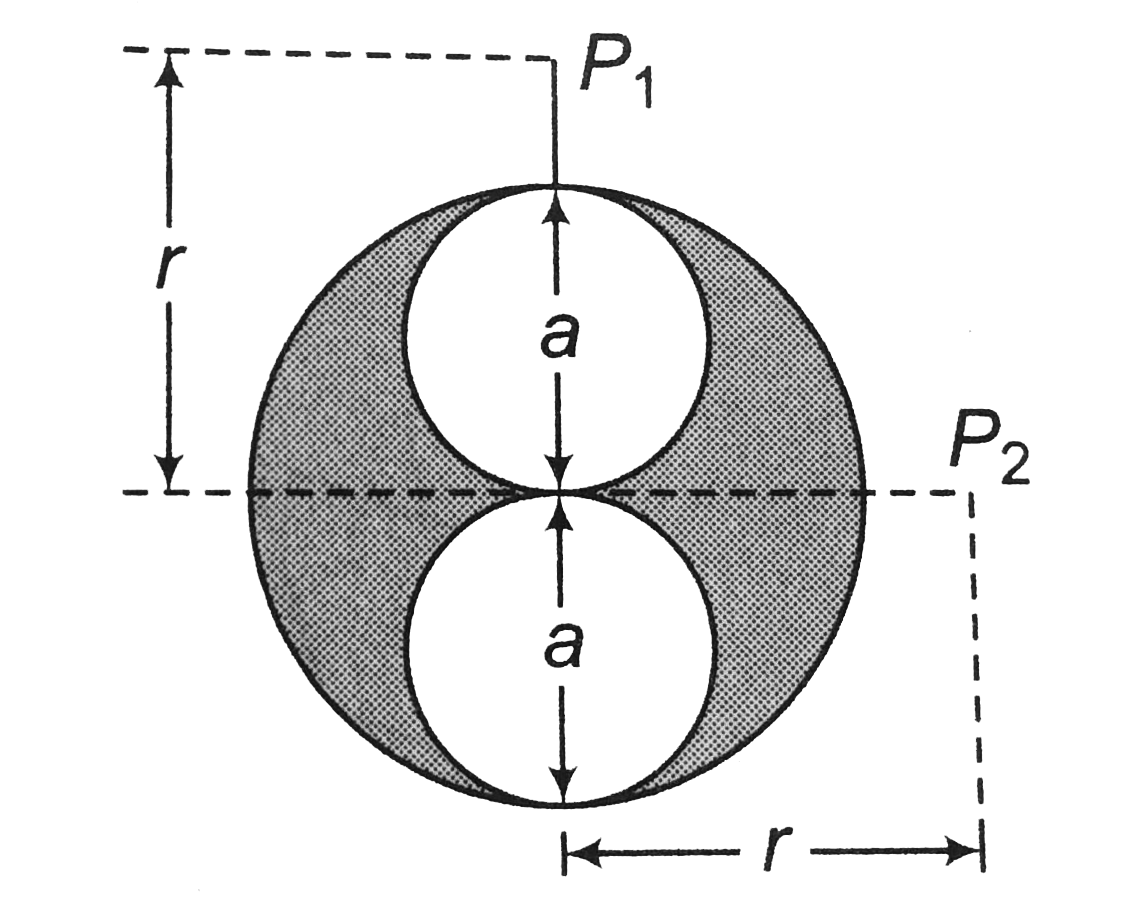
(a) at point P1 and (b) at point P2
The correct Answer is:A, B, D
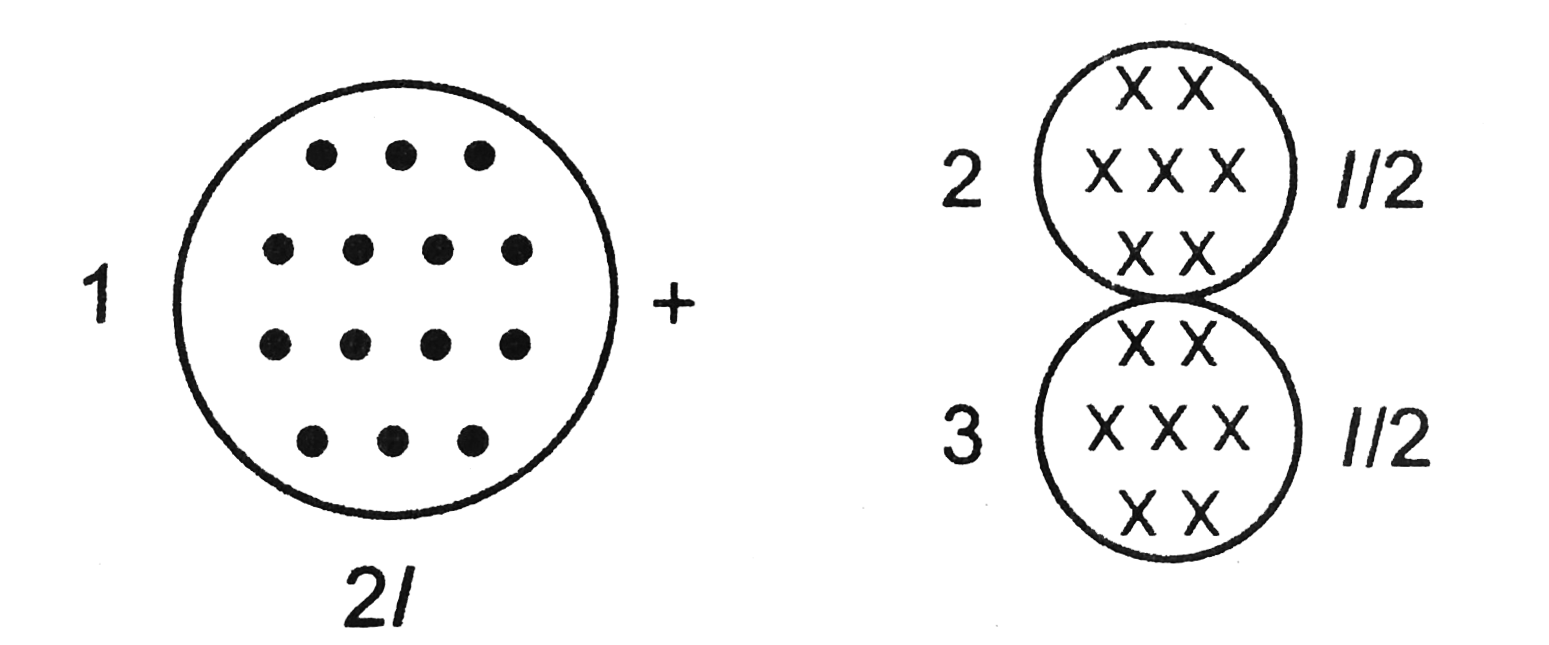
Current per unit area
σ=Iπaw2−π(a2)2−(a2)2
2Iπa2
Total area is (πa2).Therefore the total current is
I1=(σ)(πa2)=2I
Cavity area is π(a2)2 Therefore cavity current is
I2=(sigm)(πa24)=I2
Now the given current system can be assumed as shown below.
a. At P1,B1=μ02π2It=μ0Iπr(towards left)
B2=μ02πI2(r−a2) (towards right)
and B2=μ02πI2(r+a2) (towards right)
∴B≠t=B1−B2−B2 (toward left)
=μ0Iπ[1r−14r−2a−14r+2a]
=μ0Iπ[16r3−4a2−4r2−2ar−4r2+2arr(16r2−4a2)]
=μ0Iπr[2r2−a24r2−a2] (towards left)
b.
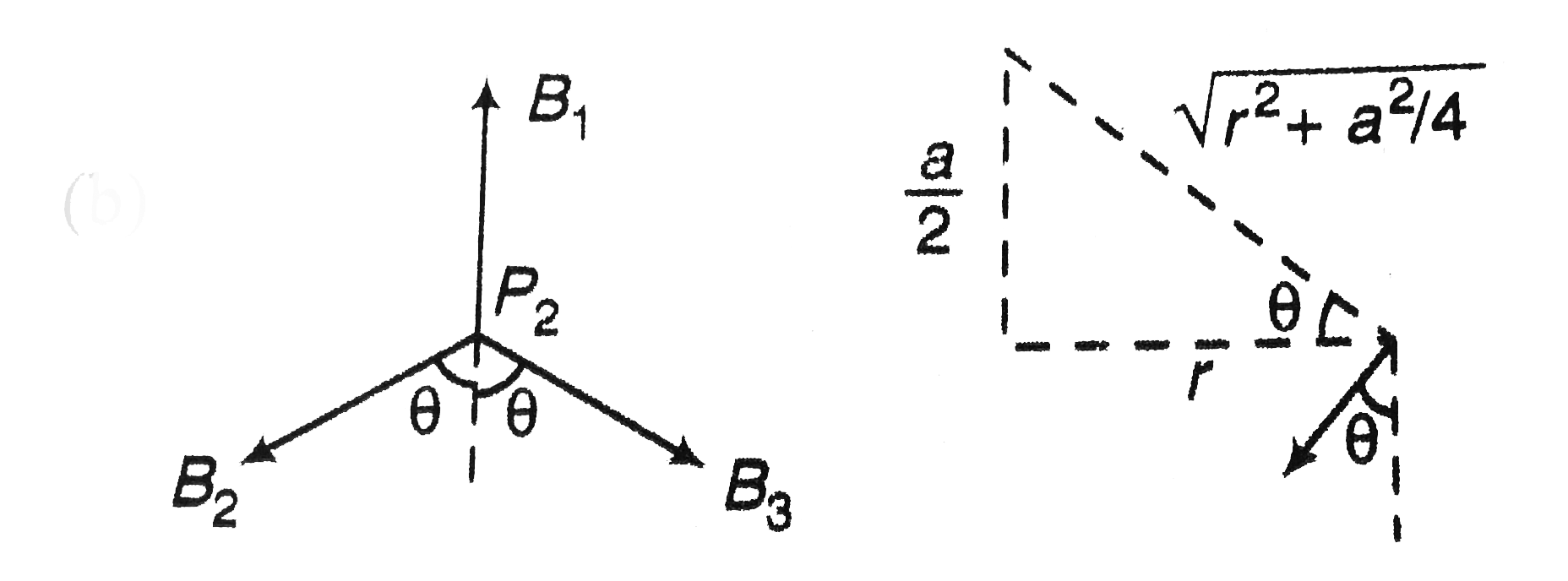
B≠t=B1−2B2cosθ] (towards the top)
=μ02π2IR−2⎡⎢
⎢
⎢
⎢⎣μ02πI2√(r2+a24)⎤⎥
⎥
⎥
⎥⎦r√(r2+a24)
μ0Iπr[2r2+a24r2+a2](towards the top)
Topper's Solved these Questions
- MAGNETICSBOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - INTRODUCTORY EXERCISE
1 Videos
- MAGNETICSBOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - SUBJECTIVE_TYPE
1 Videos
- MAGNETICSBOOK - DC PANDEYCHAPTER - MAGNETICSEXERCISE - MCQ_TYPE
1 Videos
- MAGNETIC FIELD AND FORCESBOOK - DC PANDEYCHAPTER - MAGNETIC FIELD AND FORCESEXERCISE - Medical entrance s gallery
59 Videos
- MAGNETISM AND MATTERBOOK - DC PANDEYCHAPTER - MAGNETISM AND MATTEREXERCISE - Medical gallery
1 Videos
Similar Questions
A long cylindrical conductor of radius R has two cylindrical cavities of diameter R through its entire length, as shown in the figure. There is a current I through the conductor distributed uniformly in its entire cross section (apart from the cavity region). Find magnetic field at point P at a distance r=2R from the axis of the conductor (see figure).

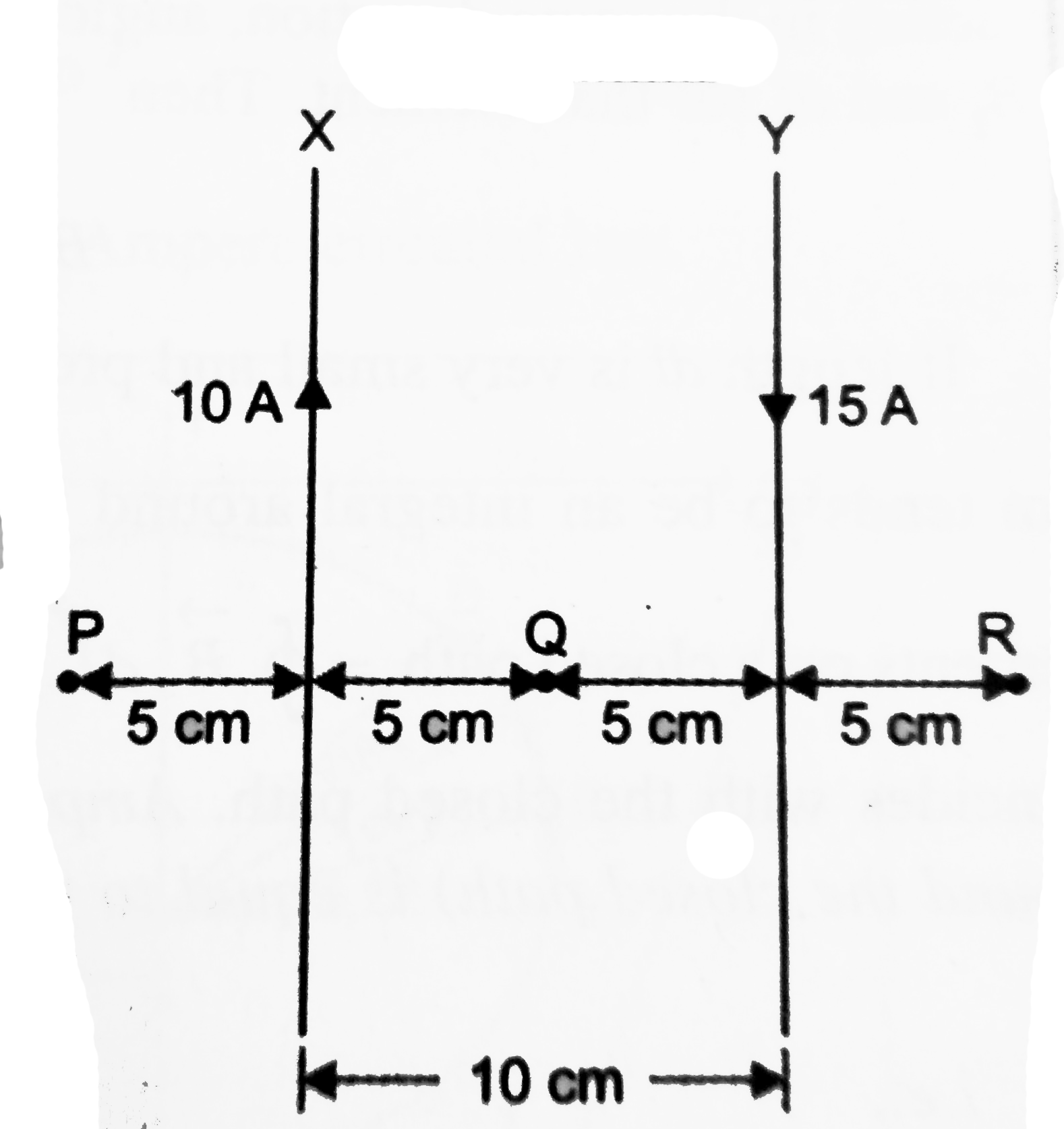
View SolutionThere are two parallel current carrying wires X and Y as shown in figure. Find the magnitude and direction of the magnetic field at points, P, Q and R.
View Solution
Knowledge Check
A long cylindrical conductor of radius R having a cylindrical cavity of radius R/2 through out its length is lying with its axis parallel to x-axis. The distance between the axes of the cylinder and the cavity length in the positive x direction. The across sectional view of the cylinder is shown in the figure
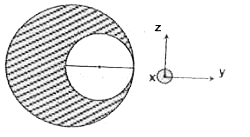
The magnetic induction at point on the cavity isAμ0I3πR(−ˆl)Bμ0i3πR(ˆk)Cμ0i6πR(ˆj)Dμ0I6πR(−ˆj)
Two infinite plates shown in cross-section in figure carry λ amperes of current out of thepage per unit width of plate. Find the magnetic field at points P and Q.
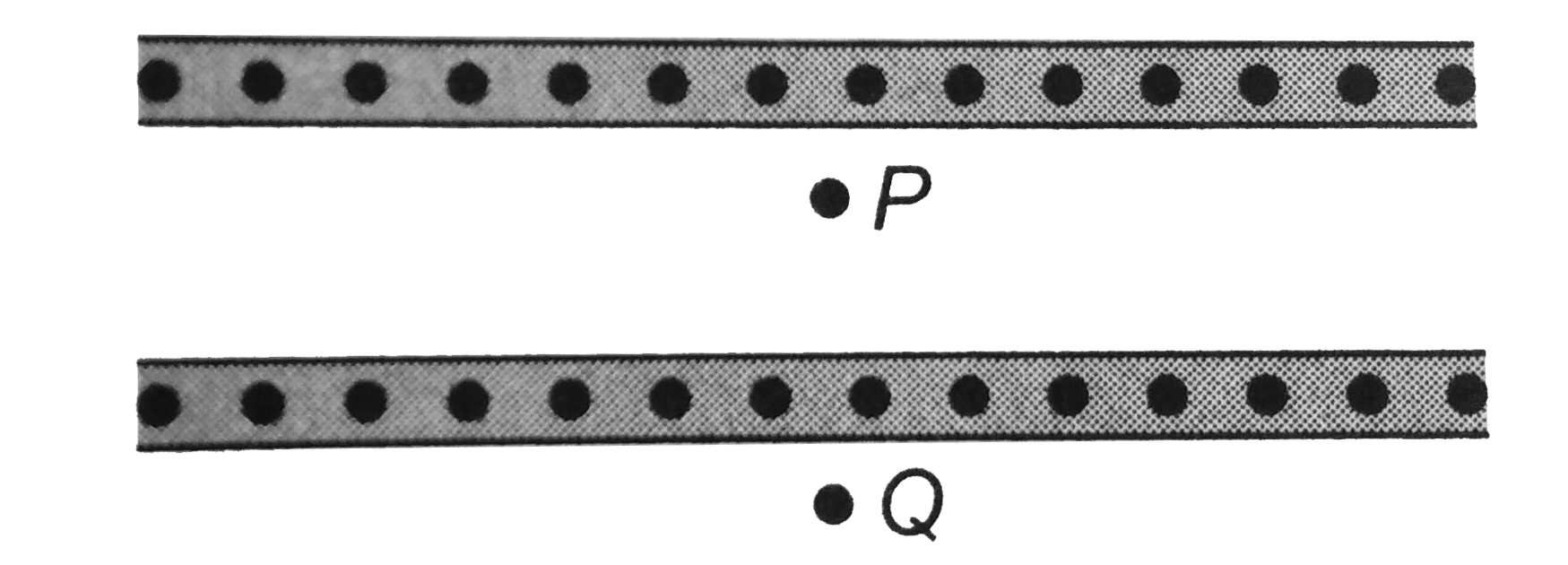
View SolutionIn a long insulating cylinder of radius R a cylindrical tunnel of radius a located at a distance b from the axis of the cylinder as shown in the figure. R R b - The solid part of the cylinder has a uniform volume charge density P. Find the magnitude and direction of the electric field inside the tunnel.
View SolutionIn the figure are shown two long parallel current carrying wires I and II. Find the magnitudes and directions of the magnetic field induction at the point P, Q and R in the plane of paper.

View SolutionCalculate the magnitude of the magnetic field at point P as shown in Fig, in terms of R,I1andI2.

View SolutionFigure shows a cross-section of a long ribbon of width ω that is carrying a uniformly distributed total current i into the page. Calculate the magnitude and direction of the magnetic field B at a point P in the plane of the ribbon at a distance d from its edge.
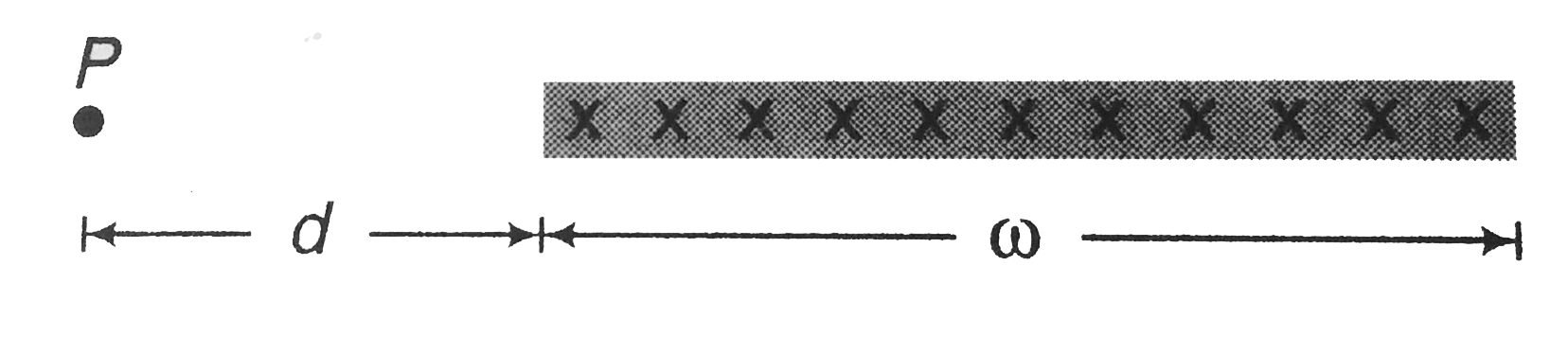
View SolutionAn electric current of I ampere is flowing in a long conductor CG as shown in figure. Find the magnitude and direction of magnetic induction at the centre O of circular part.
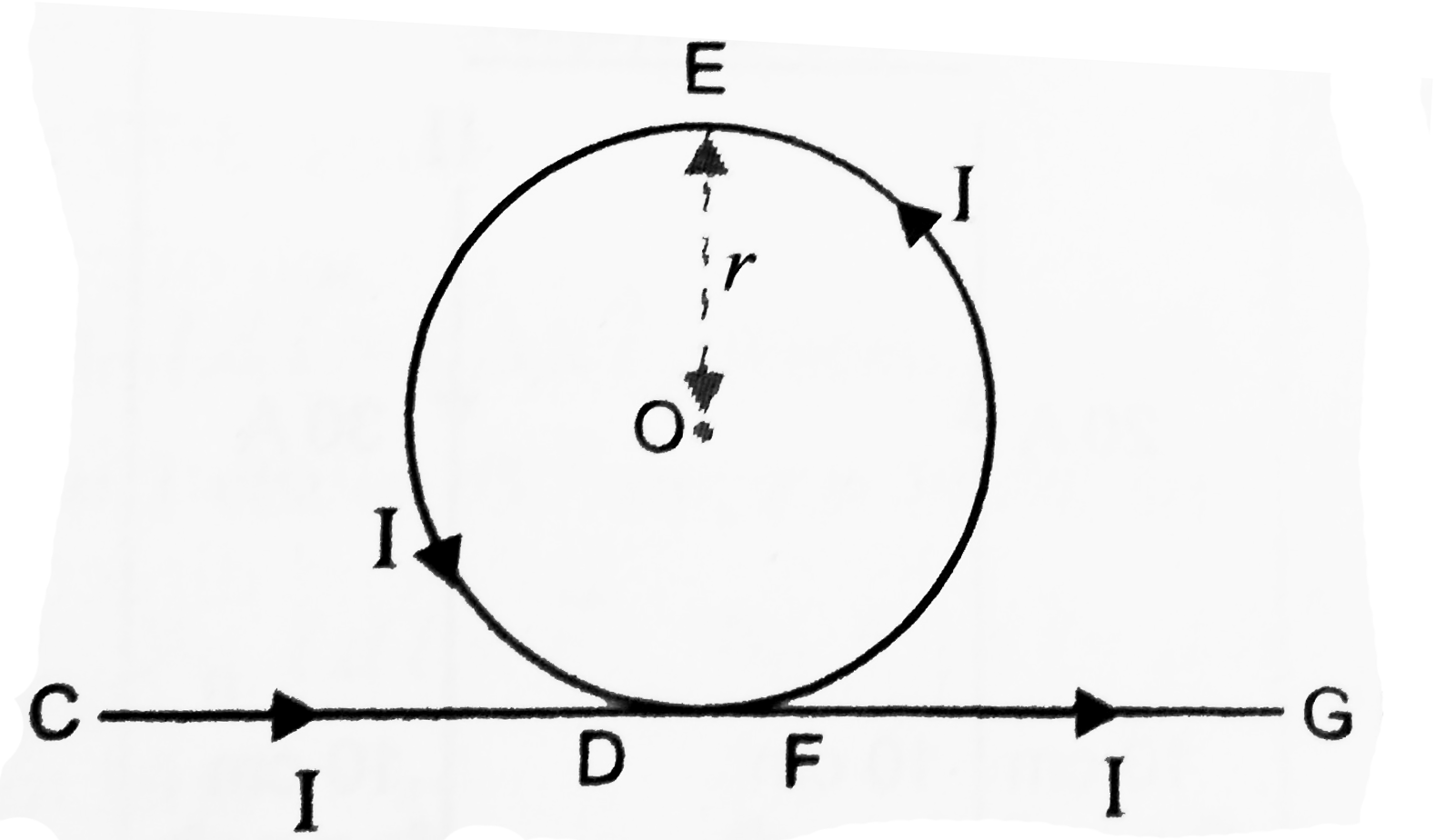
View SolutionA current I flows in a long straight wire whose cross section is in the shape of a thin quarter ring of radius R. Find the induction of the magnetic field (B) at point O on the axis.

View Solution
