Potential difference beween centre and surface of the sphere of radius R and uniorm volume charge density ρ within it will be
ρR26ε0
ρR24ε0
ρR23ε0
ρR22ε0
The correct Answer is:A
To find the potential difference between the center and the surface of a sphere with radius R and uniform volume charge density ρ, we can follow these steps:
Step 1: Calculate the total charge Q inside the sphere
The total charge Q within a sphere of radius R with uniform volume charge density ρ can be calculated using the formula:
Q=ρ⋅V
where V is the volume of the sphere given by:
V=43πR3
Thus, we have:
Q=ρ⋅43πR3
Step 2: Calculate the electric potential Vc at the center of the sphere
The electric potential Vc at the center of a uniformly charged sphere can be calculated using the formula:
Vc=14πϵ0⋅QR
Substituting the expression for Q:
Vc=14πϵ0⋅ρ⋅43πR3R
This simplifies to:
Vc=ρ3ϵ0R
Step 3: Calculate the electric potential Vs at the surface of the sphere
The electric potential Vs at the surface of the sphere (radius R) is given by:
Vs=14πϵ0⋅QR
Again substituting for Q:
Vs=14πϵ0⋅ρ⋅43πR3R
This simplifies to:
Vs=ρ3ϵ0R
Step 4: Calculate the potential difference Vdiff
The potential difference Vdiff between the center and the surface of the sphere is given by:
Vdiff=Vc−Vs
Substituting the values of Vc and Vs:
Vdiff=(ρ3ϵ0R)−(ρ3ϵ0R)=0
Conclusion
The potential difference between the center and the surface of the sphere is:
Vdiff=0
The total charge Q within a sphere of radius R with uniform volume charge density ρ can be calculated using the formula:
Q=ρ⋅V
where V is the volume of the sphere given by:
V=43πR3
Thus, we have:
Q=ρ⋅43πR3
The electric potential Vc at the center of a uniformly charged sphere can be calculated using the formula:
Vc=14πϵ0⋅QR
Substituting the expression for Q:
Vc=14πϵ0⋅ρ⋅43πR3R
This simplifies to:
Vc=ρ3ϵ0R
The electric potential Vs at the surface of the sphere (radius R) is given by:
Vs=14πϵ0⋅QR
Again substituting for Q:
Vs=14πϵ0⋅ρ⋅43πR3R
This simplifies to:
Vs=ρ3ϵ0R
The potential difference Vdiff between the center and the surface of the sphere is given by:
Vdiff=Vc−Vs
Substituting the values of Vc and Vs:
Vdiff=(ρ3ϵ0R)−(ρ3ϵ0R)=0
The potential difference between the center and the surface of the sphere is:
Vdiff=0
Topper's Solved these Questions
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.1
4 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Exercise 24.2
9 Videos
- ELECTROSTATICSBOOK - DC PANDEYCHAPTER - ELECTROSTATICSEXERCISE - Example Type 8
3 Videos
- ELECTROSTATIC POTENTIAL AND CAPACITORSBOOK - DC PANDEYCHAPTER - ELECTROSTATIC POTENTIAL AND CAPACITORSEXERCISE - (C) Chapter exercises
50 Videos
- GRAVITATIONBOOK - DC PANDEYCHAPTER - GRAVITATIONEXERCISE - All Questions
120 Videos
Similar Questions
A smooth chute is made in a dielectric sphere of radius R and uniform volume charge density. ρ. A charge particle of mass m and charge -q is placed at the centre of the sphere. Find the time period of motion of the particle?

View SolutionThere is a hemisphere of radius R having a uniform volume charge density ρ. Find the electric potential and field at the centre
View Solution
Knowledge Check
An insulating solid sphere of radius R has a uniformly positive charge density ρ. As a result of this uniform charge distribution there is a finite value of electric potential at the centre of the sphere, at the surface of the sphere and also at a point out side the sphere. The electric potential at infinity is zero.
Statement-1: When a charge 'q' is taken from the centre to the surface of the sphere, its potential energy changes by qρ3∈0
Statement-2 : The electric field at a distance r(r<R) from the centre of the the sphere is ρr3∈0A(a) Statement 1 is true, Statement 2 is true, Statement 2 is not the correct explanation of statement 1.B(b) Statement 1 is true Statement 2 is false.C(c) Statement 1 is false Statement 2 is true.D(d) Statement 1 is true, Statement 2 is true, Statement 2 is the correct explanation of Statement 1Potential at a point x-distance from the centre inside the conducting sphere of radius R and charged with charge Q is
AKQRBKQxCQx2DxQA hollow charged metal sphere has radius r. If the potential difference between its surface and a point at a distance 3r from the centre is V, then electric field intensity at a distance 3r is
AV6rBV4rCV3rDV2r
A system consists of a uniformly charged sphere of radius R and a surrounding by a medium with volume charge density ρ=ar, where α is a positive constant and r is the distance from the centre of the sphere. If NαR2 is the charge on the sphere when the electric field intensity outside the sphere is independent of r. What is the value of N ?
View SolutionRepeat the question if sphere is a hollow non-conducting sphere of radius R and has uniform surface charge density σ.
View SolutionFind the electric field strength vector at the centre of a ball of raiduius R with volume charge density ρ⇒ar, where a is a constant vector, and r is a radius vector drawn from the ball's centre.
View SolutionA hollow charged metal sphere has radius r. If the potential difference between its surface and a point at a distance 3r from the centre is V, then electric field intensity at a distance 3r is
View SolutionA nonconducting sphere of radius R is filled with uniform volume charge density −ρ. The center of this sphere is displaced from the origin by →d. The electric field →E at any point P having position vector inside the sphere is
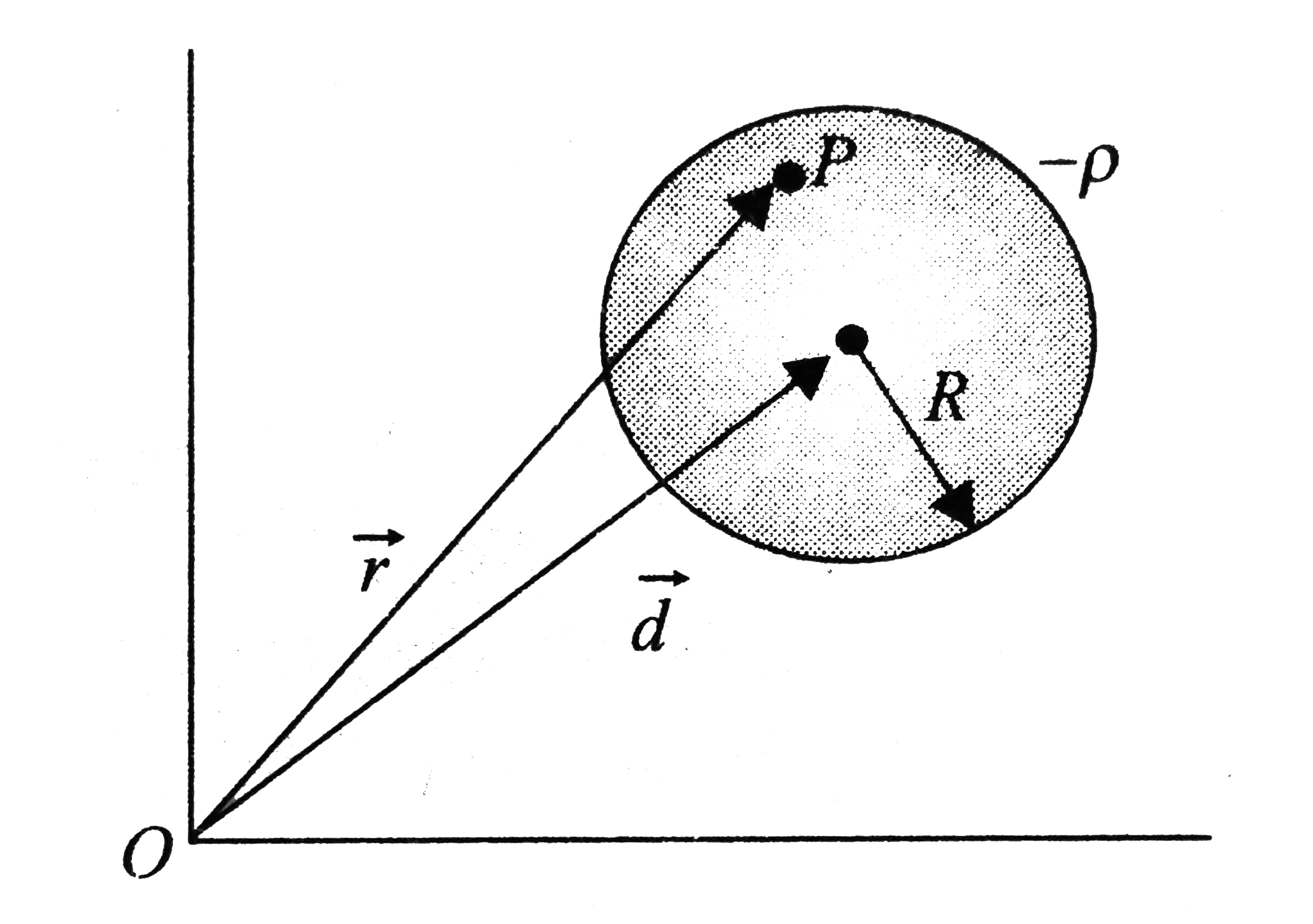
View Solution