Four point charges $+ 8 μ C, - 1 μ C, - 1 μ C$ and , $+ 8 μ C$ are fixed at the points $- \sqrt{27 / 2} m, - \sqrt{3 / 2} m, + \sqrt{3 / 2} m$
and $+ \sqrt{27 / 2} m$ respectively on the y-axis. A particle of mass $6 \times 10^{- 4} k g$ and $+ 0.1 μ C$ moves along the x-direction. Its speed at $x = + \infty$ is $v_{0}$ . find the least value of $v_{0}$ for which the particle will cross the origin. find also the kinetic energy of the particle at the origin in tyhis case. Assume that there is no force part from electrostatic force.

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A, C, D

In the figure
$q = μ C = 10^{- 6} C$
$q_{0} = + 0.1 μ C = 10^{- 7} C$
$m = 6 \times 10^{- 4} k g$
and $Q = 8 μ C = 8 \time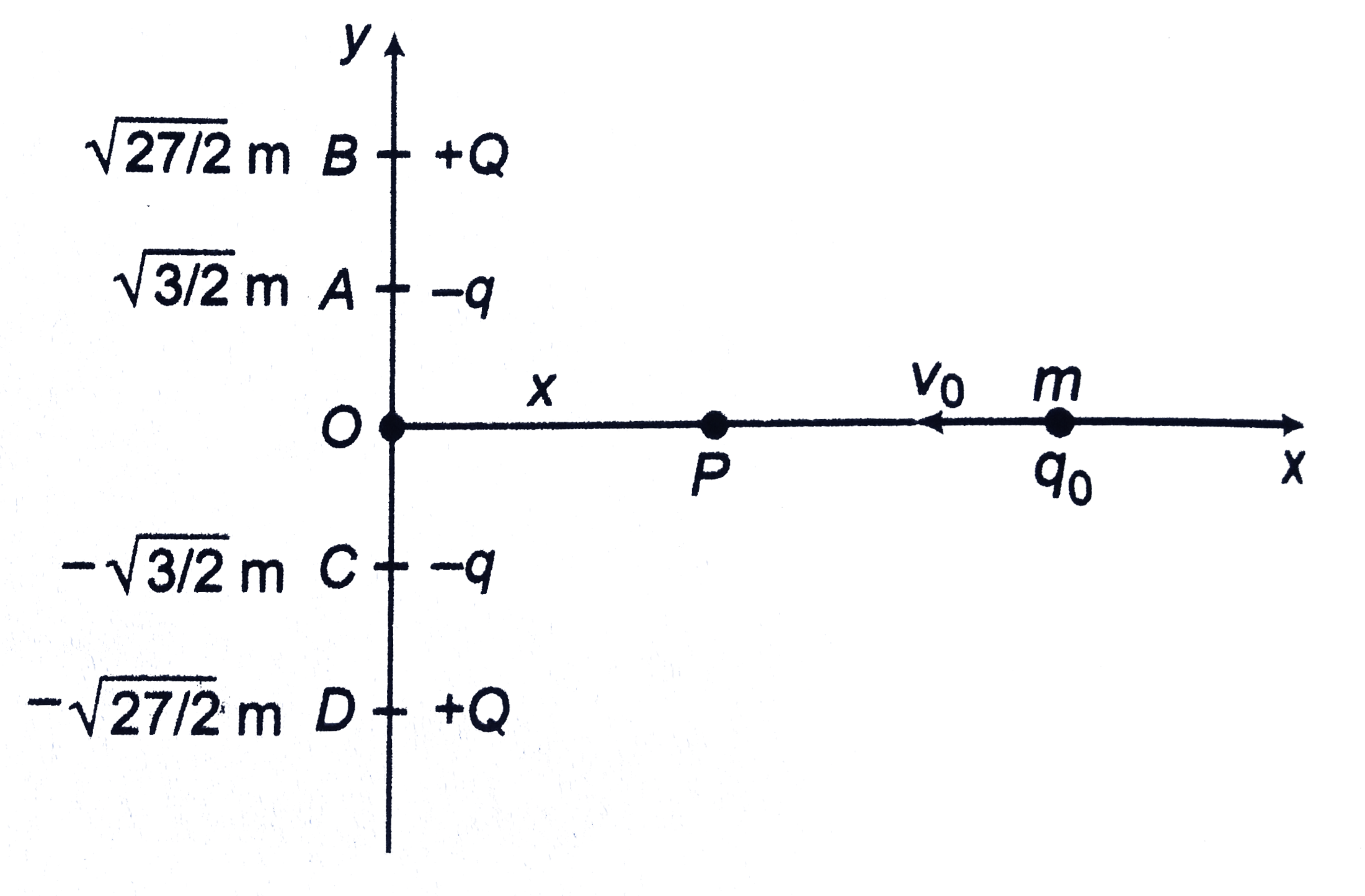s 10^{- 6} C$
Let $P$ be any point at a distance x from origin O.
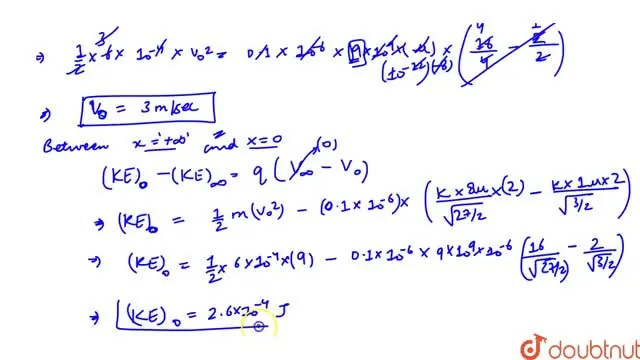
$T h e n < b r >$ AP=CP=sqrt(3/2)+x^2 $< b r >$ BP=DP=sqrt(27/2+x^2) $< b r > E \leq c t r i c p o t e n t i a l a t p \oint P w i l l b e < b r >$ V=(2kQ)/(BP)-(2kq)/(AP) $< b r > w h e r e,$ k=1/(4piepsilon_0)=9xx10^-9Nm^2//C^2 $< b r >$ V=2xx9xx10^9[(8xx10^-6)/(sqrt(27/2+x^2))-10^(-6)/(sqrt((3/2+x^2)))] $< b r >$ V=1.8xx10^4[8/(sqrt(27/2+x^2))-1/(sqrt((3/2+x^2)))] $... ... ... . . i < b r >$ :. $E \leq c t r i c f i e l d a t P i s < b r >$ E=-(dV)/(dx)=-1.8xx10^4[(8)(-1/2)(27/2+x^2)^(-3//2)-(1)(-1/2)(3/2+x^2)^(-3//2)](2x) $< b r >$ E=0 $o n x - a ξ s w h e r e < b r >$ 8/((27/2+x^2)^(3//2))=1/((3/+x^2)^/(3//2) $< b r >$ (27/2+x^2)=((4))^(3/2+x^2) $< b r > T h i s e q u a t i o n g i v e s$ x=+-sqrt(5/2)m $< b r > T h e \leq * v a l u e o f k \in e t i c e \neq r g y o f t h e p a r t i c \leq a t \in f \in i t y s h o d - - b e e n o u g h \to t a k e t h e p a r t i c \leq u p \to$ x=+sqrt(5/2)m $∵ < b r > a t$ x=+sqrt(5/2)m,E=0implies $E \leq c t r o s a t i c f or c e o n c h a r \geq$ q_0 $i s z e r o or$ F_e=0 $< b r > f or$ xltsqrt(5/2) $m, E i s i s a r a c t i v e (\to w a r d s \neg a t i v e x - a ξ s) < b r > N o w, o m e q n i p o t e n t i a l a t$ x=sqrt(5/2)m $< b r >$ V=1.8xx10^4[8/sqrt(27/2+5/2)-1/sqrt(3/2+5/2) $< b r >$ V=2.7xx10^4V $< b r > A p p l y \in g e \neq r g y c o n s e r v a t i o n a t$ x=oo $and$ x=sqrt(5/2)m $< b r >$ 1/2mv_0^2=q_0V $< b r >$ :. v_0=sqrt((2q_0V)/m) $< b r > S u b s t i t u t \in g t h e v a l u e s$ v_0=sqrt((2xx10^-7xx2.7xx10^4)/(6xx10^-4)) $< b r >$ v_0=3m/s $< b r >$ :. $M \in i μ m v a l u e o f$ v_0 $i s$ 3/m/s $. < b r > F r o m e q n i p o t e n t i a l a t or i g \in$ (x=0) $i s < b r >$ V_0=1.8xx10^4[8/(sqrt27/2)-1/sqrt(3/2)]=2.4xx10^4V $< b r > L e t T b e t h e k \in e t i c e \neq r g y o f t h e p a r t i c \leq a t or i g \in . < b r > A p p l y \in g e \neq r g y c o n s e r v a t i o n a t$ x=0 $and a t$ x=oo $< b r > T + q_{0} V_{0} = \frac{1}{2} m v_{0}^{2}$
But $\frac{1}{2} m v_{0}^{2} = v_{0} V$ [from eqn ii]
$∴ T = q_{0} (V - V_{0})$
$T + (10^{- 7}) (2.7 \times 10^{4} - 2.4 \times 10^{4})$
$T = 3 \times 10^{- 4} J$

Updated on:21/07/2023

|

Class 12 PHYSICS ELECTRIC CHARGES AND FIELDS

Topper's Solved these Questions

Similar Questions

Knowledge Check

Four point charges q, -2q, -q and 2q are placed at points (0,0,0) m , $(\sqrt{2}, 0, 0) m, (\sqrt{2}, \sqrt{2}, 0) m and (0, \sqrt{2}, 0)$ m respectively . The electric field at point $(\frac{1}{\sqrt{2}}, \frac{1}{\sqrt{2}}, 0) m is (q = 1 μ C)$
A $18 \times 10^{3} N / C$
B $36 \times 10^{3} N / C$
C $18 \sqrt{5} \times 10^{3} N / C$
D $18 \sqrt{3} \times 10^{3} N / C$
Three point charges $1 μ C, 1 μ C and - 2 μ C$ are placed at points having coordinates (0,1,0)cm, (1,0,0) cm and (0,0,0)cm respectively. The magnitude of electric dipole moment of the system is
A $10^{- 8} C m$
B $\sqrt{2} \times 10^{- 8} C m$
C $\sqrt{3} \times 10^{- 8} C m$
D $2 \times 10^{- 8} C m$
Electric charges of $+ 10 μ C, + 5 μ C, - 3 μ C and + 8 μ C$ are placed at the corners of a square of side $\sqrt{2}$ m, the potential at the centre of the square is
A1.8 V
B $1.8 \times 10^{6} V$
C $1.8 \times 10^{5} V$
D $1.8 \times 10^{4} V$

DC PANDEY-ELECTROSTATICS-Level 2 Single Correct

Home
Ask Doubt
Profile