A block of mass m is placed at rest on a horizontal rought surface with angle of friction ϕ. The block is pulley with a force F at an angle θ with the horizontal. The minimum value of F required to move the block is
mgsinϕcos(θ−ϕ)
mgcosϕcos(θ−ϕ)
mgtanϕ
mgsinϕ
The correct Answer is:A
μ=tanϕ
N=mg−Fsinθ

Fcosθ=μN=(tanϕ)(mg−Fsinθ)
=(sinϕcosϕ)(mg−Fsinθ)
On solving this equation,we get
F=mgsinϕcos(θ−ϕ)
Topper's Solved these Questions
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Objective Question
32 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Comprehension
9 Videos
- LAWS OF MOTIONBOOK - DC PANDEYCHAPTER - LAWS OF MOTIONEXERCISE - Exercise 8.5
2 Videos
- KINEMATICS 1BOOK - DC PANDEYCHAPTER - KINEMATICS 1EXERCISE - INTEGER_TYPE
15 Videos
- LAWS OF THERMODYNAMICSBOOK - DC PANDEYCHAPTER - LAWS OF THERMODYNAMICSEXERCISE - Level 2 Subjective
18 Videos
Similar Questions
A block of mass m is attached with a massless spring of force constant K. the block is placed over a rough inclined surface for which the coefficient of friction is μ=34 find the minimum value of M required to move the block up the place. (Neglect mass of string and pulley. Ignore friction in pulley).
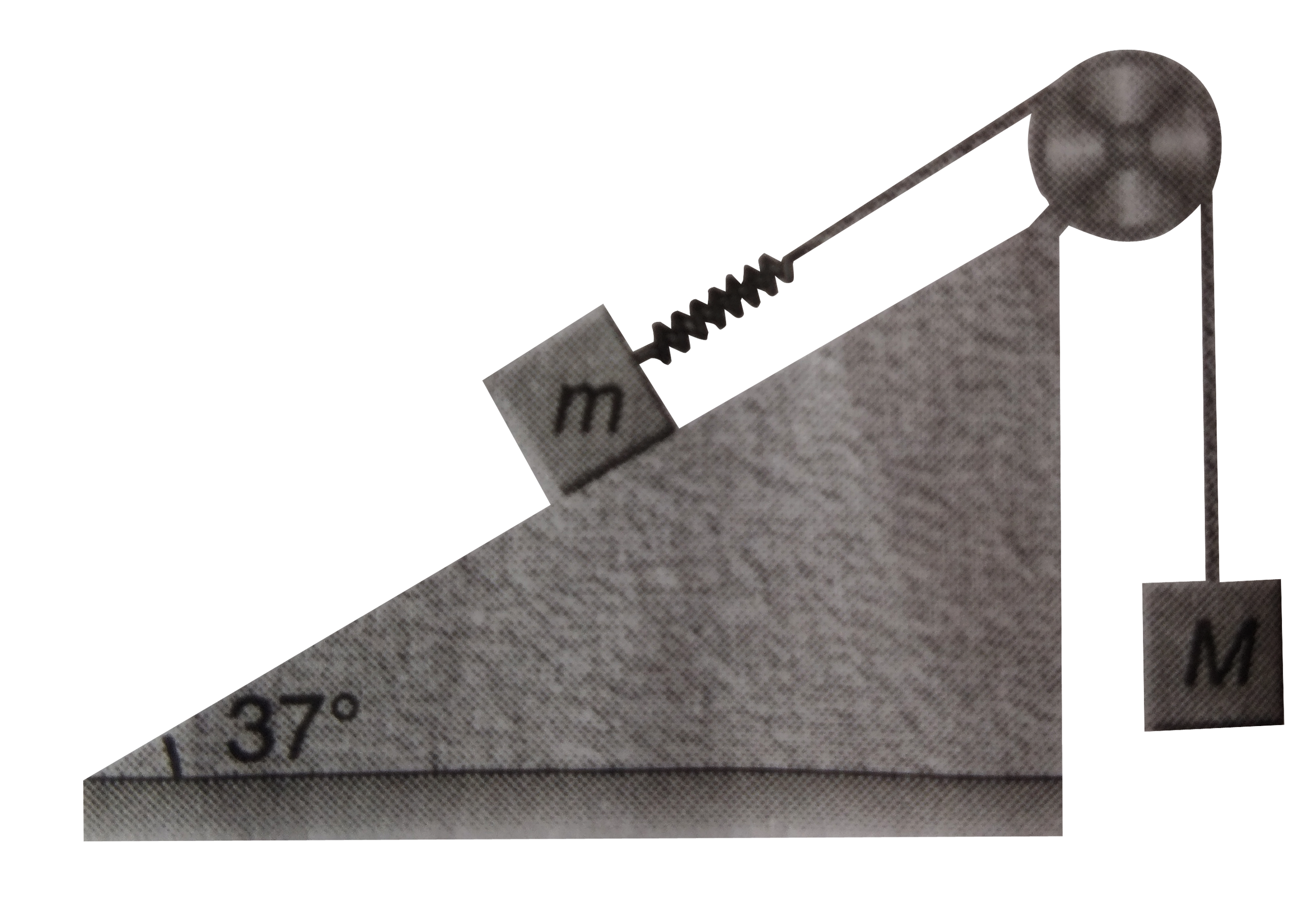 .
.
View SolutionA block of mass m lying on a horizontal surface (coefficient of static friction =μs) is to be brought into motion by a pulling force F At what angle θ with the horizontal should the force F be applied so that its magnitude is minimum ? Also find the minimum magnitude

View Solution
Knowledge Check
Two block mandM tied together with an inextensible string are placed at rest on a rought horizontal surface with coefficient of friction μ The block m is pulled with a variable force F at a varying angle θ with the horizontal .The value of θ at which the least value of F is required to move the blocks given by
 Aθ=tan−1μBθ>tan−1μCθ<tan−1μDInsufficient data
Aθ=tan−1μBθ>tan−1μCθ<tan−1μDInsufficient dataA block of mass m is lying on horizontal surface of coefficient of friction μ. A force is applied to the block at an angle θ with the horizontal. The block will move with a minimum force F if
Aμ=tanθBμ=cosθCμ=sinθDμ=cotθA block of mass m is placed on a horizontla surface. If the block is pulled by applying a force of magnitude F=5mg at an angle θ=37∘, with horizontal as shown in fig. find the acceleration of the block at the given instant.
 A5√5gB3√5gC2√3gD2√5g
A5√5gB3√5gC2√3gD2√5g
A block of mass m is placed on a rough horizontal surface. If minimum force to pull the block is mg2 then coefficient of friction is :
View SolutionA block of mass M is at rest on a rough horizontal surface. The coefficient of friction between the block and the surface is μ. A force F = Mg acting at an angle θ with the vertical side of the block pulls it. In which of the following cases, the block can be pulled along the surface?
View SolutionA block of mass m is attached with a massless spring of force constant k. The block is placed over a rough inclined surface for which the coefficient of friction is μ=34. The minimum value of M required to move the block up the plane is (Neglect mass of string, mass of pulley and friction in pulley)
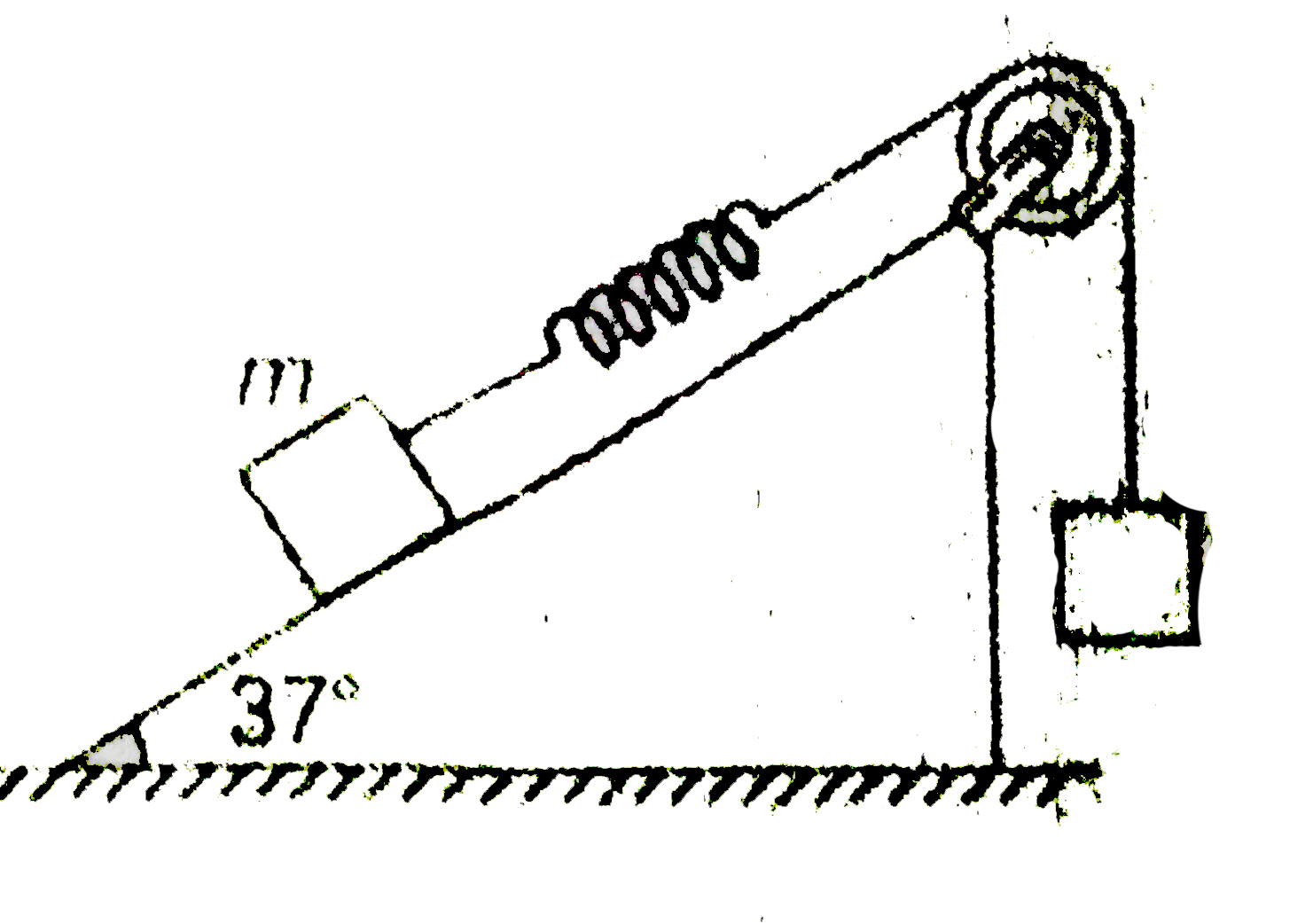
View SolutionA wooden block of mass M resting on a rough horizontal surface is pulled with a force F at an angle with the horizontal. If is the coefficient of kinetic friction between the block and the surface, then acceleration of the block is -
View SolutionA block of mass 10 kg is placed on the rough horizontal surface. A pulling force F is acting on the block which makes an angle θ above the horizontal. If coefficient of friction between block and surface is 43 then minimum value of force required to just move the block is (g=10ms2)
View Solution
