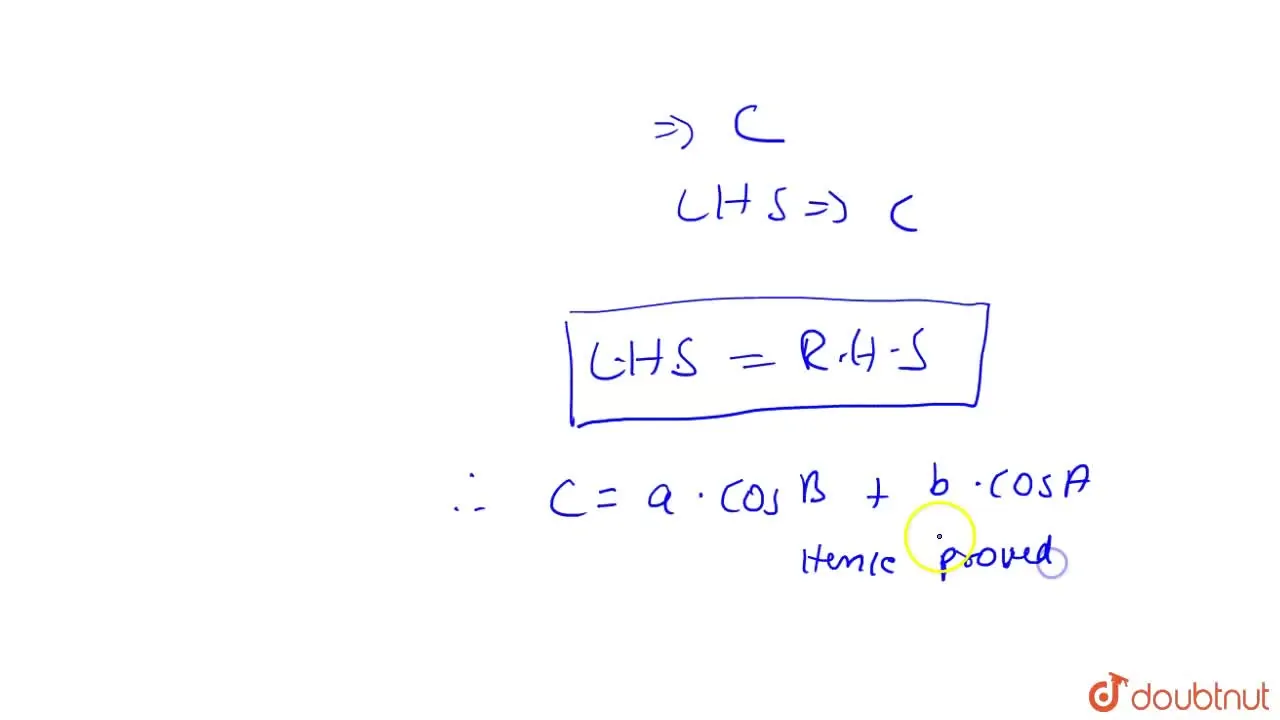
If any triangle ABC with usual notations prove $c = a cos B + b cos A$ .

Video Solution

Text Solution

Verified by Experts

By cosine rule and with usual notations, we have
$b^{2} = c^{2} + a^{2} - 2 c a cos B$ .
$∴ cos B = \frac{c^{2} + a^{2} - b^{2}}{2 c a}$
Similarly, $cos A = \frac{b^{2} + c^{2} - a^{2}}{2 b c}$
R.H.S. $= a \cdot cos B + b \cdot cos A$
$= a \cdot (\frac{c^{2} + a^{2} - b^{2}}{2 c a}) + b \cdot (\frac{b^{2} + c^{2} - a^{2}}{2 b c})$
$= \frac{c^{2} + a^{2} - b^{2}}{2 c} + \frac{b^{2} + c^{2} - a^{2}}{2 c}$
$= \frac{c^{2} + a^{2} - b^{2} + b^{2} + c^{2} - a^{2}}{2 c}$
$= \frac{2 c^{2}}{2 c}$
$= c =$ L.H.S.
$∴ c = a \cdot cos B + b \cdot cos A$ Hence Proved.

Updated on:21/07/2023

In a $Δ A B C$ with usual notations, if $\frac{a}{cos A} = \frac{b}{cos B} = \frac{c}{cos C}$ , then the triangle is :
Aright angled
Bisosceles
Cobtuse angled
Dequilateral
Given $\frac{b + c}{11} = \frac{c + a}{12} = \frac{a + b}{13}$ for a $Δ A B C$ with usual notation. If $\frac{cos A}{α} = \frac{cos B}{β} = \frac{cos C}{γ}$ , then the ordered triad $(α, β, γ)$ has a value:
A(3, 4, 5)
B(5, 12, 13)
C(7, 19, 25)
D(19, 7, 25)