In ΔABC , with usual notations prove that :
b2=c2+a2−2cacosB.
Video Solution
Text Solution
Consider that for ΔABC,∠B is in a standed
position i.e. vertex B is at the origin and the side BC
is along positive x-axis. As ∠B is an angle of a triangle .
∴∠B can be acute or obtuse
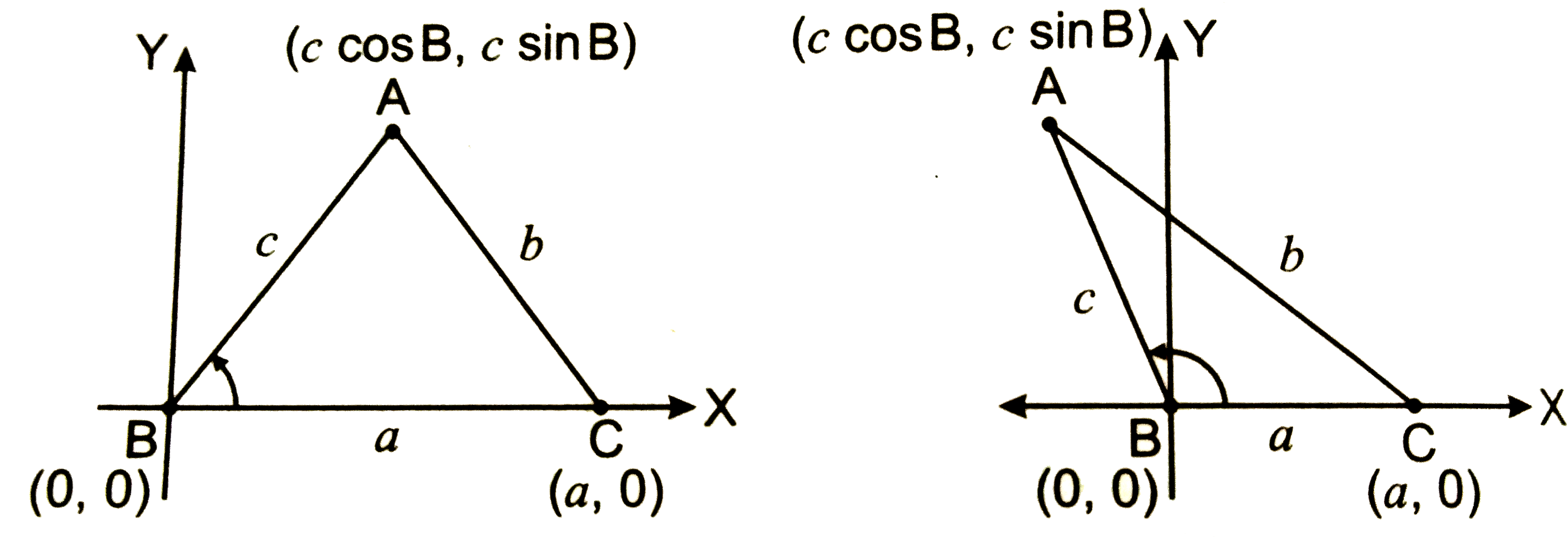
From cartesian co-ordinates of 1 and 2 we get .
B≡(0,0),A≡(ccosB,csinB)andC≡(a,0)
Now consider l(CA)=b
∴l(CA)2=b2
∴b2=(a−ccosB)2+(0−csinB)2
" " ... [By distance formula]
=a2−2ca cos B+c2cos2B+c2sin2B
a2−2ca cos B+c2(cos2B+sin2B)
a2−2cacosB+c2
∴b2=c2+a2−2ca cos B
|
Topper's Solved these Questions
- MARCH 2019BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2019EXERCISE - SECTION-C
8 Videos
- MARCH 2018BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - MARCH 2018EXERCISE - SECTION - II
20 Videos
- OCTOBER 2014BOOK - GURUKUL PUBLICATION - MAHARASHTRA PREVIOUS YEAR PAPERSCHAPTER - OCTOBER 2014EXERCISE - SECTION - II
19 Videos
Similar Questions
Knowledge Check
With usual notations, in triangle ABC, acos(B−C)+bcos(C−A)+ccos(A−B) is equal to
AabcR2Babc4R2C4abcR2Dabc2R2
In a △ABC , with usual notations , prove that a−bcosCb−acosC=cosBcosA.
View SolutionIn △ABC , with the usual notations , prove that 2{asin2.C2+csin2.A2}=a+c−b
View SolutionWith usual notations prove that in a triangle ABC, cot(A2)+cot(B2)+cot(C2)=s2Δ
View SolutionIf in a △ABC with usual notation, a(a−c)=b2−c2 ,then 1572(sin2(πcos2B)) is equal to
View SolutionIn ΔABC prove that a2+b2+c2=2(bccosA+cacosB+abcosC)
View SolutionIn any ΔABC, if B=600, then prove that (c−a)2=b2−ca
View SolutionIn ΔABC with usual notation r1bc+r2ca+r3ab is
View Solution
