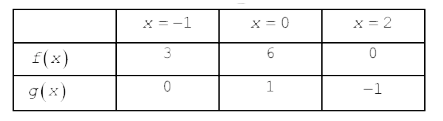
Let f,g:[−1,2]→R be continuous functions which are twice differentiable on the interval (-1, 2). Let the values of f and g at the points –1, 0 and 2 be as given in the following table : x=−1x=0x=2f(x)360g(x)01−1 In each of the intervals (-1,0) and (0, 2) the function (f – 3g)'' never vanishes. Then the correct statement(s) is(are)
f'(x)−3g'(x)=0 has exactly three solutions in (−1,0)∪(0,2)
f'(x)−3g'(x)=0 has exactly one solution in (−1,0)
f'(x)−3g'(x)=0 has exactly one solution in (0, 2)
f'(x)−3g'(x)=0 has exactly two solution in (-1, 0) and exactly two solutions in (0,2)
The correct Answer is:B, C
Let F (x) = f(x) -3 g(x)
∴F(−1)=3,F(0)=3andF(2)=3
So, F'(x) will vanish atleast twice in (−1,)∪(0,2).
∵F''(x)>or0,∀x∈(−1,0)∪(0,2)
Hence, f'(x)−3g'(x)=0 has exactly one solution in (-1, 0) and one solution in (0, 2) .
Related Playlists
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - LIMIT,CONTINUITY AND DIFFERENTIABILITY EXERCISE - Differentiability at a point ( Assertion and Reason)1videos
LIMIT,CONTINUITY AND DIFFERENTIABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - LIMIT,CONTINUITY AND DIFFERENTIABILITY EXERCISE - Differentiability at a point (Match the Columns)2videos
LIMIT,CONTINUITY AND DIFFERENTIABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - LIMIT,CONTINUITY AND DIFFERENTIABILITY EXERCISE - Differentiability at a point ( Objective Questions I)25videos
LIMIT,CONTINUITY AND DIFFERENTIABILITY
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - JEE MAINSEXERCISE - All Questions1videos
JEE MAINS
- BOOK - IIT JEE PREVIOUS YEARCHAPTER - MATRICES AND DETERMINANTSEXERCISE - SOLVING SYSTEM OF EQUATIONS (INTEGER ANSWER TYPE QUESTION)2videos
MATRICES AND DETERMINANTS
Similar Questions
Let f,g:[−1,2]→ be continuous functions which are twice differentiable on the interval (-1,2). Let the values of f and g at the points and 2 be as given in the following table:
In each of the intervals (-1,0) and (0, 2) the function (f-3g) never vanishes. Then the correct statements(s) is(are) :View SolutionLet f be any continuous function on [0, 2] and twice differentiable on (0, 2). If f(0) = 0, f(1) = 1 and f(2) = 2, then :
View SolutionLet f:[0,1] → R be a function . Suppose the fuction f is twice differentiable with f(0) =f(1)=0 and satisfies
f(x)−2f(x)+f(x)≥ex for all x∈[0,1].Which of the following is true for x ∈(0,1] ?View SolutionLet f(x)=cot−1g(x)] where g(x) is an increasing function on the interval (0,π) Then f(x) is
View SolutionLet f(x), be a function which is continuous in closed interval [0, 1] and differentiable in open interval ] 0,1[. Show that ∃ a point c∈]0,1[ such that f'(c)=f(1)−f(0).
View SolutionLet f:[0,1]→R (the set of all real numbers) be a function. Suppose the function f is twice differentiable, f(0)=f(1)=0andsatiiesf\'\'(x)-2f\'(x)+f(x) ge e^x, x in [0,1]Whichofthefollow∈gistruefor0 lt x lt 1?(A)0 lt f(x) lt oo(B)-1/2 lt f(x) lt 1/2(C)-1/4 lt f(x) lt 1(D)-oo lt f(x) lt 0
View SolutionA function f(x) is, continuous in the closed interval [0,1] and differentiable in the open interval [0,1] prove that f'(x1)=f(1)−f(0),0<x1<1
View SolutionIf f(0)=f(1)=f(2)=0 and function f (x) is twice differentiable in (0, 2) and continuous in [0, 2]. Then, which of the following is(are) true ?
View SolutionLet f(x) be a twice differentiable function in (−∞,∞) such that f''(x)<0∀x∈R, g(x)=f(x)+f(1-x) and g'((1)/(4))=0 then
View SolutionLet f:[0,1]→R be a continuous function then the maximum value of ∫10f(x).x2dx−∈10x.(f(x))2dx for all such function(s) is:
View Solution


