A cavity of radius R/2 is made inside a solid sphere of radius R. The centre of the cavity is located at a distance R/2 from the centre of the sphere. The gravitational force on a particle of a mass 'm' at a distance R/2 from the centre of the sphere on the line joining both the centres of sphere and cavity is (opposite to the centre of cavity). [Here g=GM/R2, where M is the mass of the solid sphere]
mg2
3mg8
mg16
none of these
The correct Answer is:B
Gravitational field at mass 'm' due to full solid sphere
→E1=ρ→r3ε0=ρR6ε0............[ε0=14πG]
Gravitational field at mass m due to cavity (−ρ)
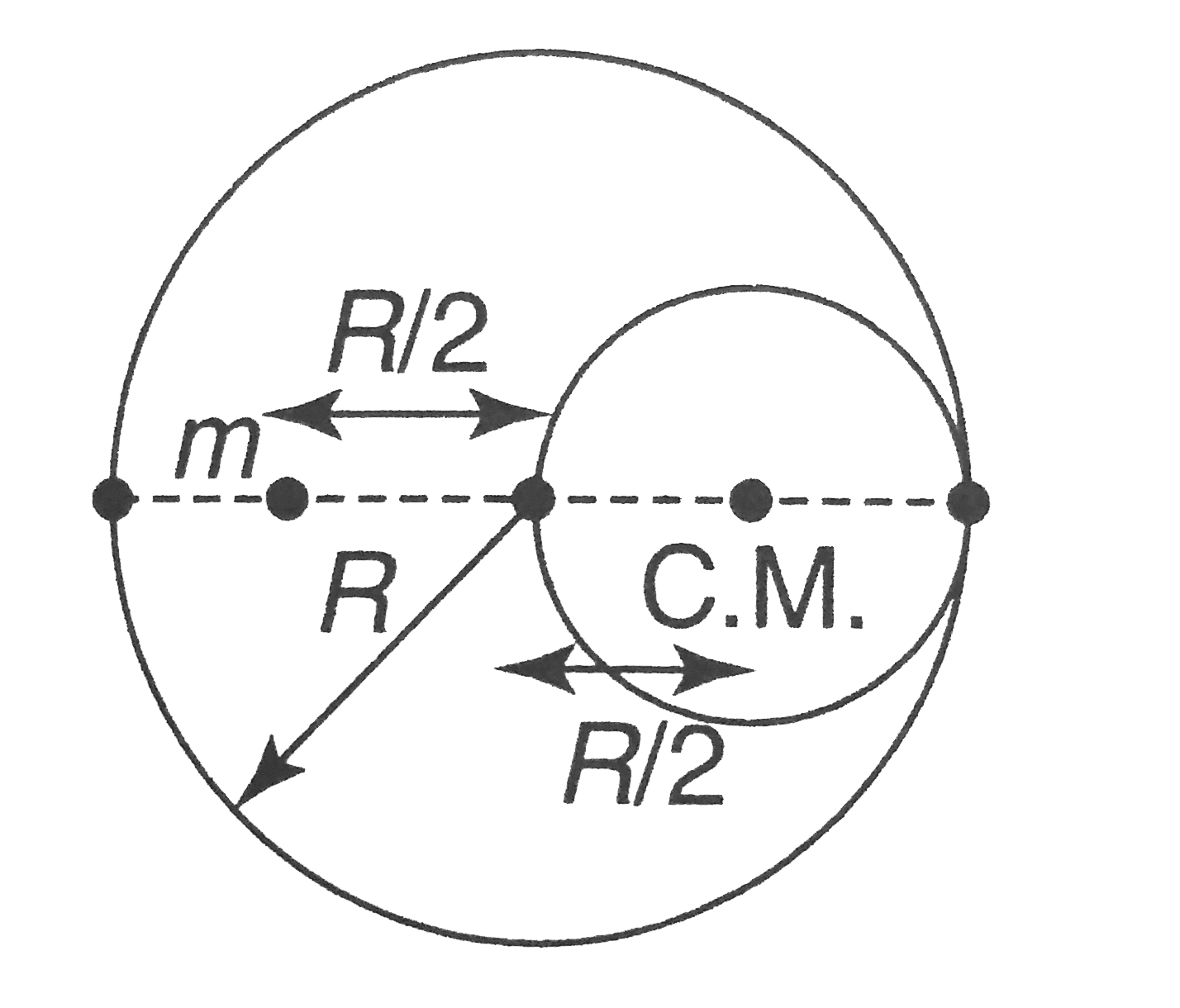
→E2=(−ρ)(R/2)33ε0R2......[ρa33ε0r2]
=−(−ρ)R324ε0R2=−−ρR24ε0
Net gravitational field
→E=→E1+→E2=ρR6ε0−ρR24ε0=ρR8ε0
Net force on 'm'→F=m→E=mρR8ε0
Here, ρ=M4/3πR3&ε0=14πG
Then F=3mg8
Related Playlists
- BOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Assertion Reasoning16videos
GRAVITATION
- BOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - NEET Questions42videos
GRAVITATION
- BOOK - A2ZCHAPTER - GRAVITATIONEXERCISE - Motion Of Satellite33videos
GRAVITATION
- BOOK - A2ZCHAPTER - GENERAL KINEMATICS AND MOTION IN ONE DIMENSIONEXERCISE - Chapter Test30videos
GENERAL KINEMATICS AND MOTION IN ONE DIMENSION
- BOOK - A2ZCHAPTER - KINETIC THEORY OF GASES AND THERMODYNAMICSEXERCISE - Chapter Test29videos
KINETIC THEORY OF GASES AND THERMODYNAMICS
Similar Questions
A cavity of radius R/2 is made inside a solid sphere of radius R. The centre of the cavity is located at a distance R/2 from the centre of the sphere. The gravitational force on a particle of mass m at a distance R/2 from the centre of the sphere on the line joining both the centre of the cavity)
[Here g=(GM)/R2, where M is the mass of the sphereView SolutionA spherical cavity of radius R2 is made in a sphere of radius R and mass M. The centre of the cavity is at a distance R2 from the centre of the sphere. The intensity of gravitational field at the centre of cavity is GMnR2. Find n.
View SolutionThe potential at a distance R/2 from the centre of a conducting sphere of radius R will be
View SolutionThe figure represents a solid uniform sphere of mass M and radius R. A spherical cavity of radius r is at a distance a from the centre of the sphere. The gravitational field inside the cavity is
 View Solution
View SolutionA solid sphere of mass M and radius R has a spherical cavity of radius R/2 such that the centre of cavity is at a distance R/2 from the centre of the sphere. A point mass m is placed inside the cavity at a distance R/4 from the centre of sphere. The gravitational force on mass m is
View SolutionInside a uniform sphere of density ρ there is a spherical cavity whose centre is at a distance l from the centre of the sphere. Find the strength of the gravitational field inside the cavity.
View SolutionThe radius of gyration of a solid sphere of radius r about a certain axis is r. The distance of this axis from the centre of the sphere is
View SolutionA particle moving on the inside of a smooth sphere of radius r describing a horizontal circle at a distance r/2 below the centre of the sphere. What is its speed ?
View SolutionA hemispherical cavity of radius R is created in a solid sphere of radius 2R as shown in the figure . Then y -coordinate of the centre of mass of the remaining sphere is
 View Solution
View SolutionInside a uniform sphere of density ρ there is a spherical cavity whose centre is at a distance l from the centre of the sphere. Find the strength G of the gravitational field inside the cavity.
View Solution


