Let X be the number of successes in 'n' independent Bernoulli trials with probability of success $p = \frac{3}{4}$ , the least value of 'n' so that $P (x \geq 1) \geq 0.9375$ is

A

2

B

1

C

4

D

3

Video Solution

Text Solution

Verified by Experts

The correct Answer is:A

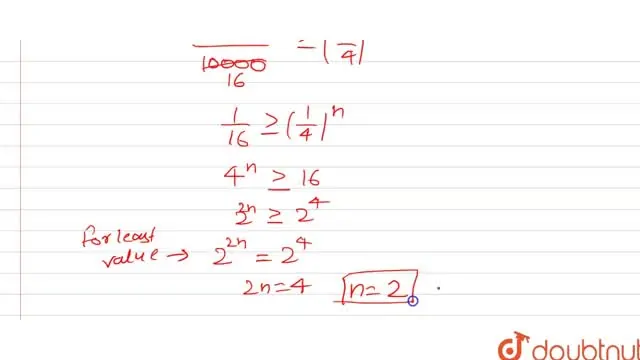
We have, $p = \frac{3}{4}, q k = 1 - p = \frac{1}{4}$
it is given that $P (X \geq 1) \geq 0.9375$
$= 1 - P (x = 0) \geq 0.9375 = 1 - .^{n} C_{0} (p^{0}) {(g)}^{n - 0} \geq 0.9375$
$= 1 - {(\frac{1}{4})}^{n} \geq 0.9375 = 1 - 0.9375 \geq {(\frac{1}{4})}^{n} = 0.0625 \geq {(\frac{1}{4})}^{n}$
$= \frac{625}{10000} \geq {(\frac{1}{4})}^{n} = \frac{1}{16} \geq {(\frac{1}{4})}^{n} = 16 \leq 4^{n}$
$\Rightarrow n = 2$

|

Updated on:21/07/2023

Class 12 MATHS QUESTION BANK

Topper's Solved these Questions

Similar Questions

Knowledge Check

Question 1 - Select One
Consider 5 independent Bernoulli trials each with probability of success p. If the probability of at least one failure is greater than or equal to $\frac{31}{2}$ , then p lies in the interval
A $(\frac{3}{4}, \frac{11}{12}]$
B $[0, \frac{1}{2}]$
C $(\frac{11}{12}, 11]$
D $(\frac{1}{2}, \frac{3}{4}]$
Question 2 - Select One
Consider 5 independent Bernoulli.s trials each with probability of success p. If the probability of at least one failure is greater than or equal to $\frac{31}{32}$ , then p lies in the interval : (1) $(\frac{1}{2}, \frac{3}{4}]$ (2) $(\frac{3}{4}, \frac{11}{12}]$ (3) $[0, \frac{1}{2}]$ (4) $(\frac{11}{12}, 1]$
A $(\frac{1}{2}, \frac{3}{4})$
B $(\frac{3}{4}, \frac{11}{12})$
C $(0, \frac{1}{2})$
D $(\frac{11}{12}, 1)$
Question 3 - Select One
If there are n independent trials, p and q are the probability of success and failure respectively, then probability of exactly r success
A $q^{n}$
B $^{n} C_{r} q^{n} p^{r}$
C $^{n} C_{r} q^{n - r} p^{r}$
D $^{n} C_{r} p^{n - r} q^{r}$

MHTCET PREVIOUS YEAR PAPERS AND PRACTICE PAPERS-SOLVED PAPER 2019-MCQS

Home
Ask Doubt
Profile