Question

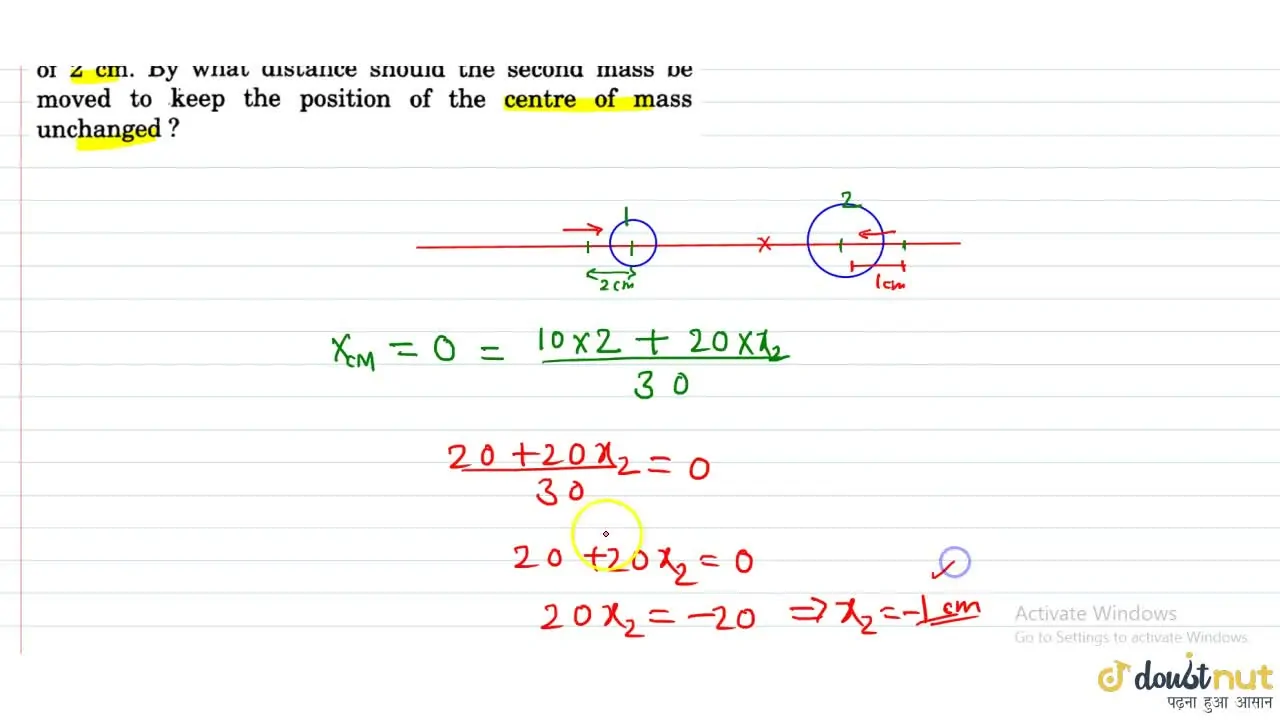
Two bocks of masses 10 g and 20 kg are placed on the X-axis. The first mass is moved on the axis by a distance of 2 cm. By what distance should the second mass be moved to keep the position of the centre of mass unchanged?

Explanation

To solve the problem, we need to find out how far the second mass (20 kg) should be moved to keep the center of mass of the system unchanged after moving the first mass (10 g) by 2 cm.

1. Identify the masses and their initial positions:
- Let the mass \( m_1 = 10 \, \text{g} = 0.01 \, \text{kg} \) (since we need to keep units consistent).
- Let the mass \( m_2 = 20 \, \text{kg} \).
- Assume the initial position of \( m_1 \) is at \( x_1 = 0 \, \text{cm} \) and \( m_2 \) is at \( x_2 = 0 \, \text{cm} \).

2. Calculate the initial center of mass (CM):
\( x_{CM, \text{initial}} = \frac{m_1 x_1 + m_2 x_2}{m_1 + m_2} \)
Substituting the values:
\( x_{CM, \text{initial}} = \frac{(0.01 \, \text{kg} \cdot 0) + (20 \, \text{kg} \cdot 0)}{0.01 \, \text{kg} + 20 \, \text{kg}} = 0 \)

3. Move the first mass:
- The first mass is moved 2 cm to the right. So, the new position of \( m_1 \) becomes \( x_1' = 2 \, \text{cm} \).

4. Calculate the new center of mass after moving \( m_1 \):
\( x_{CM, \text{new}} = \frac{m_1 x_1' + m_2 x_2}{m_1 + m_2} \)
Substituting the new position:
\( x_{CM, \text{new}} = \frac{(0.01 \, \text{kg} \cdot 2 \, \text{cm}) + (20 \, \text{kg} \cdot 0)}{0.01 \, \text{kg} + 20 \, \text{kg}} = \frac{0.02}{20.01} \approx 0.000999 \, \text{cm} \)

5. Set the new center of mass equal to the initial center of mass:
To keep the center of mass unchanged, we need:
\( x_{CM, \text{new}} = x_{CM, \text{initial}} = 0 \)

6. Let the second mass be moved by a distance \( x_2' \):
- If we move the second mass to the right by \( x_2' \), the new position of \( m_2 \) becomes \( x_2' = x_2 + x_2' = 0 + x_2' \).

7. Set up the equation for the center of mass:
\( 0 = \frac{(0.01 \cdot 2) + (20 \cdot x_2')}{20.01} \)
Rearranging gives:
\( 0.02 + 20x_2' = 0 \)
\( 20x_2' = -0.02 \)
\( x_2' = -\frac{0.02}{20} = -0.001 \, \text{cm} = -1 \, \text{cm} \)

Conclusion:
The second mass (20 kg) should be moved 1 cm to the left to keep the position of the center of mass unchanged.