Consider the function f(x) = 2x + 3 and we want to find the limit of this function at x = 1.
Video Solution
Text Solution
Let us complete the value of the function for value of x very near to 1.
First we consider values of x approaching 1 from the left i.e., x<1
x0.50.70.80.90.950.99f(x)=2x+344.44.64.84.94.98
Now, we consider values of X approaching 1 from the right ie x>1
x1.00011.0011.011.051.11.2f(x)=2x+35.00025.0025.025.15.25.4
From both the tables, we observe that value of f(x) at x = 1 should be greater than 4.98 than and less than 5.0002
So, it is reasonable to assume that value of f(x) at x = 1 as dictated by the numbers to the left of 1 is 5
i.e., limf(x)=5x→1
Similarly, when x approaches 1 form the right, f(x) should be taking value 5 i.e.,
f(x)x→1=5
Hence, the left hand limit of f(x) and right and limit of f(x are both equal to 5.
Thus, limx→1f(x)=limx→1f(x)=limx→1f(x)=5
This can be explained with the help of graph also
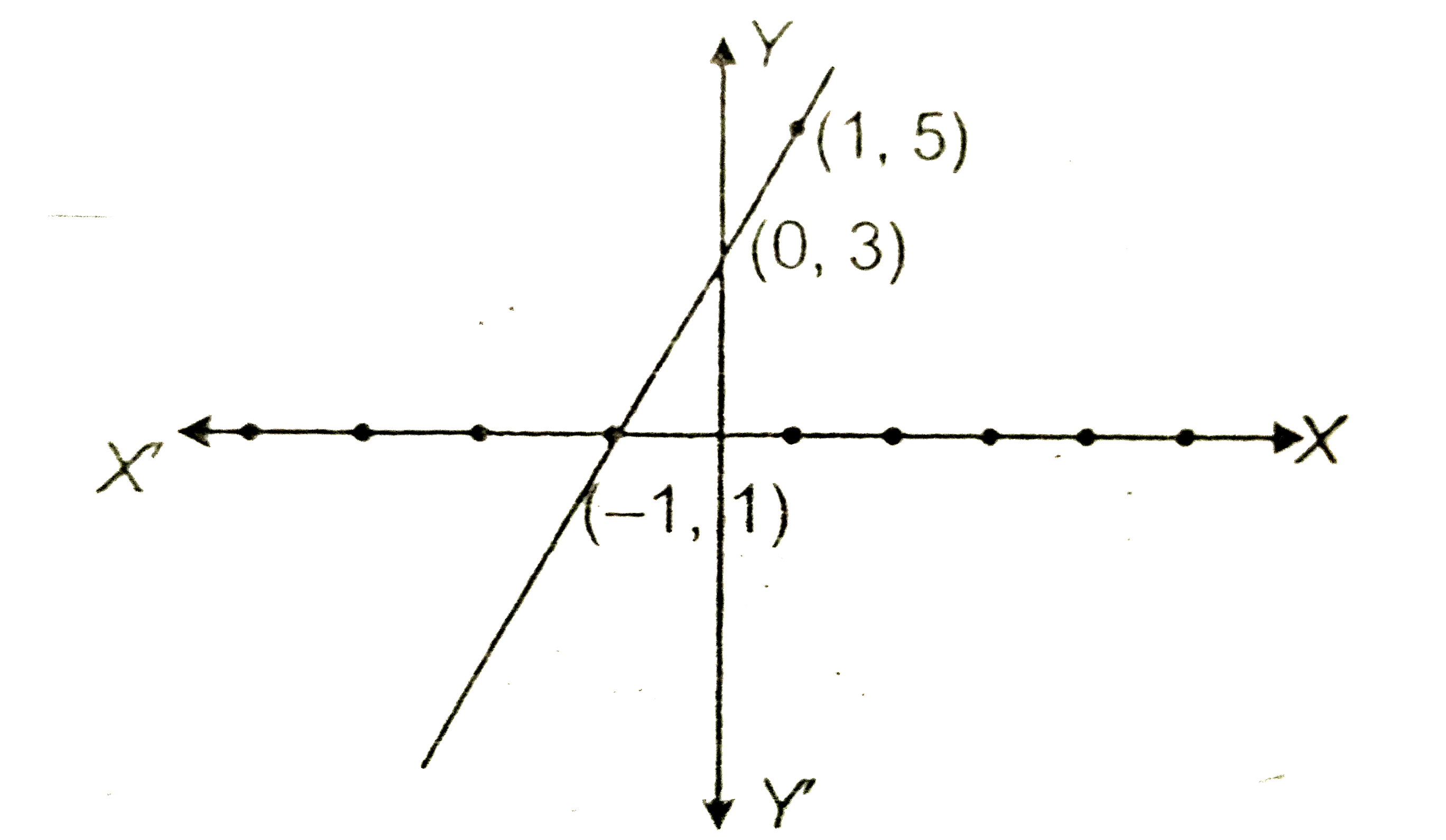
|
Topper's Solved these Questions
- LIMITS AND DERIVATIVES BOOK - AAKASH INSTITUTECHAPTER - LIMITS AND DERIVATIVES EXERCISE - Example
41 Videos
- LIMITS AND DERIVATIVES BOOK - AAKASH INSTITUTECHAPTER - LIMITS AND DERIVATIVES EXERCISE - Try yourself
64 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBOOK - AAKASH INSTITUTECHAPTER - INVERSE TRIGONOMETRIC FUNCTIONSEXERCISE - ASSIGNMENT (SECTION - J)(ANKASH CHALLENGERS QUESTIONS)
4 Videos
- MATHEMATICAL REASONINGBOOK - AAKASH INSTITUTECHAPTER - MATHEMATICAL REASONINGEXERCISE - Assignment (SECTION-D) (Assertion-Reason Type Questions)
15 Videos
Similar Questions
Knowledge Check
Consider the function f(x)=∣∣x3+1∣∣. Then,
Adomain of f x∈RBrange of f is R+Cf has no inverseDf is continuous and differentiable for every x∈RConsider the function f(x)=cosx2 then
Af is of period 2πBf is of period √2tCf is not periodicDf is of period π
Consider the constant function f(x) = 2. To find the limit of f(x) as x→3.
View SolutionConsider the function f(x) =|x−1|−2|x+2|+|x+3|

View SolutionFor the function f(x)=2. Find limx→1f(x)
View SolutionConsider the function f(x)=1x2 for x>0. To find limx→0f(x).
View SolutionConsider the function f(x)={2x+3,x≤1−x2+6,x>1
View SolutionConsider the function f(x)={2x+3,x≤1and−x2+6,x>1 Then draw the graph of the function y=f(x),y=f(|x|),y=|f(x)|,andy=|f(x)|.
View Solution
