For the function f(x)=2. Find limx→1f(x)
Video Solution
Text Solution
f(X) = 2, being a constant function takes the same value i.e., 2 in every case i.e., if we take the value of f(x) when x approaches 1 either from left or right, it will be equal to 2.
limx→1f(x)=limx→1+f(x)=limx→1f(x)=2
Graph of the function f(x) = 2 is the line parallel to x-axis and is shown below:
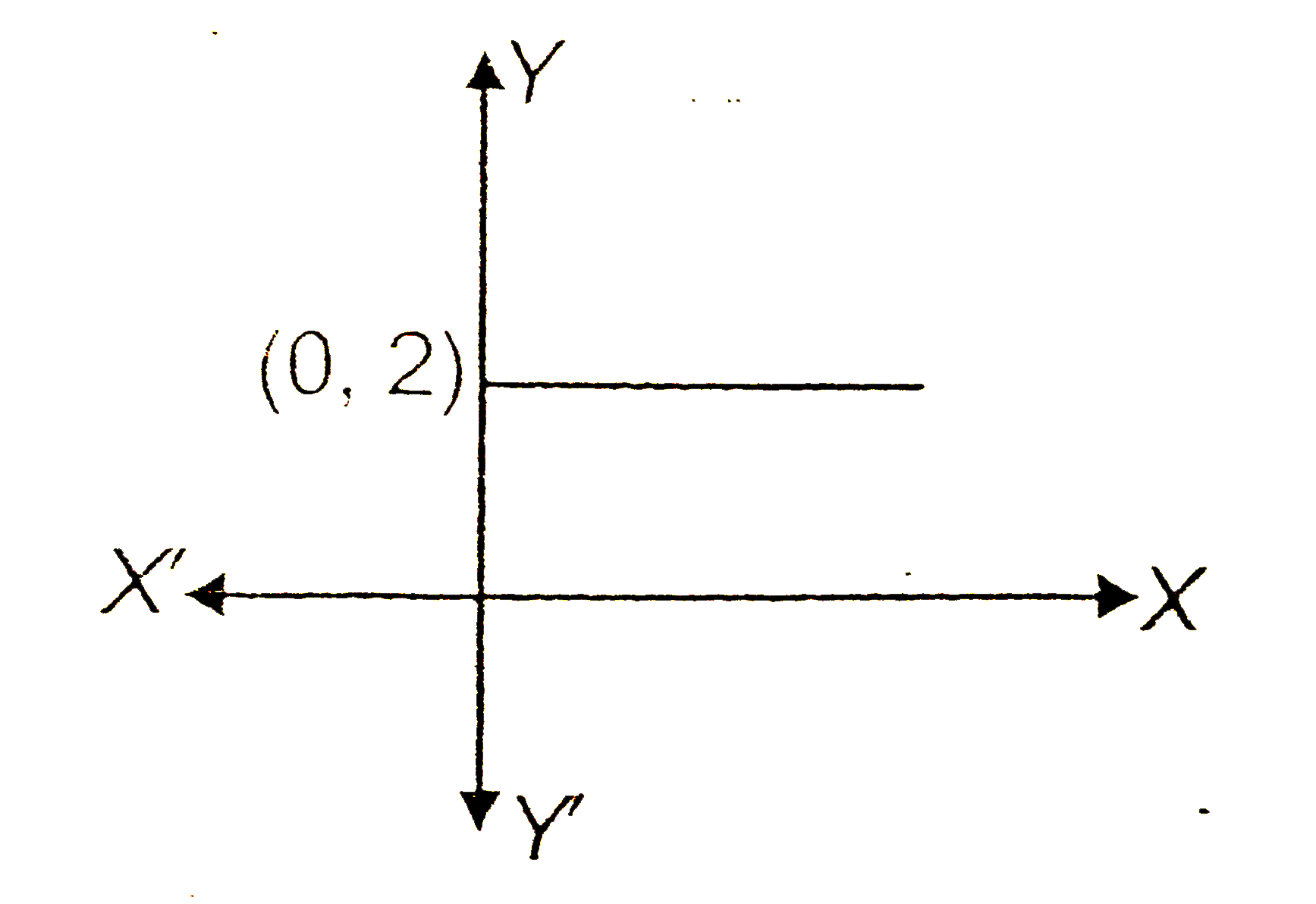
So, from graph also it is clear that the required limit is 2.
|
Topper's Solved these Questions
- LIMITS AND DERIVATIVES Book:AAKASH INSTITUTEChapter:LIMITS AND DERIVATIVES Exercise:Try yourself
Explore 64 Videos
- LIMITS AND DERIVATIVES Book:AAKASH INSTITUTEChapter:LIMITS AND DERIVATIVES Exercise:SECTION - A
Explore 50 Videos
- LIMITS AND DERIVATIVES Book:AAKASH INSTITUTEChapter:LIMITS AND DERIVATIVES Exercise:Section - j
Explore 3 Videos
- INVERSE TRIGONOMETRIC FUNCTIONSBook:AAKASH INSTITUTEChapter:INVERSE TRIGONOMETRIC FUNCTIONSExercise:ASSIGNMENT (SECTION - J)(ANKASH CHALLENGERS QUESTIONS)
Explore 4 Videos
- MATHEMATICAL REASONINGBook:AAKASH INSTITUTEChapter:MATHEMATICAL REASONINGExercise:Assignment (SECTION-D) (Assertion-Reason Type Questions)
Explore 15 Videos
Similar Questions
Knowledge Check
If the function f(x) satisfies limx→1f(x)−2x2−1=π, then limx→1f(x) is equal to
A1B2C0D3If a differentiable function f(x) satisfies
limx→−1f(x)+1x2−1=32
Then what is limx→−1f(x) equal to ?A−32B−1C0D1Let f:R→R be a positive increasing function with limx→∞f(3x)f(x)=1. Then limx→∞f(2x)f(x) =
A1B23C32D3
If the function f(x) satisfies (lim)x→1f(x)−2x2−1=π,evaluate (lim)x→1f(x)
View SolutionConsider the function f(x)={2x≤02x>0 Find limx→2
View SolutionConsider the function f(x)=1x2 for x>0. To find limx→0f(x).
View SolutionConsider the function :
f(x)={x+2,x≠10,x=1.
To find limx→1f(x).
View SolutionFind limx→0f(x) and limx→1f(x), where
f(x)={2x+3,x≤03(x+1),x>0
View Solution