For the function $f (x) = 4 x$ . Find $lim x \to 2 f (x)$

Video Solution

Text Solution

Verified by Experts

Let us compute the value of function for values of x very near to 2.
Firstly, we consider values of x approaching 2 from the left i.e., $x < 2$
$\begin{matrix} x & 1.5 & 1.6 & 1.7 & 1.8 & .1 .9 & 1.99 & 1.999 f (x) = 4 x & 6 & 6.4 & 6.8 & 7.2 & 7.6 & 7.96 & 7.996 \end{matrix}$
Now, we consider values of x approaching 2 from the right i.e., $x > 2$
$(x, 2.3, 2.2, 2.1, 2.05, 2.03, 2.01, 2.001), (f (x) = 4 x, 9.2, 8.8, 8.4, 8.2, 8.12, 8.04 .8 .004)$
Now from both the tables we observe that x approaches 2 either from left or right, the value of f(x) seems to approach 8.
So, $lim x \to 2 f (x) = lim x \to 2^{+} f (x) = lim x \to 2 f (x) = 8$
This can be shown by the graph also

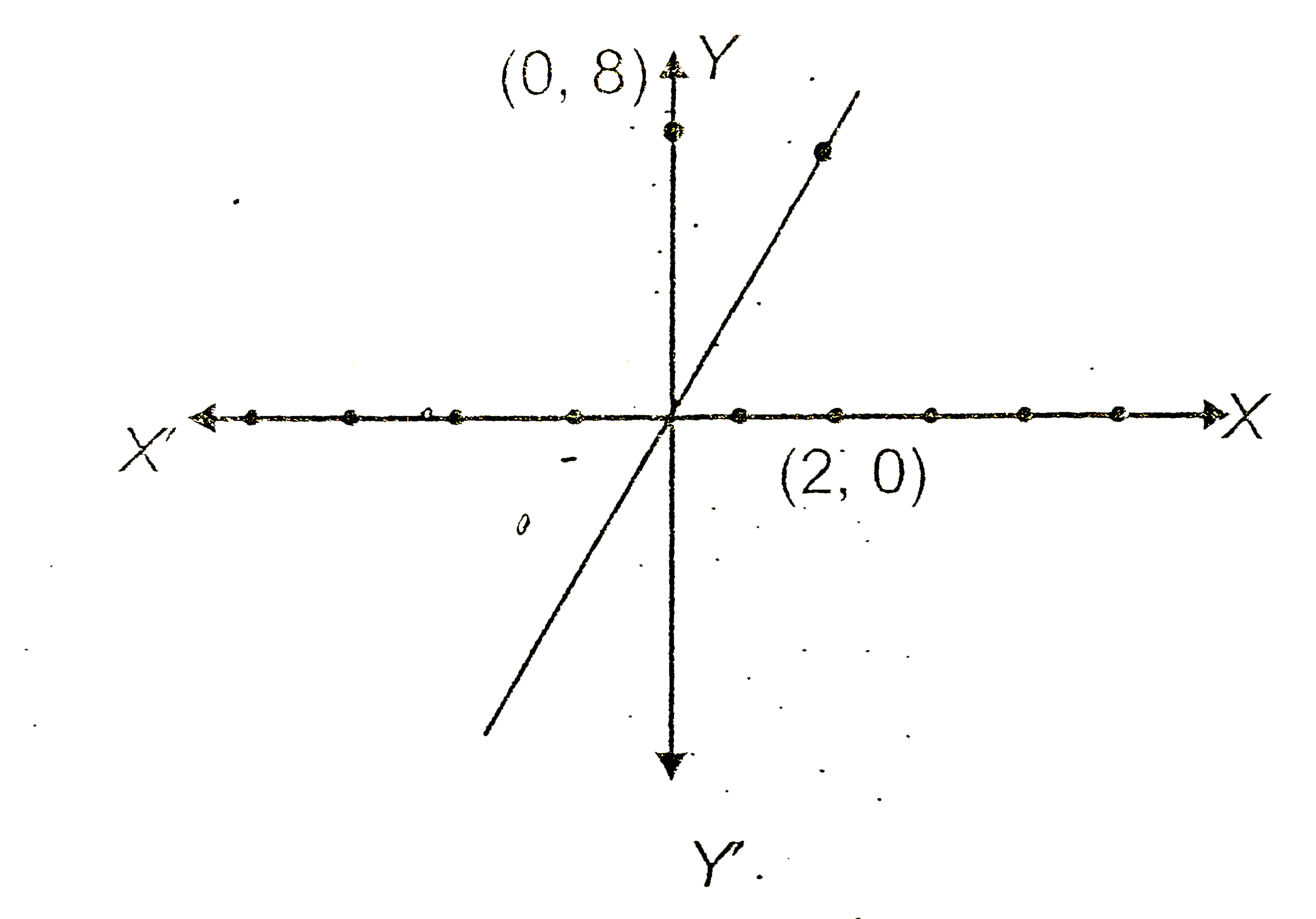
Here, again the value of the function at x = 2 coincide with the limit at x = 2

Updated on:21/07/2023

If the function f(x) satisfies $lim x \to 1 \frac{f (x) - 2}{x^{2} - 1} = π$ , then $lim x \to 1 f (x)$ is equal to
A1
B2
C0
D3
If a differentiable function f(x) satisfies
$lim x \to - 1 \frac{f (x) + 1}{x^{2} - 1} = \frac{3}{2}$
Then what is $lim x \to - 1 f (x)$ equal to ?
A $- \frac{3}{2}$
B $- 1$
C0
D1
Let $f : R \to [0, \infty)$ be such that $lim x \to 3$ f(x) exists and $lim x \to 3 \frac{{(f (x))}^{2} - 4}{\sqrt{| x - 3 |}} = 0$ . Then $lim x \to 3 f (x)$ equals
A0
B1
C2
D3