In an university, out of 120 students, 15 opted mathematics only, 16 opted statistics only, 9 opted for physics only and 45 opted physics and mathematics, 30 opted physics and statistics, 8 opted mathematics and statistics and 80 opted physics. By drawin Venn diagram, find the number of students who
(i) opted mathematics
(ii) opted statistics
(iii) did not opted any of the above three subjects.
To solve the problem, we will use a Venn diagram to represent the relationships between the students who opted for Mathematics, Statistics, and Physics. We will denote the sets as follows:
- Let M be the set of students who opted for Mathematics.
- Let S be the set of students who opted for Statistics.
- Let P be the set of students who opted for Physics.
Given Data:
- Total number of students = 120
- Students opting for Mathematics only = 15
- Students opting for Statistics only = 16
- Students opting for Physics only = 9
- Students opting for both Physics and Mathematics = 45
- Students opting for both Physics and Statistics = 30
- Students opting for both Mathematics and Statistics = 8
- Total students opting for Physics = 80
Step 1: Set Up the Venn Diagram
We will denote the number of students in the intersections of the sets as follows:
- Let x be the number of students who opted for all three subjects (Mathematics, Statistics, and Physics).
Step 2: Calculate the Number of Students in Each Section
From the given data, we can write the following equations based on the Venn diagram:
1. Students opting for Mathematics and Physics only (not Statistics) = 45−x
2. Students opting for Physics and Statistics only (not Mathematics) = 30−x
3. Students opting for Mathematics and Statistics only (not Physics) = 8−x
Step 3: Calculate Total Students in Physics
The total number of students who opted for Physics can be expressed as:
Total in Physics=(Physics only)+(Mathematics and Physics only)+(Statistics and Physics only)+(All three)
Substituting the values we have:
80=9+(45−x)+(30−x)+x
Simplifying this equation:
80=9+45+30−x
80=84−x
x=84−80=4
Step 4: Substitute x Back to Find Other Values
Now that we have x=4, we can find the number of students in each section:
- Students opting for Mathematics and Physics only = 45−4=41
- Students opting for Physics and Statistics only = 30−4=26
- Students opting for Mathematics and Statistics only = 8−4=4
Step 5: Calculate Total Students in Each Subject
Now we can calculate the total number of students who opted for each subject:
1. Total students opting for Mathematics:
|M|=(Mathematics only)+(Mathematics and Physics only)+(Mathematics and Statistics only)+(All three)
|M|=15+41+4+4=64
2. Total students opting for Statistics:
|S|=(Statistics only)+(Statistics and Physics only)+(Mathematics and Statistics only)+(All three)
|S|=16+26+4+4=50
Step 6: Calculate Students Not Opting for Any Subject
To find the number of students who did not opt for any of the subjects, we first calculate the total number of students who opted for at least one subject:
Total students opting for at least one subject=|M|+|S|+|P|−(Students in all three)
Where P is the total number of students who opted for Physics:
|P|=9+41+26+4=80
Thus:
Total=64+50+80−4=190−4=186
However, this is incorrect because we need to consider overlaps correctly. The correct calculation should be:
Total=15+16+9+41+26+4+4=115
Now, the number of students who did not opt for any subject:
Students not opting for any subject=120−115=5
Final Answers:
(i) Number of students who opted for Mathematics = 64
(ii) Number of students who opted for Statistics = 50
(iii) Number of students who did not opt for any of the above three subjects = 5
- Let S be the set of students who opted for Statistics.
- Let P be the set of students who opted for Physics.
- Total number of students = 120
- Students opting for Mathematics only = 15
- Students opting for Statistics only = 16
- Students opting for Physics only = 9
- Students opting for both Physics and Mathematics = 45
- Students opting for both Physics and Statistics = 30
- Students opting for both Mathematics and Statistics = 8
- Total students opting for Physics = 80
We will denote the number of students in the intersections of the sets as follows:
- Let x be the number of students who opted for all three subjects (Mathematics, Statistics, and Physics).
From the given data, we can write the following equations based on the Venn diagram:
2. Students opting for Physics and Statistics only (not Mathematics) = 30−x
3. Students opting for Mathematics and Statistics only (not Physics) = 8−x
The total number of students who opted for Physics can be expressed as:
Total in Physics=(Physics only)+(Mathematics and Physics only)+(Statistics and Physics only)+(All three)
Substituting the values we have:
80=9+(45−x)+(30−x)+x
Simplifying this equation:
80=9+45+30−x
80=84−x
x=84−80=4
Now that we have x=4, we can find the number of students in each section:
- Students opting for Mathematics and Physics only = 45−4=41
- Students opting for Physics and Statistics only = 30−4=26
- Students opting for Mathematics and Statistics only = 8−4=4
Now we can calculate the total number of students who opted for each subject:
1. Total students opting for Mathematics:
|M|=(Mathematics only)+(Mathematics and Physics only)+(Mathematics and Statistics only)+(All three)
|M|=15+41+4+4=64
|S|=(Statistics only)+(Statistics and Physics only)+(Mathematics and Statistics only)+(All three)
|S|=16+26+4+4=50
To find the number of students who did not opt for any of the subjects, we first calculate the total number of students who opted for at least one subject:
Total students opting for at least one subject=|M|+|S|+|P|−(Students in all three)
Where P is the total number of students who opted for Physics:
|P|=9+41+26+4=80
Thus:
Total=64+50+80−4=190−4=186
However, this is incorrect because we need to consider overlaps correctly. The correct calculation should be:
Total=15+16+9+41+26+4+4=115
Now, the number of students who did not opt for any subject:
Students not opting for any subject=120−115=5
(i) Number of students who opted for Mathematics = 64
(ii) Number of students who opted for Statistics = 50
(iii) Number of students who did not opt for any of the above three subjects = 5
Topper's Solved these Questions
- SETS BOOK - AAKASH INSTITUTECHAPTER - SETS EXERCISE - Try yourself
50 Videos
- SETS BOOK - AAKASH INSTITUTECHAPTER - SETS EXERCISE - SECTION-A(Objective Type Questions (One option is correct))
41 Videos
- SETS BOOK - AAKASH INSTITUTECHAPTER - SETS EXERCISE - SECTION-I(Aakash Challengers Questions)
5 Videos
- SEQUENCES AND SERIES BOOK - AAKASH INSTITUTECHAPTER - SEQUENCES AND SERIES EXERCISE - Assignment (SECTION - J) Aakash Challengers
12 Videos
- STATISTICSBOOK - AAKASH INSTITUTECHAPTER - STATISTICSEXERCISE - Section-C Assertion-Reason
15 Videos
Similar Questions
In an university, out of 100 students, 16 opted mathematics only, 24 opted physics only, 30 opted for chemisty only and 8 opted chemistry and mathematics, 14 opted physics and chemistry, 4 opted mathematics and physics, and 48 opted chemistry. By drawing Venn diagram, find the number of students who
View SolutionIn a group of 200 students, 20 played cricket only, 36 plated tennis only, 40 played, hockey only, 8 played cricket and tennis, 20 played cricket and hockey, 28 played hockey and tennis and 80 played hockey. By Venn diagram, find the number of students who (i) played cricket (ii) played tennis (iii) did not played any of the above three games.
View Solution
Knowledge Check
In a survey of 200 students of a school, it was found that 120 study Mathematics, 90 study Physics and 70 study Chemistry , 40 study Mathematics and Physics, 30 study Physics and Chemistry, 50 study Chemistry and Mathematics and 20 none of these subjects. Find the number of students who study all the three subjects.
A30B20C10DNone of TheseIn a class of 80 students who have appeared in a test in Mathematics and a test in Physics, 55 students have passed in Mathematics and 57 students have passed in Physics, then the number of students who have passed in Physics only is
A52B32C38D65In a survey of 25 students, it was found that 15 had taken Mathematics, 12 had taken Physics and 11 had taken Chemistry, 5 had taken Mathematics and Chemistry, 9 had taken Mathematics and Physics, 4 had taken Physics and Chemistry and 3 had taken all the three subjects.
The number of students who had taken only physics is :A2B3C5D6
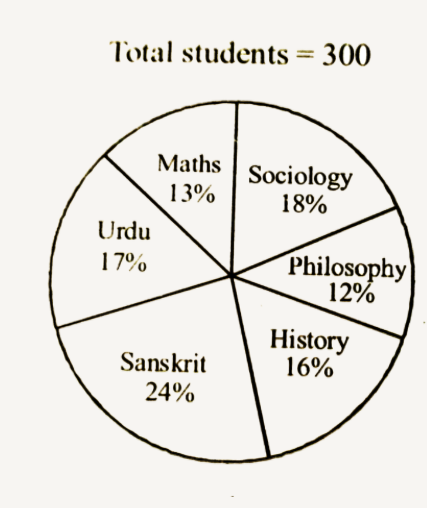
Find the central angle of total number of students who opted for History ?
View Solution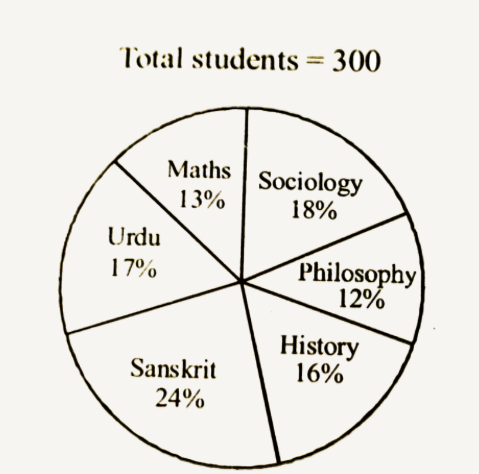
Find the average number of students who opted for Maths, Sociology and Sanskrit together ?
View SolutionIn a survey of 25 students, it was found that 15 had taken mathematics, 12 had taken physics, and 11 had taken chemistry, 5 had taken mathematics and chemistry, 9 had taken mathematics and physics, 4 had taken physics and chemistry, and 3 had taken all the three subjects. Find the number of students who had taken
(a) Only mathematics.
(b) Mathematics and physics, but not chemistry.
(C) At least one of the three subjects.
(d) Only one of the three subjects.
View SolutionIn a survey of 200 students of a school, it was found that 120 study Mathematics, 90 study Physics and 70 study Chemistry , 40 study Mathematics and Physics, 30 study Physics and Chemistry, 50 study Chemistry and Mathematics and 20 none of these subjects. Find the number of students who study all the three subjects.
View SolutionIn a class of a certain school, 50 stduents offered mathematics , 42 offered biology and 24 offered both the subjets. Find the number of students offfereing.
(i) mathematics only,
(ii) biology only,
(iii) any of the two subjects.
View Solution
