A liquid of refractive index 1.5 is poured into a cyclindrical jar of radius 20 cm upto a height of 20 cm. A small bulb at the centre of bottom glowing. Find area of the liquid surface through which the light of the bulb passes into air.
To solve the problem, we will follow these steps:
Step 1: Understand the Geometry
We have a cylindrical jar with a radius r=20 cm and a height h=20 cm filled with a liquid of refractive index μ=1.5. A bulb is located at the center of the bottom of the jar.
Step 2: Identify the Relevant Formula
The area of the liquid surface through which light passes into the air can be calculated using the formula derived from the geometry of the situation:
r=h√μ2−1
where r is the radius of the circular area through which light escapes, h is the height of the liquid, and μ is the refractive index.
Step 3: Calculate the Radius r
Substituting the known values into the formula:
- h=20 cm = 0.20 m
- μ=1.5
We calculate r:
r=0.20√(1.5)2−1=0.20√2.25−1=0.20√1.25=0.201.118≈0.179 m
Step 4: Calculate the Area
The area A of the circular surface through which light passes into the air is given by:
A=πr2
Substituting the value of r:
A=π(0.179)2≈3.14×0.032036≈0.1005 m2
Final Answer
The area of the liquid surface through which the light of the bulb passes into the air is approximately 0.1005 m2.
---
We have a cylindrical jar with a radius r=20 cm and a height h=20 cm filled with a liquid of refractive index μ=1.5. A bulb is located at the center of the bottom of the jar.
The area of the liquid surface through which light passes into the air can be calculated using the formula derived from the geometry of the situation:
r=h√μ2−1
where r is the radius of the circular area through which light escapes, h is the height of the liquid, and μ is the refractive index.
Substituting the known values into the formula:
- h=20 cm = 0.20 m
- μ=1.5
r=0.20√(1.5)2−1=0.20√2.25−1=0.20√1.25=0.201.118≈0.179 m
The area A of the circular surface through which light passes into the air is given by:
A=πr2
Substituting the value of r:
A=π(0.179)2≈3.14×0.032036≈0.1005 m2
The area of the liquid surface through which the light of the bulb passes into the air is approximately 0.1005 m2.
Similar Questions
Glycerine (refractive index 1.4) is poured into a large jar of radiys 0.2m to a depth of 0.1m. There is a small light source at the centre of the bottom of the jar. Find the area of the surface of glycerine through which the light passes.
View SolutionA point light source is attached to the base of an empty trough. A transparent liquid of refractive index 1.35 is filled in the trough to a height of 50 cm. Calculate the total area on the surface of the liquid through which light will emerge.
View Solution
Knowledge Check
A liquid of refractive index 1.6 is contained in the cavity of a glass specimen of refractive index 1.5 as shown in figure. If each of the curve surface has a radius of curvature of 0.20m, the arrangement obehaves as a
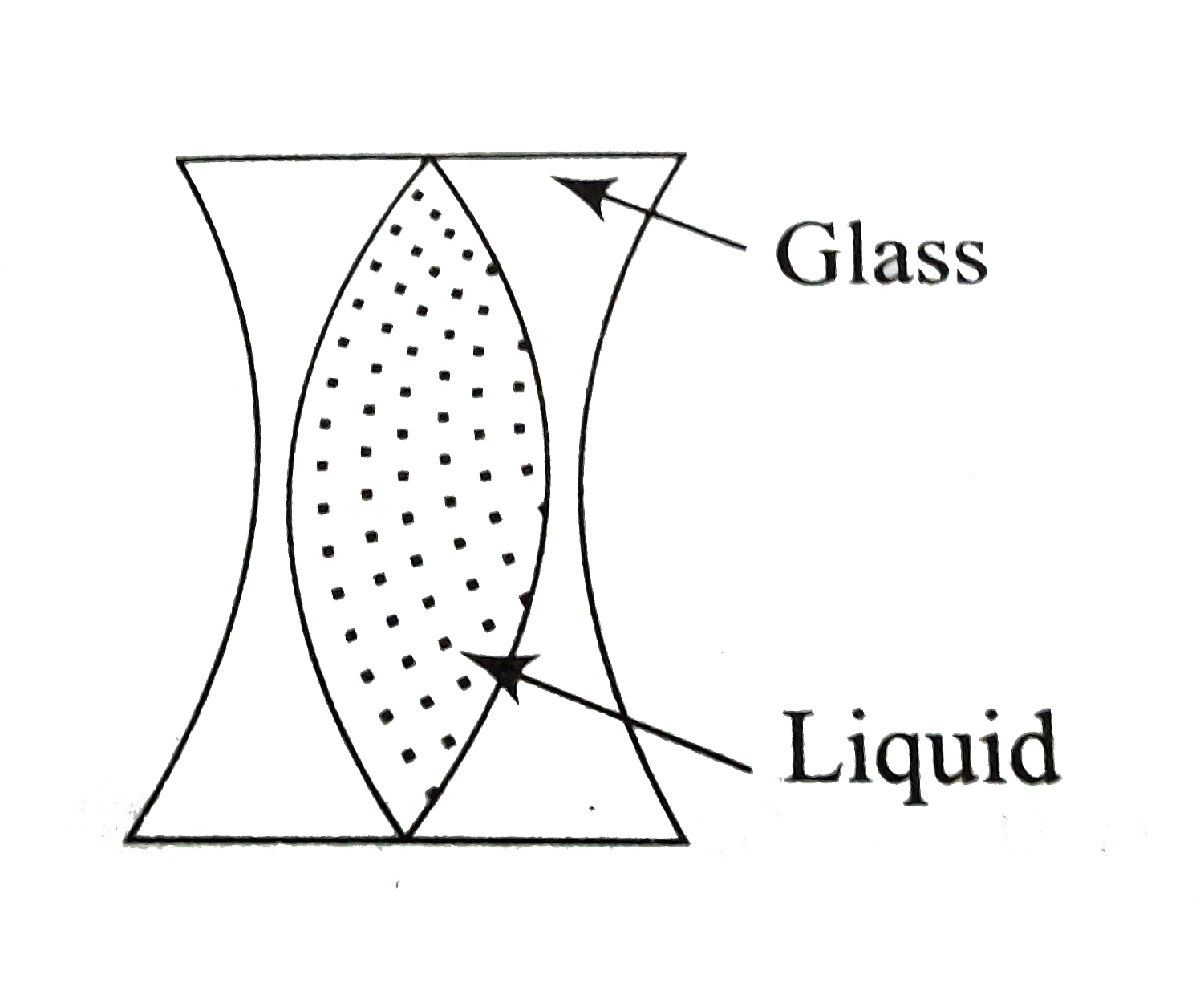 Aconverging lens of focal length 0.25mBdiverging lens of focal length 0.25mCdiverging lens of focal length 0.17mDconverging lens of focal length 0.72m
Aconverging lens of focal length 0.25mBdiverging lens of focal length 0.25mCdiverging lens of focal length 0.17mDconverging lens of focal length 0.72m
A cylindrical jar of radius 10 cm is filled with water upto a height of 15cm. 14 sphercal balls of radius 3 cm each are immersed in the jar. Find the new level to which water is filled in the jar.
View SolutionA point source of monochromatic light 'S' is kept at the centre of the bottom of a cylinder of radius 15.0cm. The cylinder contains water (refractive index 4//3) to a height of 7.0cm. Draw the ray diagram and calculate the area of water surface through which the light emerges in air.
View Solution(a) Define the term 'critical angle' for a pair of media
(b) A point source of monochromatic light S is kept at the centre of the bottom of a cylinder of radius 15.0 cm. The cylinder contains water (refractive index =43 to a height of 7.0 cm. Draw the ray diagram and calculate the area of water surface through which the light emerges in air.
View SolutionA point source of monochromatic light 'S' is kept at the centre of the bottom of a cylinder of radius 15.0cm. The cylinder contains water (refractive index 4//3) to a height of 7.0cm. Draw the ray diagram and calculate the area of water surface through which the light emerges in air.
View SolutionA small illuminated bulb is at the bottom of a tank, containing a liquid of refractive index μ upto a height H. Find the expression for the diameter of an opaque disc, floating symmetrically on the liquid surface in order to cut- off the light from the bulb.
View SolutionA point source of light S is placed at the bottom of a vessel containing a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of disc lies vertically above the source O. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot be seen at all.
View SolutionA point source of light S is placed at the bottom of a vessel containg a liquid of refractive index 5/3. A person is viewing the source from above the surface. There is an opaque disc of radius 1cm floating on the surface. The centre of the disc lies vertically above the source S. The liquid from the vessel is gradually drained out through a tap. What is the maximum height of the liquid for which the source cannot at all be seen from above?
View Solution