Question

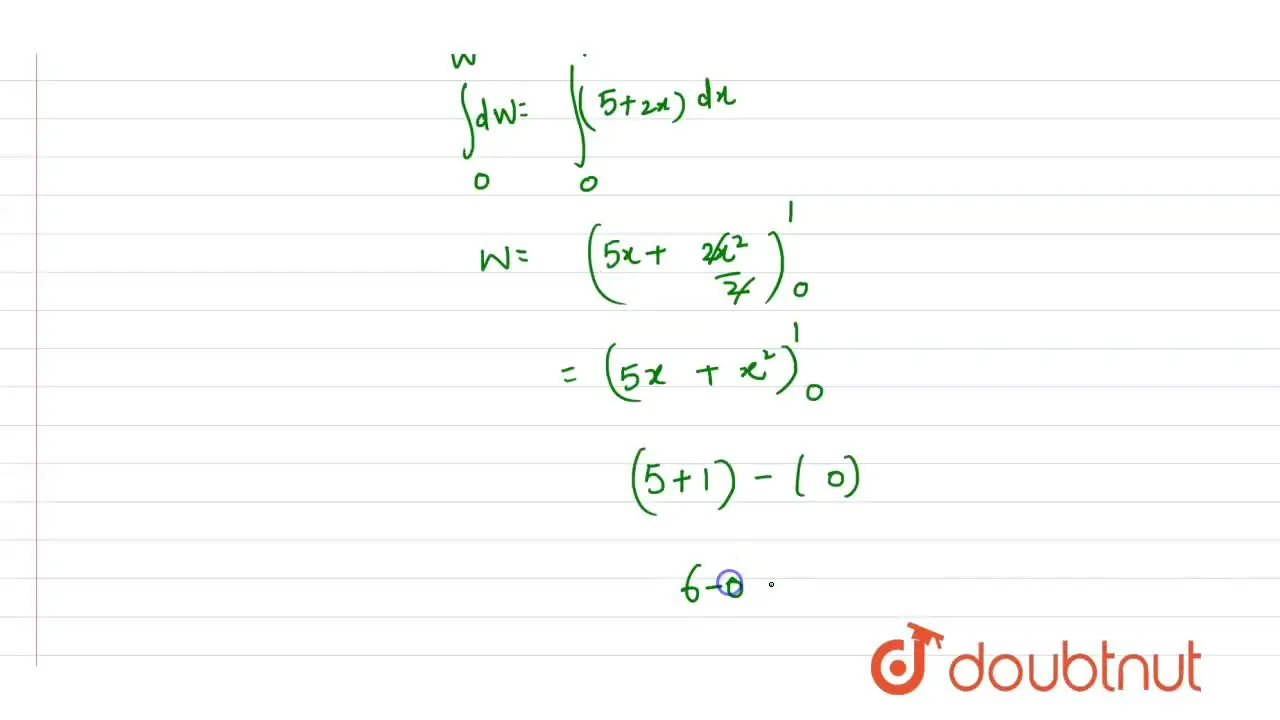
A force $F=(5+2x)$ acts on a particle in x direction where F is in newtown and x is meter. Find the work done by this force during displacement from $x=0$ to $x=1m.$

Explanation

To find the work done by the force $$F=(5+2x)$$ during the displacement from $$x=0$$ to $$x=1$$ meter, we can follow these steps:

Step 1: Understand the Work Done Formula
The work done \( W \) by a variable force can be calculated using the integral of the force over the displacement. The formula is:
\( W = \int_{x_1}^{x_2} F \, dx \)
where \( F \) is the force and \( x_1 \) and \( x_2 \) are the initial and final positions.

Step 2: Set Up the Integral
In this case, the force \( F \) is given as \( F = 5 + 2x \). We need to integrate this force from \( x = 0 \) to \( x = 1 \):
\( W = \int_{0}^{1} (5 + 2x) \, dx \)

Step 3: Calculate the Integral
Now we can calculate the integral:
\( W = \int_{0}^{1} (5 + 2x) \, dx = \int_{0}^{1} 5 \, dx + \int_{0}^{1} 2x \, dx \)
Calculating each part separately:
1. For \( \int_{0}^{1} 5 \, dx \):
\( = 5x \bigg|_{0}^{1} = 5(1) - 5(0) = 5 \)
2. For \( \int_{0}^{1} 2x \, dx \):
\( = x^2 \bigg|_{0}^{1} = (1)^2 - (0)^2 = 1 \)

Step 4: Combine the Results
Now, combine the results from both integrals:
\( W = 5 + 1 = 6 \, \text{Joules} \)

Final Answer
The work done by the force during the displacement from \( x = 0 \) to \( x = 1 \) meter is \( 6 \, \text{Joules} \).

---