A particle of mass m is released from point A as shown in the gigure. Would it loop the loop if H=4r? What is the force on the circular track when it is at point (a) B, (b) C?
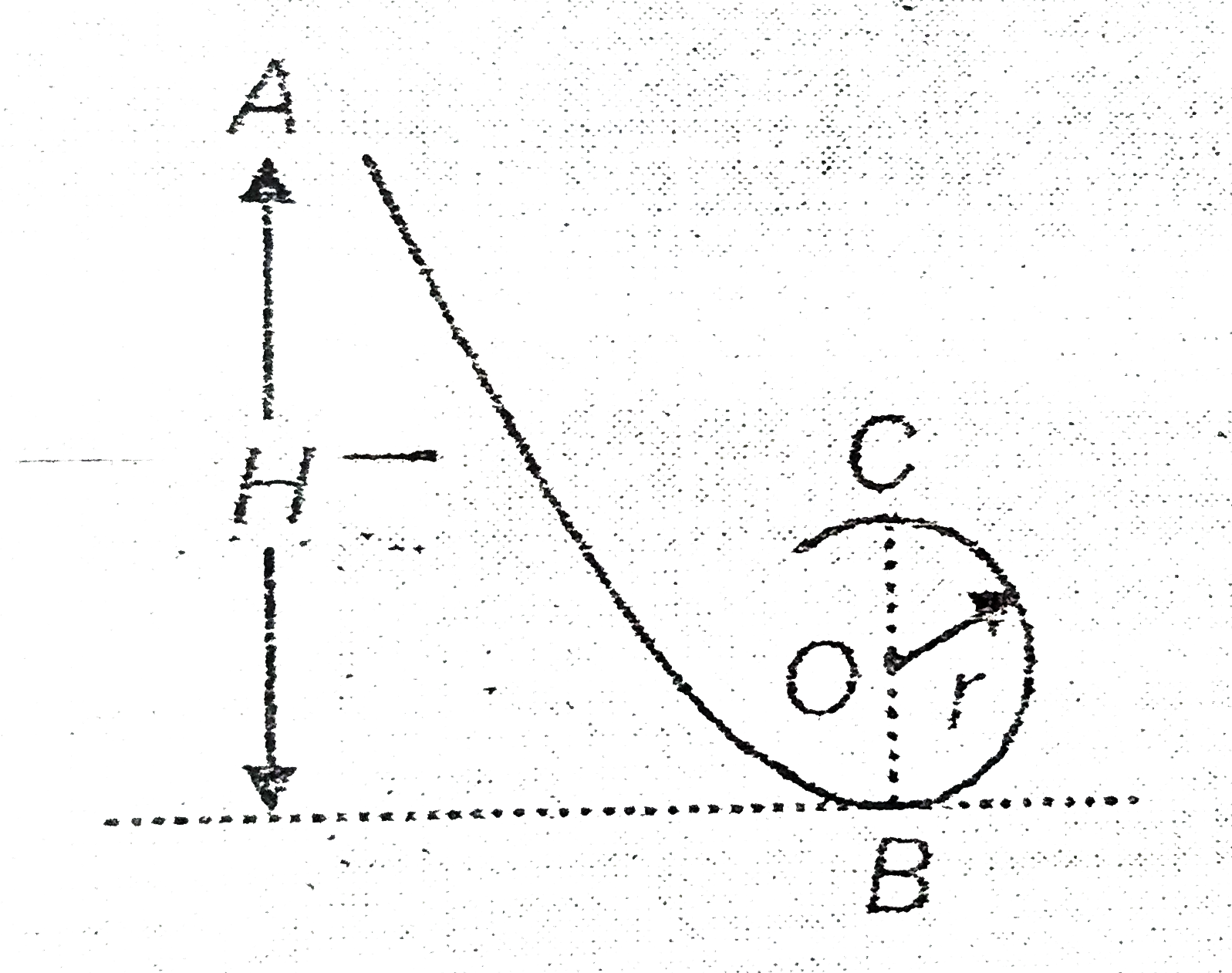
Let speed at the lowest point B ve vB
Total energy A= Total energy at B
0+mgH=12mv2B
vB=√2gH=√8gr
Let N1 be the force exerted by the track at B.
N1=mg+mv2BR
If vc is velocity at C.
v2C=v2B−4gr
N2 is force exerted by the track at C
N2=mv2cr−mg
Let speed at lowest point B be vB.
Total energy at A= Total energy at B
0+mgH=12mv2B+0
vB=√2gh=√2g×4r=√8gr>vmin(=√5gr)
So, the particle can loop the loop
Let N1 be the force exerted by the track at B.
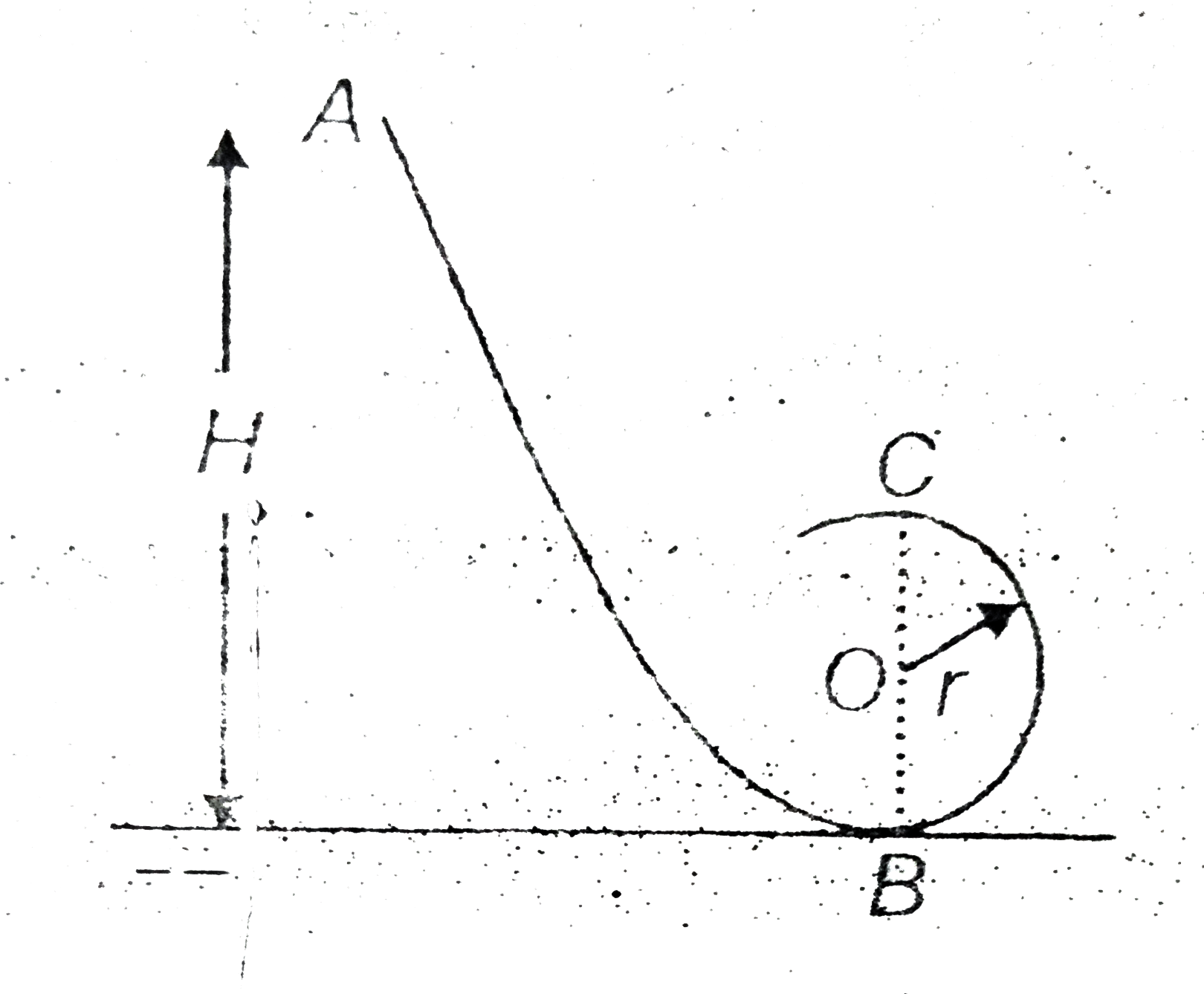
According to III law,
N1=mg+mv2Br
=mg+m×8grr=9mg
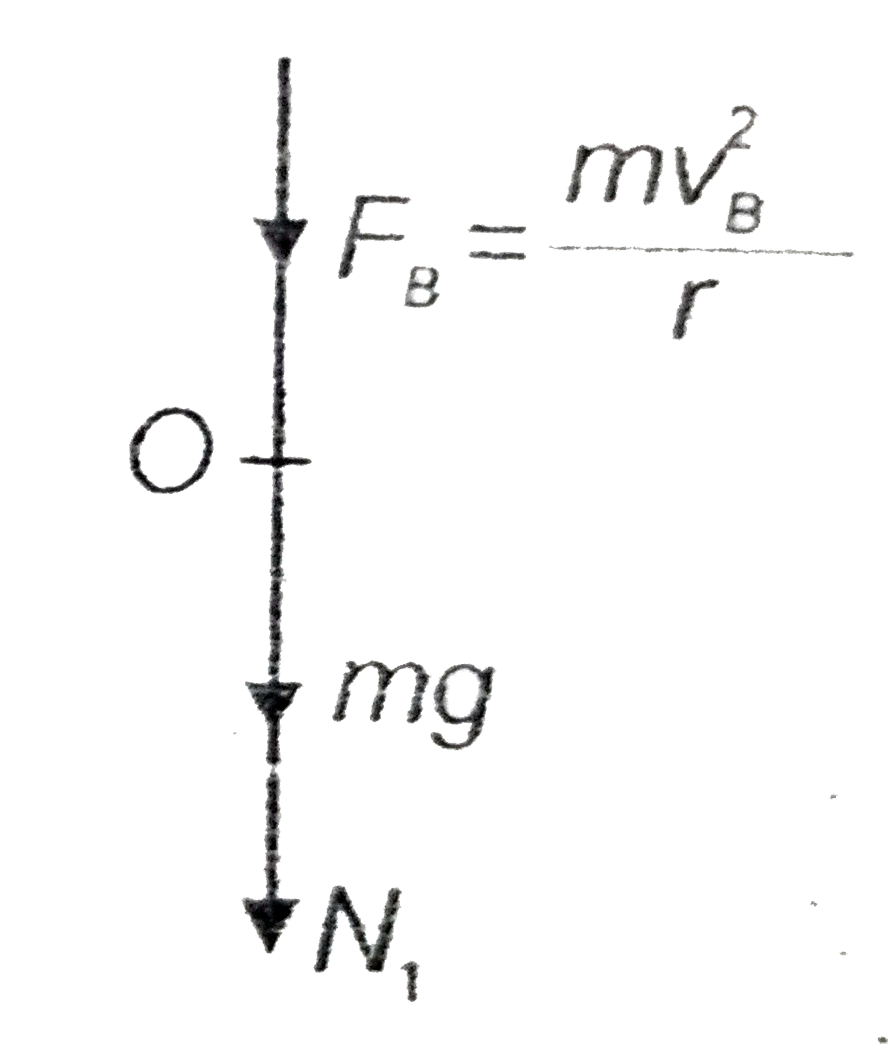
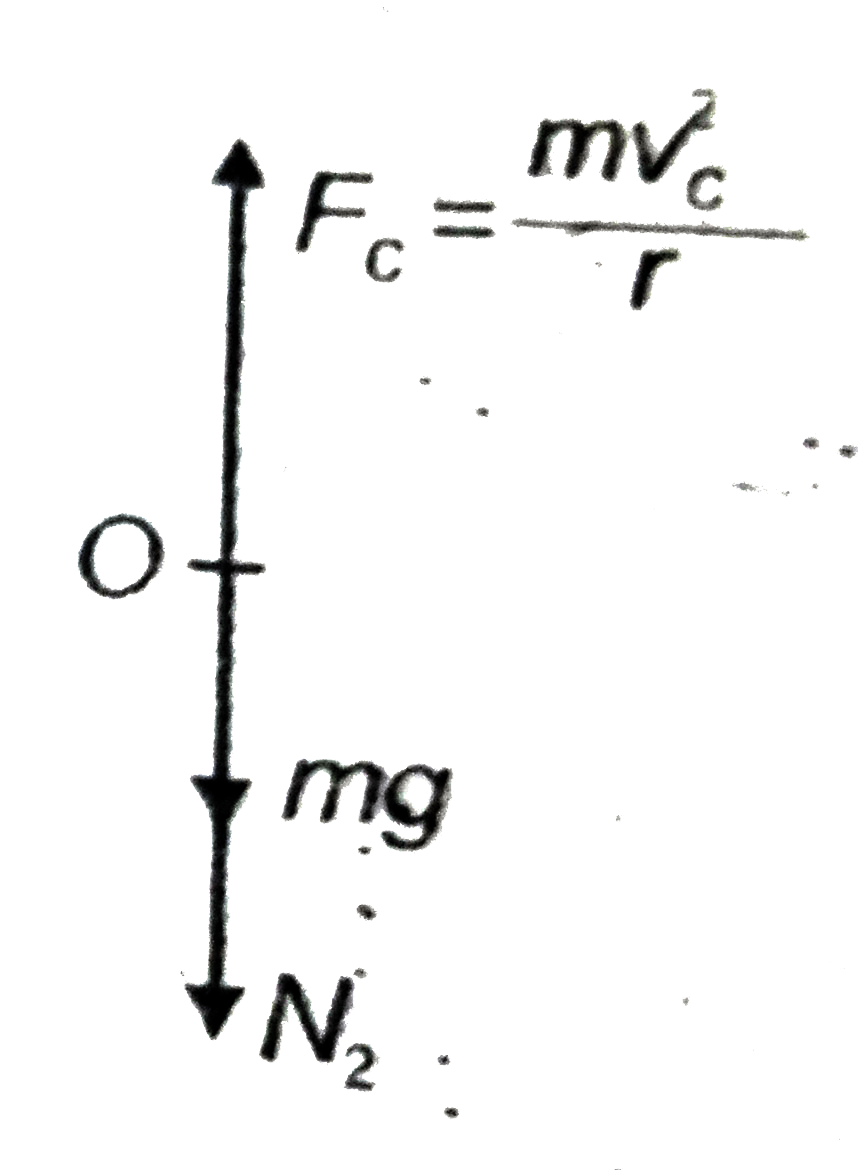
Force exerted by the particle on the track =9 mgh directed vertically downward At C,
Let the speed be vC
v2C=v2B−4gr
=8gr−4gr=4gr
Let N2 be the force exerted by the track at C
N2=mv2Cr−mg
=4mg−mg
=3mg
Force exerted by the particle on the track at C is 3 mg directed redially outward.
Topper's Solved these Questions
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-A (OBJECTIVE TYPE QUESTIONS (ONE OPTIONISCORRECT)
60 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - SECTION-B (SUBJECTIVE TYPE QUESTIONS) (ONE OPTIONS IS CORRECT)
44 Videos
- WORK, ENERGY AND POWERBOOK - AAKASH INSTITUTECHAPTER - WORK, ENERGY AND POWEREXERCISE - IILUSTRATION
2 Videos
- WAVESBOOK - AAKASH INSTITUTECHAPTER - WAVESEXERCISE - ASSIGNMENT ( SECTION-D ( Assertion - Reason Type Questions ))
12 Videos
Similar Questions
Shown a smooth looping the loop track A particle of mass m is released from oint A , as shown If H = 3 r , whould the particle loop the loop ? What is the force on the circular track when the particle is at point (i) B (ii) C ? .
View SolutionA particle of mass m=5.00kg is released from point A and it slides on the frictionless track shown in figure. Determine (a) the particle's speed at points B and C and (b) the net work done by the gravitational force as the particle moves from A to C.
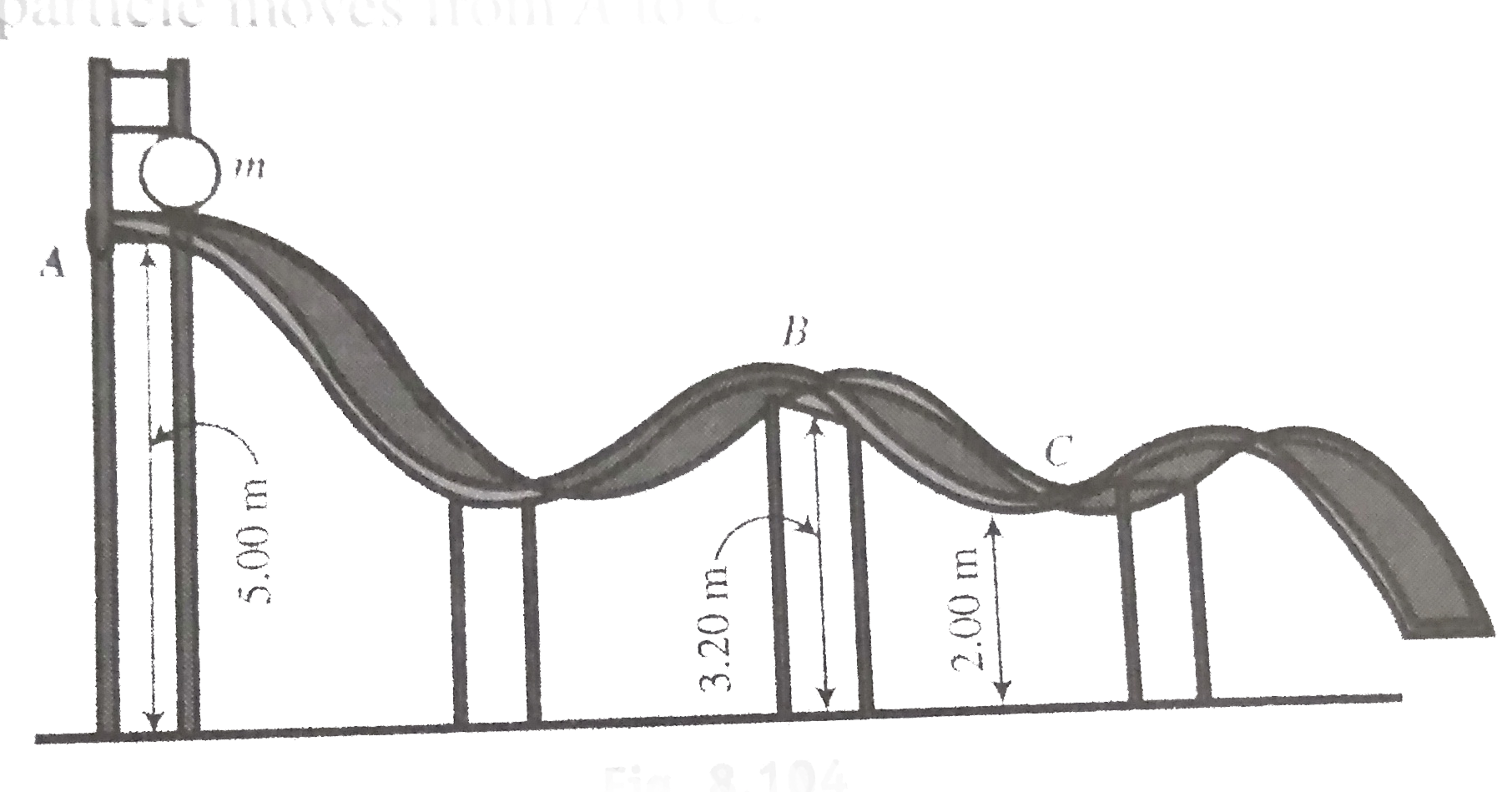
View Solution
Knowledge Check
A ball of mass m is released from A inside a smooth wedge of mass m as shown in figure. What is the speed of the wedge when the ball reaches point B?
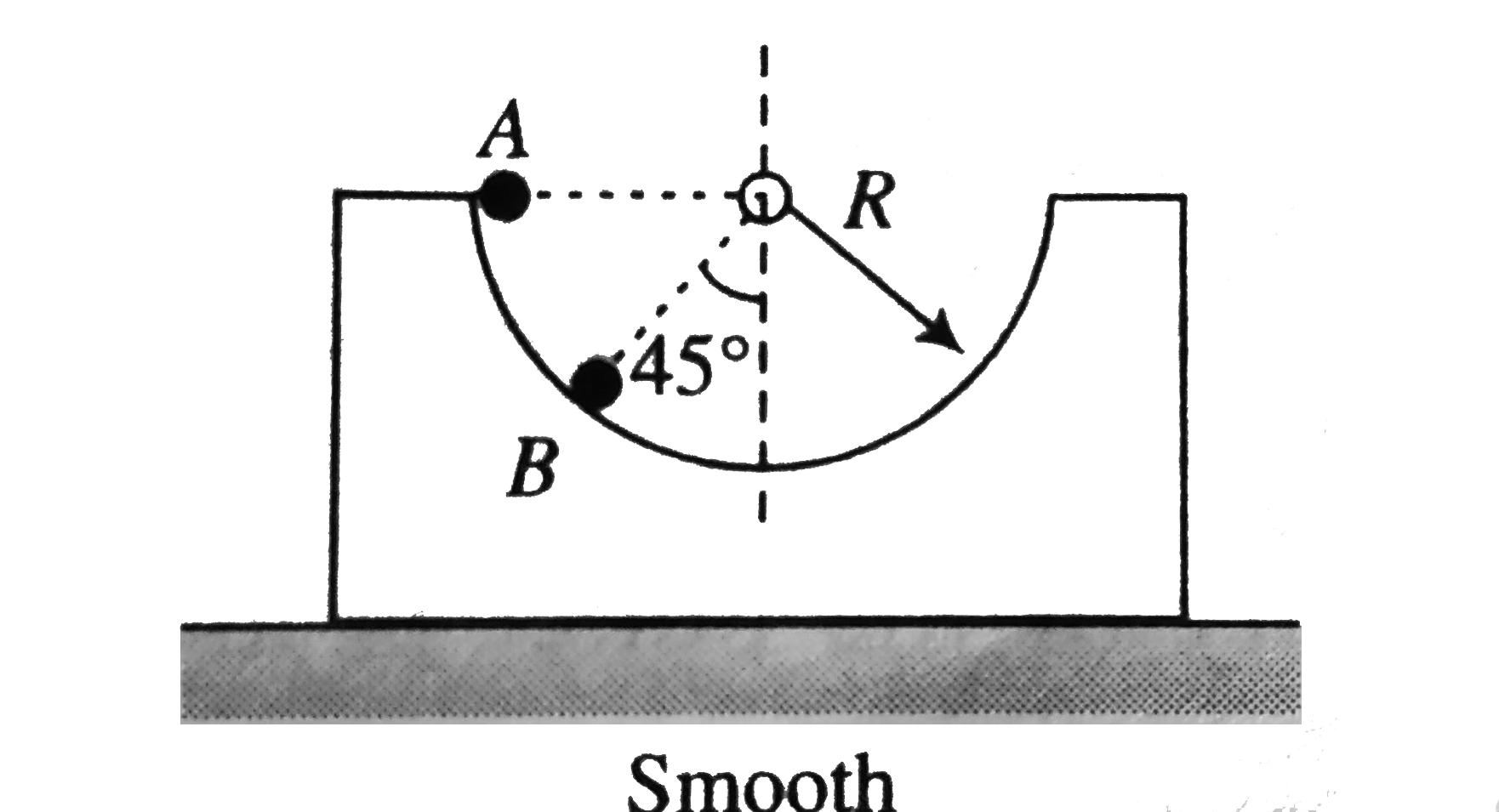 A(a) (gR3√2)1/2B(b) √2gRC(c) (5gR2√3)1/2D(d) √32gR
A(a) (gR3√2)1/2B(b) √2gRC(c) (5gR2√3)1/2D(d) √32gRFigure shows a circular frications trck of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along on the track. Then the coefficient of restitution e is.
 A0.2B0.5C1Dzero
A0.2B0.5C1DzeroA particle of mass m is released from a height H on a smooth curved surface which ends into a vertical loop of radius R, as shown. Choose the correct alernative(s) if H=2R.
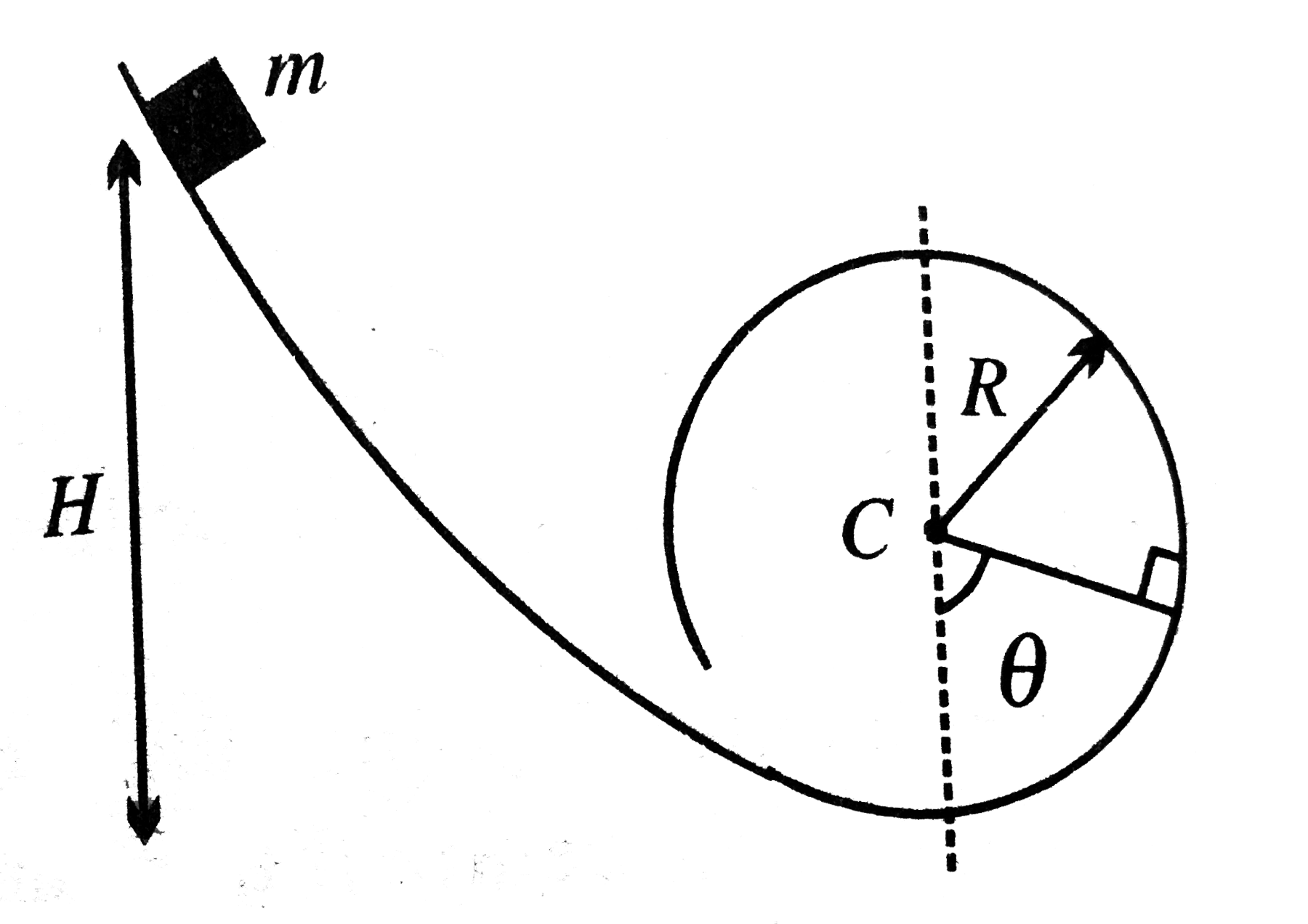 AThe particles reaches the top of the loop with zero velocityBThe particle cannot reach the top of the loop.CThe particle breaks off at a height h=R from the base of the loop.DThe particle breaks off at a height h from the base of the loop such that R<h<2R
AThe particles reaches the top of the loop with zero velocityBThe particle cannot reach the top of the loop.CThe particle breaks off at a height h=R from the base of the loop.DThe particle breaks off at a height h from the base of the loop such that R<h<2R
A circular metal loop is heated at point O as shown in figure.
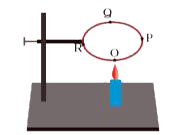
In which direction would heat flow in the loop ?
View SolutionWhen does a charged circular loop behave as a point charge.
View SolutionFigure shows a circular frictionless track of radius R, centred at point O. A particle of mass M is released from point A(OA=R/2). After collision with the track, the particle moves along the track.
(a) Find the coefficient of restitution e.
(b) What will be value of e if the velocity of the particle becomes horizontal just after collision ?
View SolutionA particle of mass 4kg moves between two points A and B on a smooth horizontal surface under the aciton of two forces such that when it is at a point P, the forces are 2→PAN and 2→PBN. If the particle is released from rest at A, find the time it takes to travel a quarter of the way A to B.
View SolutionA particle of mass 100 g is moving in a vertical circle of radius 2 m The particle is just looping the loop . What is the speed of the particle and tension in the string at the highest point of the circular path ? . (g=10ms−2) .
View Solution
