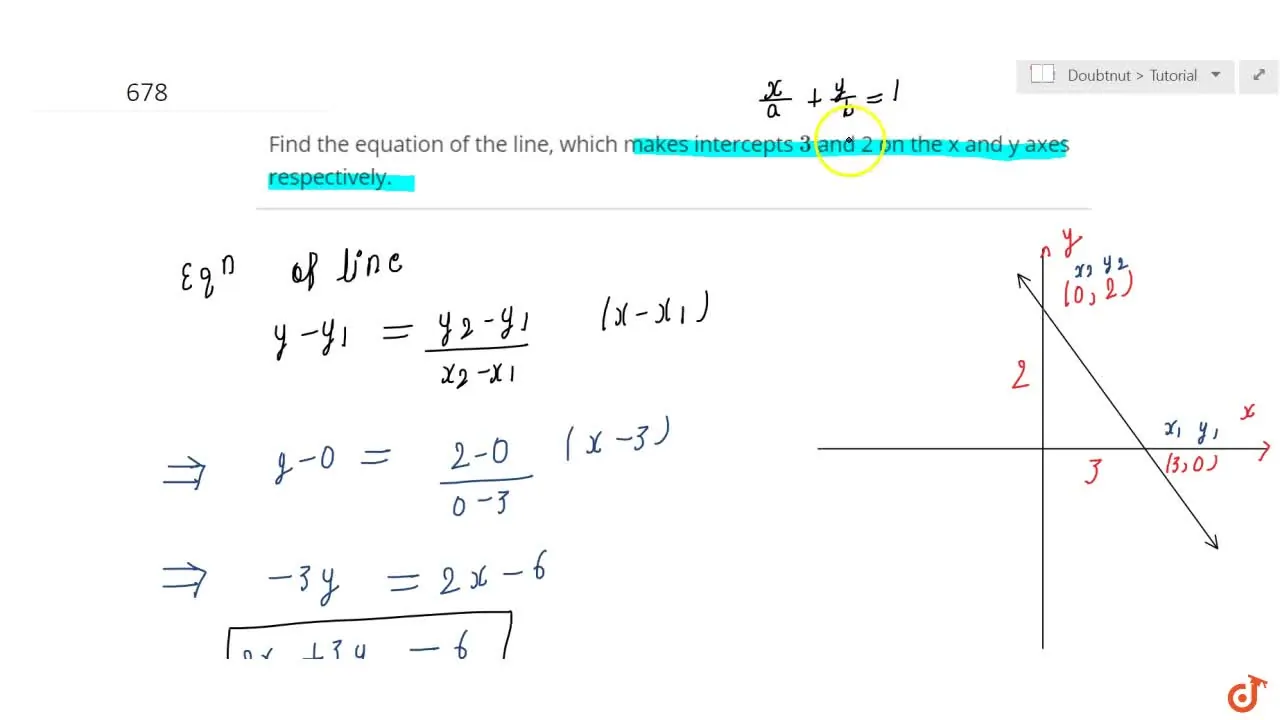
Find the equation of the line, which makes intercepts $3$ and 2 on the x and y axes respectively.

Video Solution

Text Solution

Verified by Experts

$x cos θ + 4 sin θ = p$
let p=normal distance from origin
$θ$ =angle normal makes with +ve x direction
given $P = 4$
$θ = 15^{\circ}$
since we know that
$cos 2 θ = 2 {cos θ}^{2} - 1$
$t a k e θ = 15^{\circ}$
${cos 30}^{\circ} = 2 {cos}^{2} 15 - 1$
$\frac{\sqrt{3}}{2} + 1 = 2 {cos}^{2} 15$
$cos 15 = \sqrt{(\frac{\sqrt{3}}{4} + 2)}$
now ${(\sqrt{3} + 1)}^{2} = 3 + 2 \sqrt{3} + 1$
$= \frac{\sqrt{\frac{{(\sqrt{3} + 1)}^{2}}{2} - 2 + 2}}{4}$
${cos 15}^{\circ} = \frac{\sqrt{{(\sqrt{3} + 1)}^{2}}}{8}$
$= \frac{\sqrt{3} + 1}{2 \sqrt{2}}$
$sin 15 = \sqrt{1} - {cos}^{2} 15$
$= \sqrt{1 - \frac{\sqrt{3} + 2}{4}}$
$= \frac{\sqrt{4 - (\sqrt{3} +)}}{4}$
$sin 15 = \frac{\sqrt{2 - 15}}{4}$
${(\sqrt{3} - 1)}^{2} = 1 + 3 - 2 \sqrt{3}$
$- \sqrt{3} = \frac{{(\sqrt{3} - 1)}^{2} - 4}{2}$
$sin 15 = \frac{\sqrt{2 + ⎛ ⎝ \frac{{(\sqrt{3} - 1)}^{2}}{2} ⎞ ⎠ - 2}}{4}$
$sin 15 = {\sqrt{\sqrt{3} - 1}}^{2} \frac{)}{8}$
$= \frac{\sqrt{3} - 1}{2 \sqrt{2}}$
$x cos θ + 4 sin θ = P$
$x (\frac{\sqrt{3} + 1}{2 \sqrt{2}}) + 4 (\frac{\sqrt{3} - 1}{2 \sqrt{2}}) = 4$
$x ((\sqrt{3} + 1)) + 4 ((\sqrt{3} - 1)) = 8 \sqrt{2}$

Updated on:21/07/2023