Two identical cells each of emf 1.5 V are connected in parallel across a parallel combination of two resistors each of resistance 20Ω . A voltmeter connected in the circuit measures 1.2 V. The internal resistance of each cell is :
25Ω
4Ω
5Ω
10Ω
The correct Answer is:C
To solve the problem, we need to find the internal resistance of each cell given the following information:
- Two identical cells, each with an EMF (Electromotive Force) of 1.5 V, are connected in parallel.
- The cells are connected across a parallel combination of two resistors, each of resistance 20 Ω.
- A voltmeter connected in the circuit measures a voltage of 1.2 V.
1. Understanding the Circuit Configuration:
- We have two cells in parallel, each with an EMF of 1.5 V.
- The two resistors (20 Ω each) are also connected in parallel.
- The reading on the voltmeter is 1.2 V, which indicates the potential difference across the parallel combination of the resistors.
2. Calculate the Equivalent Resistance of the Resistors:
- The equivalent resistance Req of two resistors in parallel is given by:
Req=R1⋅R2R1+R2=20⋅2020+20=40040=10Ω
3. Determine the Total Current in the Circuit:
- The total voltage from the cells is 1.5 V. However, the voltmeter reads 1.2 V, which is the voltage across the equivalent resistance.
- Using Ohm's law, the total current I flowing through the circuit can be calculated as:
I=VReq=1.2V10Ω=0.12A
4. Setting Up the Equation for the Total Voltage:
- The total voltage from the cells must equal the voltage drop across the equivalent resistance and the internal resistance of the cells.
- The total resistance in the circuit is the internal resistance of the two cells in parallel plus the equivalent resistance of the resistors:
Rtotal=R+Req=R+10Ω
- The voltage equation can be set up as:
1.5V=I⋅(R+10)
- Substituting the value of I:
1.5=0.12⋅(R+10)
5. Solving for Internal Resistance R:
- Rearranging the equation:
1.5=0.12R+1.2
- Subtracting 1.2 from both sides:
0.3=0.12R
- Dividing both sides by 0.12:
R=0.30.12=2.5Ω
6. Final Calculation for Internal Resistance:
- Since there are two cells in parallel, the effective internal resistance Rinternal of each cell is:
Rinternal=2.5Ω
Conclusion:
The internal resistance of each cell is 2.5 Ω.
- The cells are connected across a parallel combination of two resistors, each of resistance 20 Ω.
- A voltmeter connected in the circuit measures a voltage of 1.2 V.
- We have two cells in parallel, each with an EMF of 1.5 V.
- The two resistors (20 Ω each) are also connected in parallel.
- The reading on the voltmeter is 1.2 V, which indicates the potential difference across the parallel combination of the resistors.
- The equivalent resistance Req of two resistors in parallel is given by:
Req=R1⋅R2R1+R2=20⋅2020+20=40040=10Ω
- The total voltage from the cells is 1.5 V. However, the voltmeter reads 1.2 V, which is the voltage across the equivalent resistance.
- Using Ohm's law, the total current I flowing through the circuit can be calculated as:
I=VReq=1.2V10Ω=0.12A
- The total voltage from the cells must equal the voltage drop across the equivalent resistance and the internal resistance of the cells.
- The total resistance in the circuit is the internal resistance of the two cells in parallel plus the equivalent resistance of the resistors:
Rtotal=R+Req=R+10Ω
- The voltage equation can be set up as:
1.5V=I⋅(R+10)
- Substituting the value of I:
1.5=0.12⋅(R+10)
- Rearranging the equation:
1.5=0.12R+1.2
- Subtracting 1.2 from both sides:
0.3=0.12R
- Dividing both sides by 0.12:
R=0.30.12=2.5Ω
- Since there are two cells in parallel, the effective internal resistance Rinternal of each cell is:
Rinternal=2.5Ω
The internal resistance of each cell is 2.5 Ω.
Topper's Solved these Questions
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - Physics - Section B
40 Videos
- JEE MAINS 2022BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2022EXERCISE - PHYSICS- SECTION A
19 Videos
- JEE MAINS 2021BOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2021EXERCISE - Physics (Section B )
10 Videos
- JEE MAINS 2023 JAN ACTUAL PAPERBOOK - JEE MAINS PREVIOUS YEARCHAPTER - JEE MAINS 2023 JAN ACTUAL PAPEREXERCISE - Question
360 Videos
Similar Questions
In the given circuit, an ideal voltmeter connected across the 10Ω resistance reads 2V. The internal resistance r, of each cell is:
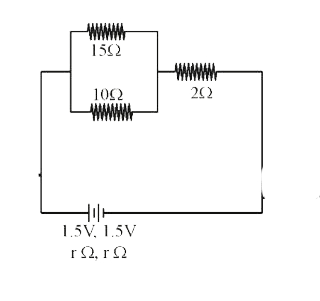
View SolutionThree identical cells each of emf 2 V and unknown internal resistance are connected in parallel. This combination is connected to a 5Ω resistor. If the terminal voltage across the cells is 1.5 V, what is the internal resistance of each cell ?
View Solution
Knowledge Check
Five dry cell each of e.m.f 1.5V are connected in parallel. The e.m.f of the combination is
A7.5 VB0.3 VC3VD1.5 VIf six identical cells each having an e.m.f. of 6 V are connected in parallel, the e.m.f. of the combination is
A1VB36VC16VD6VIn a mixed grouping of identical cells, five rows are connected in parallel and each row contains 10 cells. This combination sends a current I through an external resistance of 20Ω. if the emf and internal resistance of each cell is 1.5 V and 1Ω, respectively then find the value of I.
A5/22 AB15/22 AC3/7 AD5/9 A
A galvanometer together with an unkonwn resistance in series is connected across two identical cells, each of emf 1.5 V. When the cells are connected inseries, the galvanometer records a current of 1 A and when the cells are connected in parallel, the current =0.6 A. What is the internal resistance of each cell ?
View SolutionA cell of emf 2V and internal resistance 4Ω is connected across a parallel combination of two resistors of resistance 10Ω and 20Ω. Find the current through each resistor using Kirchhoff's laws.
View SolutionThree identical cells each of 2 V and unknow internal resistance are connected in parallel. This combination is connected to a 5 ohm resistor. IF the terminal voltage across the cell is 1.5 volt, what is the internal resistance of each cell?
View SolutionTwo identical cells of emf 1.5 V each joined in parallel provided supply to an external circuit cosisting of two resistances of 17Ω each joined in parallel. A very high resistance voltmeter reads. The teminal voltage of cells to be 1.4V. Calculate the internal resistance of each cell.
View SolutionA cell of emf 1.5 V is connected with an external resistance 20Ω. The potential difference falls to 1.0 V. The internal resistance of cell is
View Solution
