A cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
2πR2E
πR2/E
(πR2/πR)/E
Zero
The correct Answer is:D
Flux through left surface
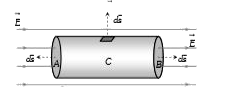
ϕL=−E×πR2
[Cosθ=−1]⇒θ=180∘
ϕR=E×πr2[cosθ=1⇒θ=0∘]
Flux through curved surface C
∮→E.→S=EScosθ=0[θ=90∘]
Total flux ϕL+ϕR+ϕC=0
Similar Questions
A cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionConsider a cylindrical surface of radius R and length l in a uniform electric field E. Compute the electric flux if the axis of the cylinder is parallel to the field direction.
View SolutionA cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionA cylinder of radius r and length l is placed in a uniform electric field of intensity E acting parallel to the axis of the cylinder. The total flux over curved surface area is
View SolutionA cylinder of radius R of length L is placed in a uniform electric field E parallel to the cylinder axis. The total flux for the surface of the cylinder is given by
View SolutionA cylinder of length L and radius R is placed in a uniform electric field E parallel to the axis o the cylinder. The total electric flux for the surface of the cylinder is given by
View SolutionA cylinder of radius r and length l is placed in an uniform electric field parallel to the axis of the cylinder. The total flux for the surface of the cylinder is given by-
View Solution