Consider a cylindrical surface of radius R and length l in a uniform electric field E. Compute the electric flux if the axis of the cylinder is parallel to the field direction.
To compute the electric flux through a cylindrical surface of radius R and length l in a uniform electric field E when the axis of the cylinder is parallel to the field direction, we can follow these steps:
Step 1: Understand the Geometry
The cylindrical surface has two circular ends (top and bottom) and a curved surface. The electric field E is uniform and directed along the axis of the cylinder.
Step 2: Identify the Surfaces
1. Curved Surface: The electric field lines are parallel to the axis of the cylinder, which means that the electric field is perpendicular to the normal of the curved surface.
2. Circular Ends: The electric field is perpendicular to the normal of the circular ends.
Step 3: Calculate the Electric Flux
The electric flux Φ through a surface is given by the formula:
Φ=∫→E⋅d→A
Where d→A is the differential area vector pointing outward from the surface.
Curved Surface:
For the curved surface, the angle between the electric field E and the normal to the surface is 90∘. Therefore, the flux through the curved surface is:
Φcurved=E⋅A⋅cos(90∘)=0
Where A is the area of the curved surface. Since cos(90∘)=0, the flux through the curved surface is zero.
Circular Ends:
For the two circular ends, the electric field is perpendicular to the area vector of the circular surfaces. The area of each circular end is:
A=πR2
The flux through one circular end is:
Φend=E⋅A⋅cos(0∘)=E⋅πR2
Since there are two ends, the total flux through both ends is:
Φtotal=Φend+Φend=E⋅πR2+E⋅πR2=2E⋅πR2
Step 4: Combine the Results
The total electric flux through the entire cylindrical surface is the sum of the flux through the curved surface and the flux through the two circular ends:
Φtotal=0+2E⋅πR2=2E⋅πR2
Final Answer
The electric flux through the cylindrical surface is:
Φ=2E⋅πR2
The cylindrical surface has two circular ends (top and bottom) and a curved surface. The electric field E is uniform and directed along the axis of the cylinder.
1. Curved Surface: The electric field lines are parallel to the axis of the cylinder, which means that the electric field is perpendicular to the normal of the curved surface.
2. Circular Ends: The electric field is perpendicular to the normal of the circular ends.
The electric flux Φ through a surface is given by the formula:
Φ=∫→E⋅d→A
Where d→A is the differential area vector pointing outward from the surface.
For the curved surface, the angle between the electric field E and the normal to the surface is 90∘. Therefore, the flux through the curved surface is:
Φcurved=E⋅A⋅cos(90∘)=0
Where A is the area of the curved surface. Since cos(90∘)=0, the flux through the curved surface is zero.
For the two circular ends, the electric field is perpendicular to the area vector of the circular surfaces. The area of each circular end is:
A=πR2
The flux through one circular end is:
Φend=E⋅A⋅cos(0∘)=E⋅πR2
Since there are two ends, the total flux through both ends is:
Φtotal=Φend+Φend=E⋅πR2+E⋅πR2=2E⋅πR2
The total electric flux through the entire cylindrical surface is the sum of the flux through the curved surface and the flux through the two circular ends:
Φtotal=0+2E⋅πR2=2E⋅πR2
The electric flux through the cylindrical surface is:
Φ=2E⋅πR2
Topper's Solved these Questions
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC FLUX AND GAUSS LAWExercise:Example
Explore 7 Videos
- ELECTRIC FLUX AND GAUSS LAWBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC FLUX AND GAUSS LAWExercise:Exercise 2.1
Explore 16 Videos
- ELECTRIC CURRENT AND CIRCUITBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC CURRENT AND CIRCUITExercise:Interger
Explore 8 Videos
- ELECTRIC POTENTIALBook:CENGAGE PHYSICS ENGLISHChapter:ELECTRIC POTENTIALExercise:DPP 3.5
Explore 15 Videos
Similar Questions
In figure , a cone lies in a uniform electric field E. Determine the electric flux entering the cone.
 .
.
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View SolutionA cylinder of radius R and length L is placed in a uniform electric field E parallel to the axis. The total flux for the surface of the cylinder is given by
View Solutionin the given figure a cone lies in a uniform electric field E the electric flux entering the cone is

View SolutionA cylinder of radius R and length l is placed in a uniform electric field E parallel to the axis of the cylinder. The total flux over the curved surface of the cylinder is
View SolutionIf electric field is uniform, then the electric lines of forces are :
View SolutionA hemispherical surface of radius R is kept in a uniform electric field E as shown in figure. The flux through the curved surface is
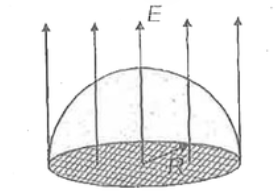
View SolutionA hollow half cylinder surface of radius R and length l is placed in a uniform electric field →E. Electric field is acting perpendicular on the plane ABCD. Find the flux through the curved surface of the hollow cylindrical surface

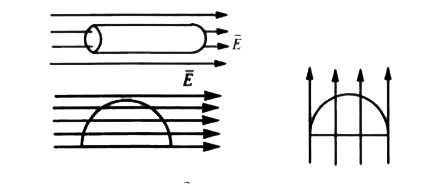
View SolutionIn a uniform electric field find the total flux associated with the given surfaces
View SolutionA Gaussian surface in the cylinder of cross-section πa2 and length L is immersed in a uniform electric field E with the cylinder axis parallel to the field. The flux ϕ of the electric field through the closed surface is
View Solution