A disc of radius R and mass M is pivoted at the rim and it set for small oscillations. If simple pendulum has to have the same period as that of the disc, the length of the simple pendulum should be
54 R
23 R
34 R
32 R
The correct Answer is:D
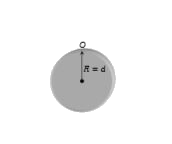
TD=2π√Imgd
=2π
⎷(MR22+MR2)MgR
=2π√3R2g
Since, TP=2π√lgandTP=TD
So, 2π√lg=2π√3R2g
∴l=32R (on comparing).
Similar Questions
In a simple pendulum the period of oscillation (T ) is related to the length of the pendulum (L) as
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View SolutionA disc of radius R is pivoted at its rim. The period for small oscillations about an axis perpendicular to the plane of disc is
View SolutionIn a simple pendulum the period of oscillation (T) is related to the length of the pendulum (L) as
View SolutionA disc of radius R and mass M is pivoted at the rim and it set for small oscillations. If simple pendulum has to have the same period as that of the disc, the length of the simple pendulum should be
View SolutionA disc of radius R and mass M is pivoted at the rim and it set for small oscillations. If simple pendulum has to have the same period as that of the disc, the length of the simple pendulum should be
View SolutionA ring of radius R and mass M is pivoted at the rim and is set for small oscillation about an axis perpendicular to plane of ring. If a simple pendulum has to have the same time period as that of ring, the length of the pendulum should be
View Solution
