Figure below shows a small mass connected to a string, which is attached to a vertical post. If the ball is released when the string is horizontal as shown, the magnitude of the total acceleration (including radial and tangential) of the mass as a function of the angle Θis

gsinθ
g√3cos2θ+1
gcosθ
g√3sin2θ+1
The correct Answer is:D
Radial acceleration = v2R=2glsinθl=2gsinθ
[∴v=√2gh]
Tangential acceleration =gcosθ
Total acceleration
=√4g2sin2θcos2θ+g2cos2θ⇒g√1+3sin2θ
Similar Questions
A pendulum is made by attaching a ball at the end of a string (the other end of the string is attached to the ceiling). The ball is released from rest at the position shown at some angle ϕ. Which of the following vectors may represent the impulse delivered by the string to the ball from the time it is released unitl the ball is at the bottom of the swing when string becomes vertical
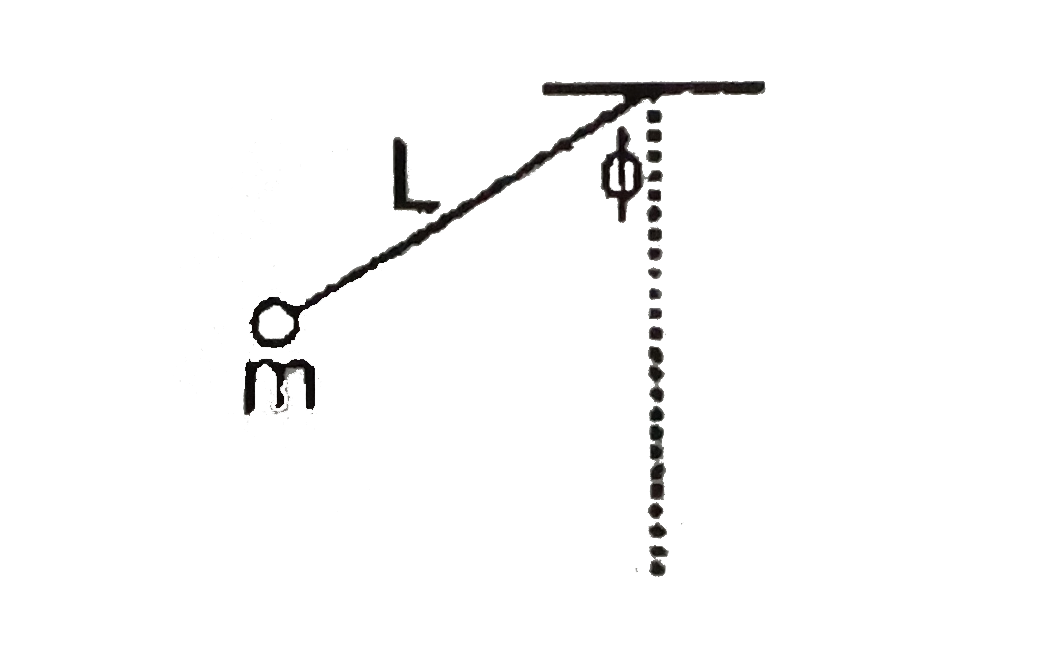
View SolutionIn the given figure balls A&B are released simultaneously , when string is horizontal , length of strings are same but mass of ball B is twice of mass of ball A. Then
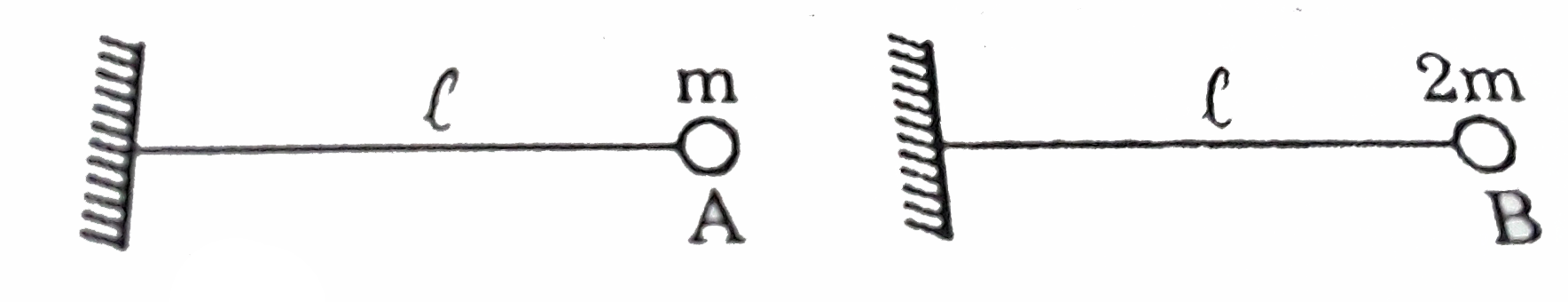
View SolutionA small mass is suspended by a string from the ceiling of a car. As the car accelerates with an acceleration a horizontal road, the angle made by the string with the vertical is
View SolutionA small mass is suspended by a string from the ceiling of a car. As the car accelerates with an acceleration a horizontal road, the angle made by the string with the vertical is
View SolutionA ball connect ed with string is released at an angle 45^(@) with the vertical as shown in figure.Then the acceleration of the box at this instant will be (mass of the box is equal to mass of ball)
View SolutionA small mass is attached to a chord as shown in the picture, the chord is connected to a vertical stationary point as shown in the picture. If the mass is dropped from the break when the chord is horizontal as shown, then the magnitude angle of the resulting acceleration of the mass theta The function will be -
View SolutionA simple pendulum consisting of a mass M attached to a string of length L is released from rest at an angle alpha . A pin is located at a distance l below the pivot point. When the pendulum swings down, the string hits the pin as shown in figure. The maximum angle theta which the string makes with the vertical after hitting the pin is
View SolutionA small ball B of mass m is suspended with light inelastic string of length L from a block A of same mass in which can move on smooth horizontal surface as shown in the figure. The ball is displaced by angle θ from equilibrium position and then released.
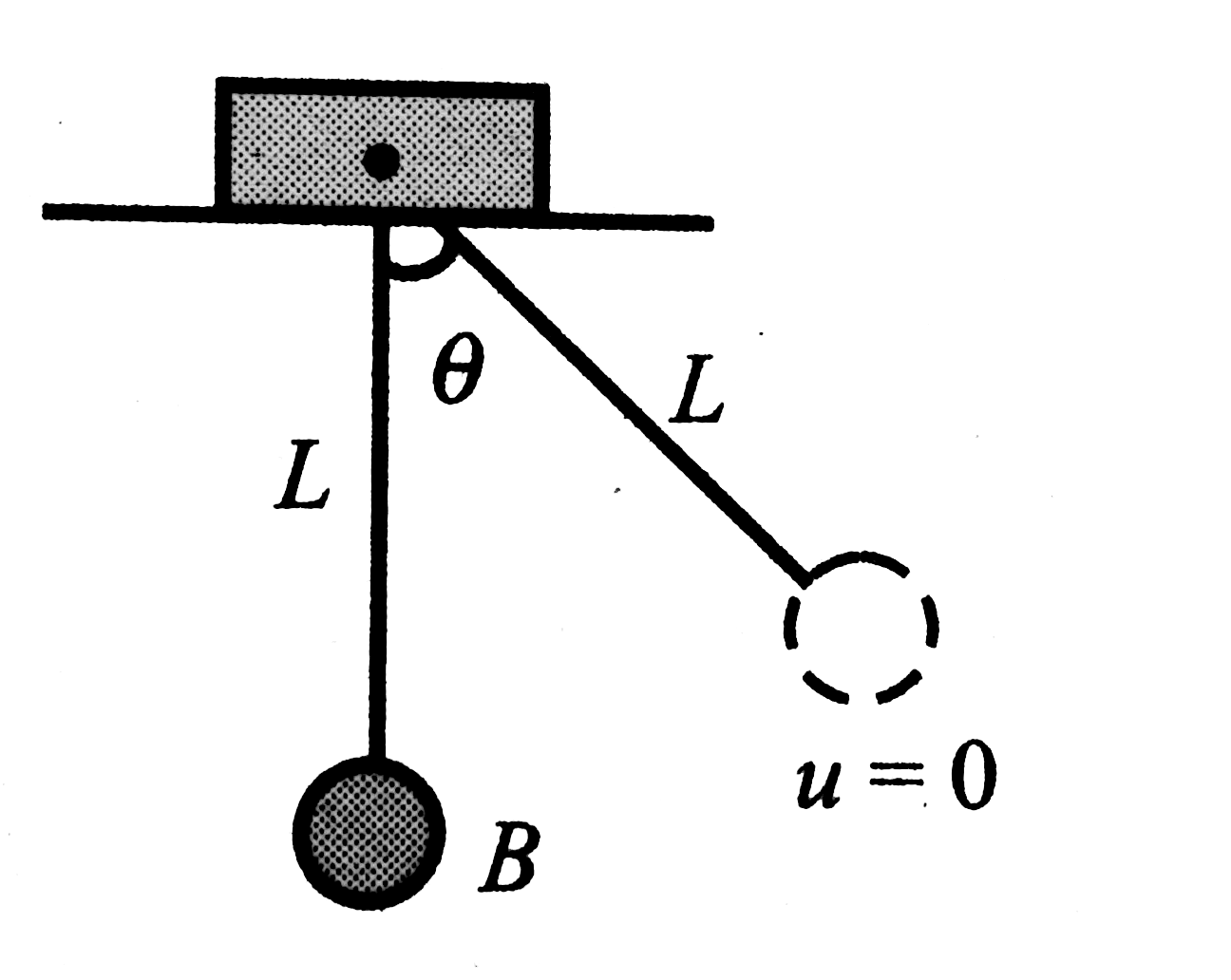
Tension in string when it is vertical, is
View Solution
