The pulleys in filgure are identical, each having a radius R and moment of inertia I. Find the acceleration of the block M.
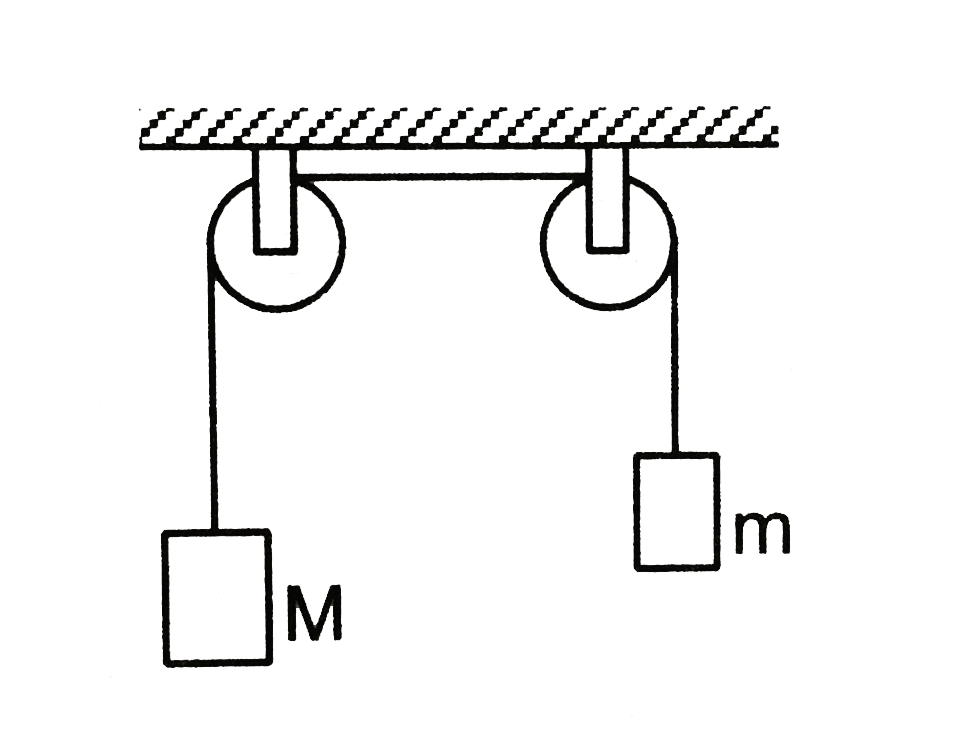 Solution in Gujrati
Solution in Gujrati
The correct Answer is:B
According to the equation
Mg−T1=Ma….1
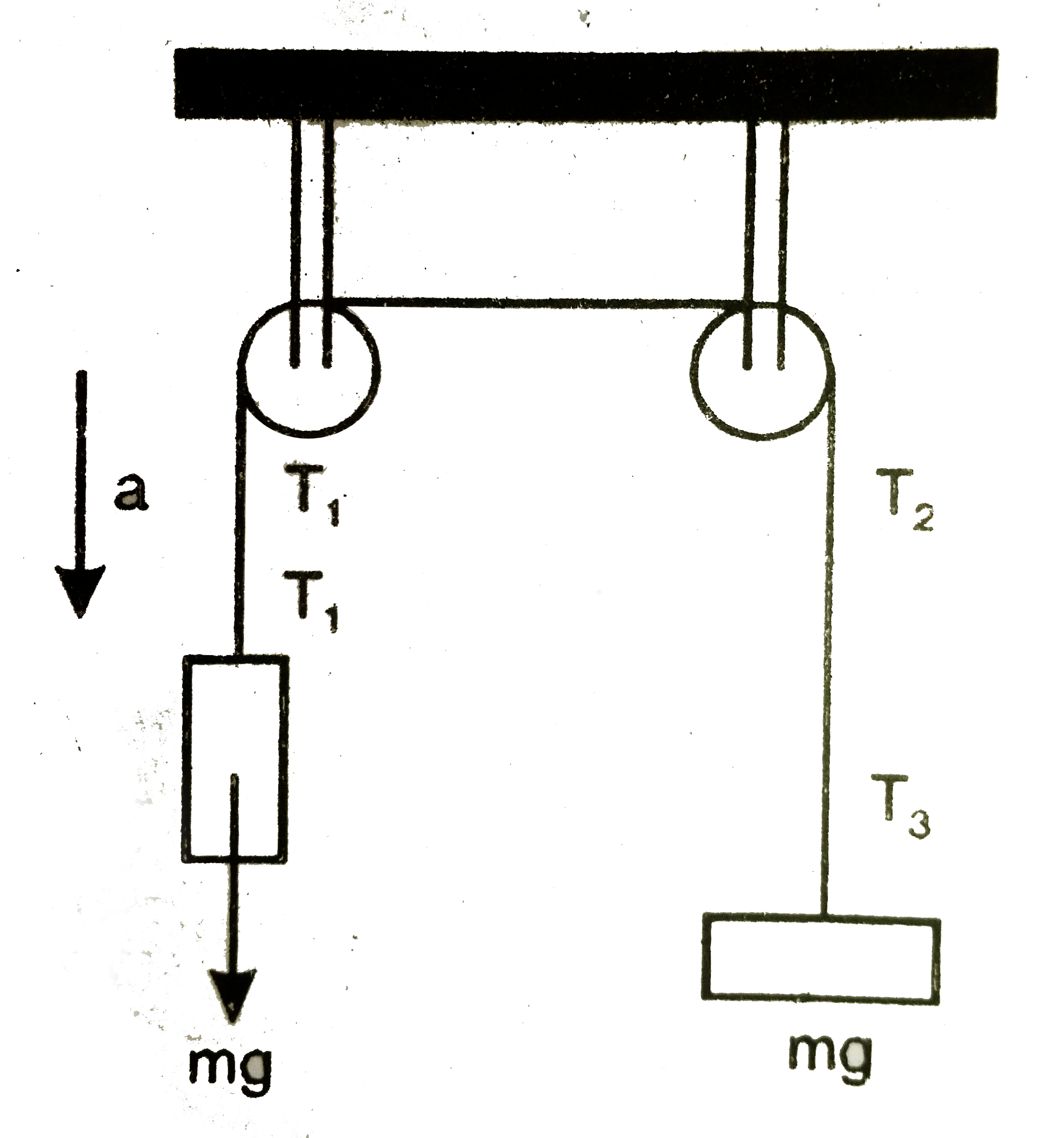
(T1−T2)R=IaR
→(T1−T2)=IaR2….. 2
→(T2−T3)R=IaR2……3
→T3−mg=ma…..4
By adding equation 2 and 3 we will get
→(T1−T3)=2IaR2......5
By adding equation 1 and 4 we will get ltbr> −mg+Mg+(T3−T1)=Ma+ma..6
Substitung the value of T3−T1 we will get
→Mg−mg=Ma+ma+2IaR2
→a=(M−m)G(M+m+2IR2)0
Similar Questions
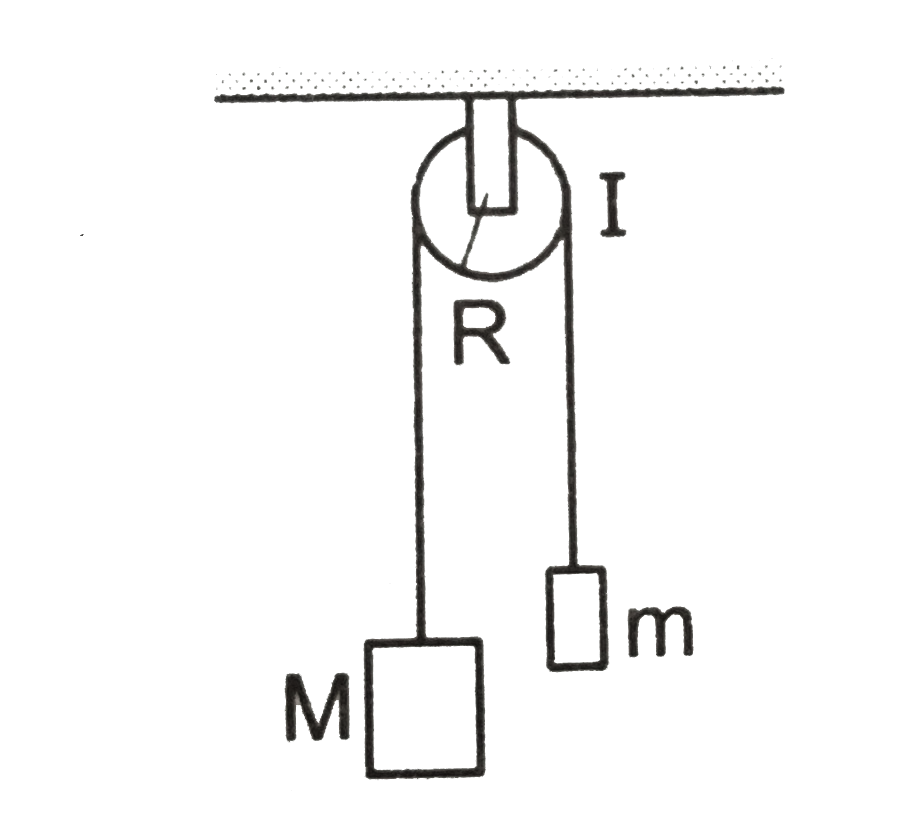
The pulley shown in figure has a moment of inertia I about it's axis and its radius is R. Find the magnitude of the acceleration of the two blocks. Assume that the string is light and does not slip on the pulley.
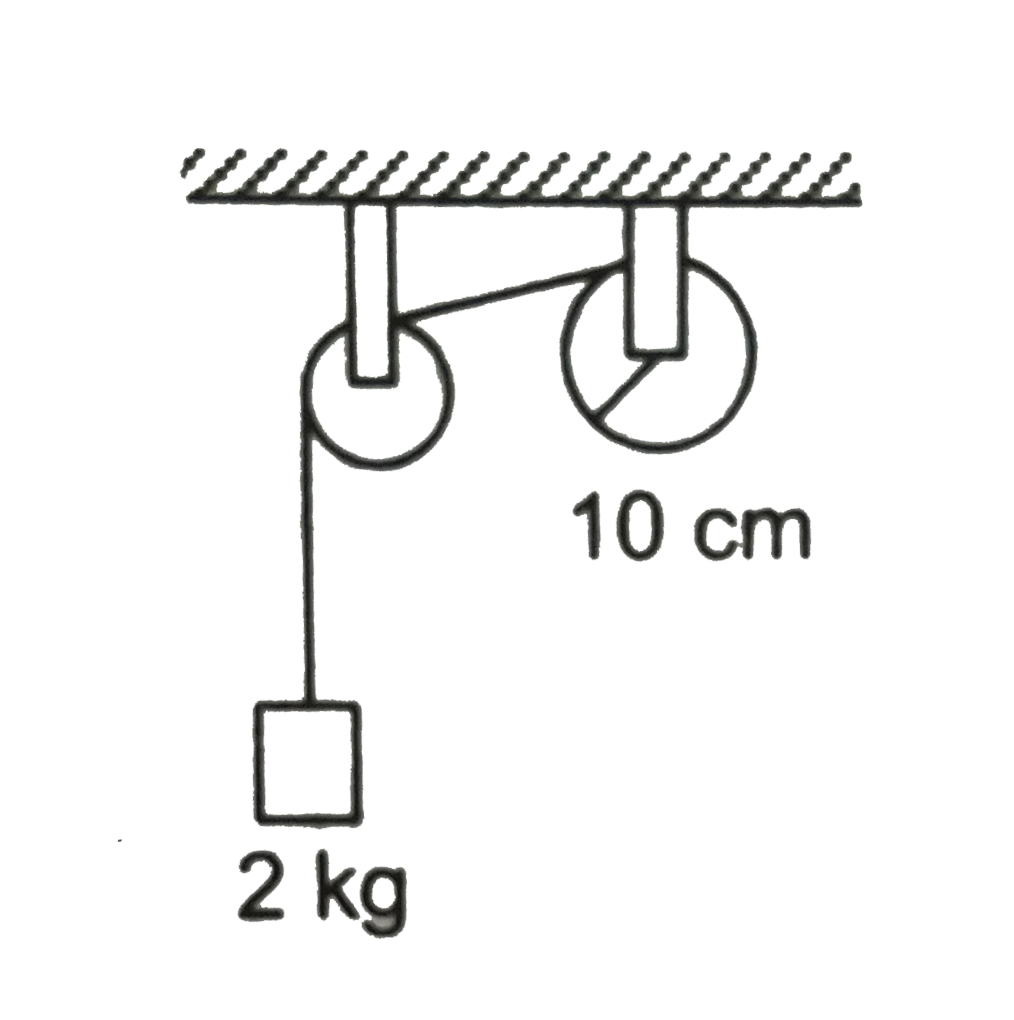
View SolutionA string is wrapped on a wheel of moment of inertia 0.20 kg-m^2 and radius 10 cm and goes through a light pulley to support a block of mass 2.0 kg as shown in figure. Find the acceleration of the block.
View SolutionThe pulleys in figure are identical, each having a radius R and moment of inertia I. Find the acceleration of the block M.

View SolutionThe pulleys in figure are identical, each having a radius R and moment of inertia I. Find the acceleration of the block M.

View SolutionThe pulley of Atwood.s machine has a moment of inertia .I. about its axis and its radius is R. Find the magnitude of acceleration of the two blocks assuming the string is light and does not slip on he pulley.
View SolutionThe pulley of Atwood.s machine has a moment of inertia Tabout its axis and its radius is R. Find the magnitude of acceleration of the two blocks assuming the string is light and does not slip on the pulley.
View SolutionThe pulley of Atwood's machine has a moment of inertia 'I' about its axis and its radius is R. Find the magnitude of acceleration of the two blocks assuming the string is light and does not slip on the pulley.
View SolutionAssuming the moment of inertia of the pulley to be I and its radius to be r.
View Solution

