Question

van der Waal's equation for calculating the pressure of a non ideal gas is
$(P+\frac{a{n}^2}{V^2})(V-nb)=nRT$
van der Waal's suggested that the pressure exerted by an ideal gas , $P_{\text{ideal}}$, is related to the experiventally measured pressure, $P_{\text{ideal}}$ by the equation
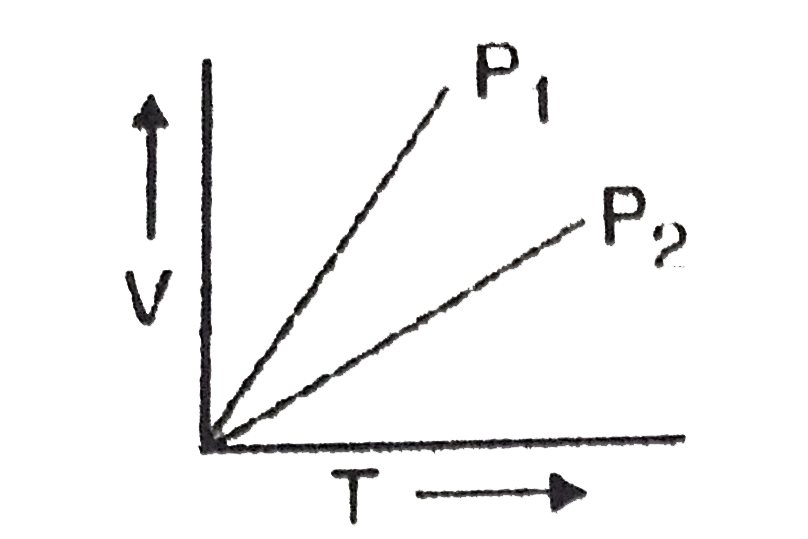
$P_{\text{ideal}}=\underset{\text{observed pressure}}{\underset{\uparrow }{P_{\text{real}}}}+\underset{\text{currection term}}{\underset{\uparrow }{\frac{a{n}^2}{V^2}}}$
Constant 'a' is measure of intermolecular interaction between gaseous molecules that gives rise to nonideal behavior. It depends upon how frequently any two molecules approach each other closely. Another correction concerns the volume occupied by the gas molecules. In the ideal gas equation, V represents the volume of the container. However, each molecule does occupy a finite, although small, intrinsic volume, so the effective volume of the gas vecomes (V-nb), where n is the number of moles of the gas and b is a constant. The term nb represents the volume occupied by gas particles present in n moles of the gas .
Having taken into account the corrections for pressure and volume, we can rewrite the ideal gas equation as follows :
$\underset{\text{corrected pressure}}{(P+\frac{a{n}^2}{V^2})}\underset{\text{corrected volume}}{(V-nb)}=nRT$
AT relatively high pressures, the van der Waals' equation of state reduces to

Options

A. `PV=RT-a//V_(m)`
B. `PV=aRT//V_(m)^(2)`
C. `P=RT-a//V_(m)^(2)`
D. `PV_(m)=RT+Pb`

Answer

d

Explanation

(d) `(P+(a)/(V_(m)^(2)))(V_(m)-b)=RT`
`Puarruarrimpliesdarrdarr" "P+(a)/(V_(m)^(2))~~P`
`P(V_(m)-b)=RT`
`PV_(m)=RT+Pb`