van der Waal's equation for calculating the pressure of a non ideal gas is
(P+an2V2)(V−nb)=nRT
van der Waal's suggested that the pressure exerted by an ideal gas , Pideal, is related to the experiventally measured pressure, Pideal by the equation
Pideal=Preal↑observed pressure+an2V2↑currection term
Constant 'a' is measure of intermolecular interaction between gaseous molecules that gives rise to nonideal behavior. It depends upon how frequently any two molecules approach each other closely. Another correction concerns the volume occupied by the gas molecules. In the ideal gas equation, V represents the volume of the container. However, each molecule does occupy a finite, although small, intrinsic volume, so the effective volume of the gas vecomes (V-nb), where n is the number of moles of the gas and b is a constant. The term nb represents the volume occupied by gas particles present in n moles of the gas .
Having taken into account the corrections for pressure and volume, we can rewrite the ideal gas equation as follows :
(P+an2V2)corrected pressure(V−nb)corrected volume=nRT
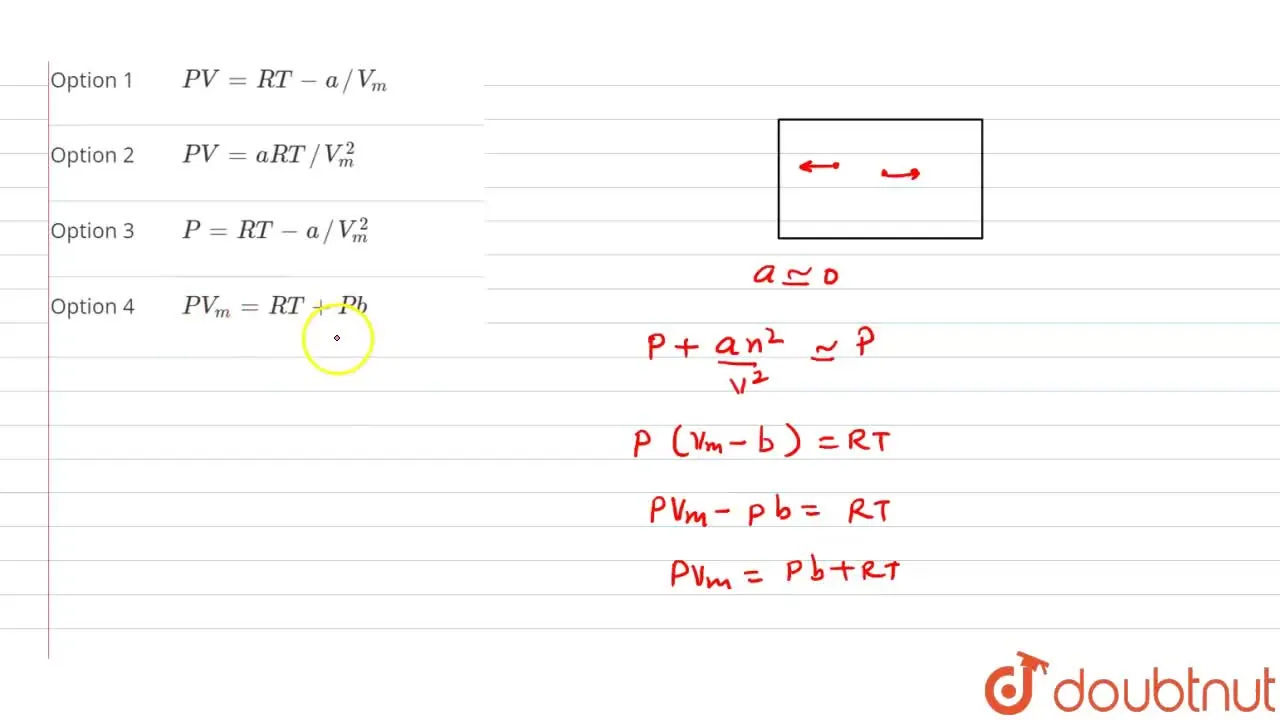
AT relatively high pressures, the van der Waals' equation of state reduces to
A
PV=RT−a/Vm
B
PV=aRT/V2m
C
P=RT−a/V2m
D
PVm=RT+Pb
Video Solution
Text Solution
The correct Answer is:d
(d) (P+aV2m)(Vm−b)=RT
P↑⏐
⏐⏐↑⏐
⏐⏐⇒⏐⏐
⏐↓⏐⏐
⏐↓ P+aV2m≈P
P(Vm−b)=RT
PVm=RT+Pb
|
Updated on:21/07/2023
Related Playlists
- BOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 26videos
GASEOUS STATE
- BOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 3 Passage 34videos
GASEOUS STATE
- BOOK - NARENDRA AWASTHI ENGLISHCHAPTER - GASEOUS STATEEXERCISE - Level 230videos
GASEOUS STATE
- BOOK - NARENDRA AWASTHI ENGLISHCHAPTER - ELECTROCHEMISTRYEXERCISE - Subjective problems14videos
ELECTROCHEMISTRY
- BOOK - NARENDRA AWASTHI ENGLISHCHAPTER - IONIC EEQUILIBRIUMEXERCISE - Subjective problems1videos
IONIC EEQUILIBRIUM
Similar Questions
At low pressure, the van der Waals equation is reduced to
View SolutionAt very high pressure, the van der Waals equation reduces to
View SolutionAt very high pressure, the van der Waals equation reduces to
View SolutionAt high pressure , the van der Waals equation is reduced to
View SolutionAt relatively high pressure, van der Waal's equation for one mole of gas reduces to
View SolutionThe van der Waal equation of gas is
(P+n2aV2)(V−nb)=nRTView SolutionThe van der Waal equation of gas is
(P+n2aV2)(V−nb)=nRTView SolutionVan der Waal's equation at high pressure for 1 mole is
View SolutionAt high temperature and low pressure van der Waal's equation becomes
View SolutionThe pressure P and volume V of an ideal gas both decreases in a process.
View Solution