The current in a conductor varies with time 't' as I = 3t + 4t2. Where I in amp and t in sec. The electric charge flows through the section of the conductor between t = 1s and t = 3s
143C
314C
1403C
3140C
The correct Answer is:C
To find the electric charge that flows through a section of the conductor between t=1 s and t=3 s, we will use the relationship between current and charge. The current I is given by the equation:
I=3t+4t2
We know that current is the rate of flow of charge, which can be expressed mathematically as:
Q=∫t2t1Idt
Where:
- Q is the total charge,
- I is the current,
- t1 and t2 are the time limits (in this case, t1=1 s and t2=3 s).
Step 1: Set up the integral for charge
We will set up the integral to find the charge Q:
Q=∫31(3t+4t2)dt
Step 2: Break down the integral
We can break this integral into two parts:
Q=∫313tdt+∫314t2dt
Step 3: Calculate the first integral
Now we calculate the first integral:
∫3tdt=3t22
Evaluating from t=1 to t=3:
(3(3)22−3(1)22)=(3⋅92−3⋅12)=(272−32)=242=12
Step 4: Calculate the second integral
Now we calculate the second integral:
∫4t2dt=4t33
Evaluating from t=1 to t=3:
(4(3)33−4(1)33)=(4⋅273−4⋅13)=(1083−43)=1043
Step 5: Combine the results
Now we combine the results from both integrals to find the total charge Q:
Q=12+1043
To add these, we convert 12 into a fraction with a denominator of 3:
12=363
So,
Q=363+1043=1403
Final Answer
Thus, the total charge that flows through the section of the conductor between t=1 s and t=3 s is:
Q=1403 coulombs
- Q is the total charge,
- I is the current,
- t1 and t2 are the time limits (in this case, t1=1 s and t2=3 s).
We will set up the integral to find the charge Q:
We can break this integral into two parts:
Now we calculate the first integral:
Now we calculate the second integral:
Now we combine the results from both integrals to find the total charge Q:
Thus, the total charge that flows through the section of the conductor between t=1 s and t=3 s is:
Topper's Solved these Questions
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MOVING CHANGES & MEGNETISM)
Explore 95 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (MAGNETISM AND MATTER )
Explore 52 Videos
- APPENDICES ( REVISION EXERCISE )Book:AAKASH SERIESChapter:APPENDICES ( REVISION EXERCISE )Exercise:REVISION EXERCISE (ELECTRIC POTENTIAL & CAPACITANCE )
Explore 143 Videos
- ALTERNATING CURRENTBook:AAKASH SERIESChapter:ALTERNATING CURRENTExercise:EXERCISE - III
Explore 24 Videos
- APPENDICES (REVISION EXERCISE)Book:AAKASH SERIESChapter:APPENDICES (REVISION EXERCISE)Exercise:LAW OF MOTION
Explore 128 Videos
Similar Questions
The current in a conductor varies with time .t. as I=3t+4t2. Where I in amp and t in sec. The electric charge flows through the section of the conductor between t = 1s and t = 3s
View SolutionThe current in conductor varies with time t as I=2t+3t2 where I is in ampere and t in seconds. Electric charge flowing through a section of the conductor during t=2sec to t=3sec is
View Solution
Knowledge Check
The current in a wire varies with time according to the equation l=4+2t, where l is in ampere and t is in sec. the quantity of charge which has passed through a cross-section of the wire during the time t=2 sec to t=6 sec will be
A40CB48CC38CD43C
The current in conductor varies with time t as I=2t+3t2 where I is in ampere and t in seconds. Electric charge flowing through a section of the conductor during t=2sec to t=3sec is
View SolutionThe current in a 90mH inductor changes with time as i=1.0t2−6t, where I is in amperes and t is in seconds.
View SolutionThe current l through a given cross -section varies with time t as I=3+2t , where l is in ampere and t is in second. The charge passed through this cross-section dring t=0 to t=2 s is
View SolutionThe current through a wire depends on time as, i=(10+4t) Here , i is ampere and t in seconds. Find the charge crossed through section in time interval between t=0 to t=10s.
View SolutionElectric current through a conductor varies with time as I(t)=50sin(100πt). Here I is in amperes and t in seconds. Total charge that passes any point from t=0 to t=1200s is
View SolutionTwo colis, 1 and 2 have a mutual inductance M=5H and resistance R=10Ω each. A current flows in coil 1, which varies with time as: I1=2t2, where t is time. Find the total charge (in C) that has flows through coil 2 between t=0 and t=2s.
View SolutionThe current I through a conductor varies with time t as shown in figure. The average electric current during t=0 to t=10 s will be
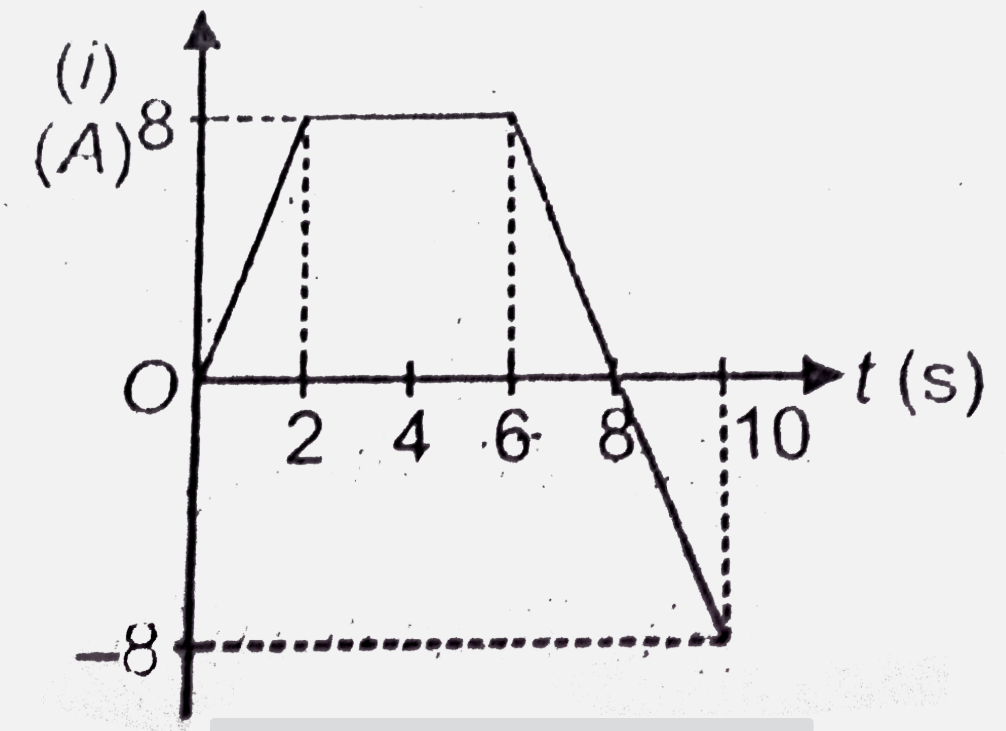
View Solution