The current in a conductor varies with time .t. as I=3t+4t2. Where I in amp and t in sec. The electric charge flows through the section of the conductor between t = 1s and t = 3s
143 C
314 C
1403 C
3140 C
The correct Answer is:C
To solve the problem, we need to find the electric charge that flows through a section of the conductor between t=1s and t=3s given the current I(t)=3t+4t2.
1. Understand the relationship between current and charge:
The current I is defined as the rate of flow of charge Q with respect to time t. Mathematically, this is expressed as:
I=dQdt
From this, we can express the charge Q as:
dQ=Idt
2. Set up the integral for charge:
To find the total charge Q that flows through the conductor from t=1s to t=3s, we integrate the current function over this time interval:
Q=∫t=3t=1I(t)dt
Substituting the expression for I(t):
Q=∫31(3t+4t2)dt
3. Perform the integration:
We can split the integral into two parts:
Q=∫313tdt+∫314t2dt
Now, we calculate each integral separately.
- For the first integral:
∫3tdt=3t22
Evaluating from 1 to 3:
(3(3)22−3(1)22)=(272−32)=242=12
- For the second integral:
∫4t2dt=4t33
Evaluating from 1 to 3:
(4(3)33−4(1)33)=(1083−43)=1043
4. Combine the results:
Now, we add the results of the two integrals:
Q=12+1043
To combine these, we convert 12 into a fraction:
12=363
Thus,
Q=363+1043=1403
5. Final answer:
The total charge that flows through the section of the conductor between t=1s and t=3s is:
Q=1403C
The current I is defined as the rate of flow of charge Q with respect to time t. Mathematically, this is expressed as:
I=dQdt
From this, we can express the charge Q as:
dQ=Idt
To find the total charge Q that flows through the conductor from t=1s to t=3s, we integrate the current function over this time interval:
Q=∫t=3t=1I(t)dt
Substituting the expression for I(t):
Q=∫31(3t+4t2)dt
We can split the integral into two parts:
Q=∫313tdt+∫314t2dt
Now, we calculate each integral separately.
∫3tdt=3t22
Evaluating from 1 to 3:
(3(3)22−3(1)22)=(272−32)=242=12
∫4t2dt=4t33
Evaluating from 1 to 3:
(4(3)33−4(1)33)=(1083−43)=1043
Now, we add the results of the two integrals:
Q=12+1043
To combine these, we convert 12 into a fraction:
12=363
Thus,
Q=363+1043=1403
The total charge that flows through the section of the conductor between t=1s and t=3s is:
Q=1403C
Topper's Solved these Questions
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE-I LEVEL- II (ADVANCED) (Straight Objective Type Questions))
4 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - LECTURE SHEET (EXERCISE-I LEVEL- II (ADVANCED) (More than one correct answer type Questions))
3 Videos
- CURRENT ELECTRICITYBOOK - AAKASH SERIESCHAPTER - CURRENT ELECTRICITYEXERCISE - PROBLEMS (LEVEL-II)
27 Videos
- DUAL NATURE OF RADIATION AND MATTERBOOK - AAKASH SERIESCHAPTER - DUAL NATURE OF RADIATION AND MATTEREXERCISE - PRACTICE EXERCISEX
42 Videos
Similar Questions
The current in conductor varies with time t as I=2t+3t2 where I is in ampere and t in seconds. Electric charge flowing through a section of the conductor during t=2sec to t=3sec is
View SolutionThe current in conductor varies with time t as I=2t+3t2 where I is in ampere and t in seconds. Electric charge flowing through a section of the conductor during t=2sec to t=3sec is
View Solution
Knowledge Check
The current in a wire varies with time according to the equation l=4+2t, where l is in ampere and t is in sec. the quantity of charge which has passed through a cross-section of the wire during the time t=2 sec to t=6 sec will be
A40CB48CC38CD43C
The current in a 90mH inductor changes with time as i=1.0t2−6t, where I is in amperes and t is in seconds.
View SolutionThe current l through a given cross -section varies with time t as I=3+2t , where l is in ampere and t is in second. The charge passed through this cross-section dring t=0 to t=2 s is
View SolutionThe current through a wire depends on time as, i=(10+4t) Here , i is ampere and t in seconds. Find the charge crossed through section in time interval between t=0 to t=10s.
View SolutionTwo colis, 1 and 2 have a mutual inductance M=5H and resistance R=10Ω each. A current flows in coil 1, which varies with time as: I1=2t2, where t is time. Find the total charge (in C) that has flows through coil 2 between t=0 and t=2s.
View SolutionElectric current through a conductor varies with time as I(t)=50sin(100πt). Here I is in amperes and t in seconds. Total charge that passes any point from t=0 to t=1200s is
View SolutionThe current I through a conductor varies with time t as shown in figure. The average electric current during t=0 to t=10 s will be
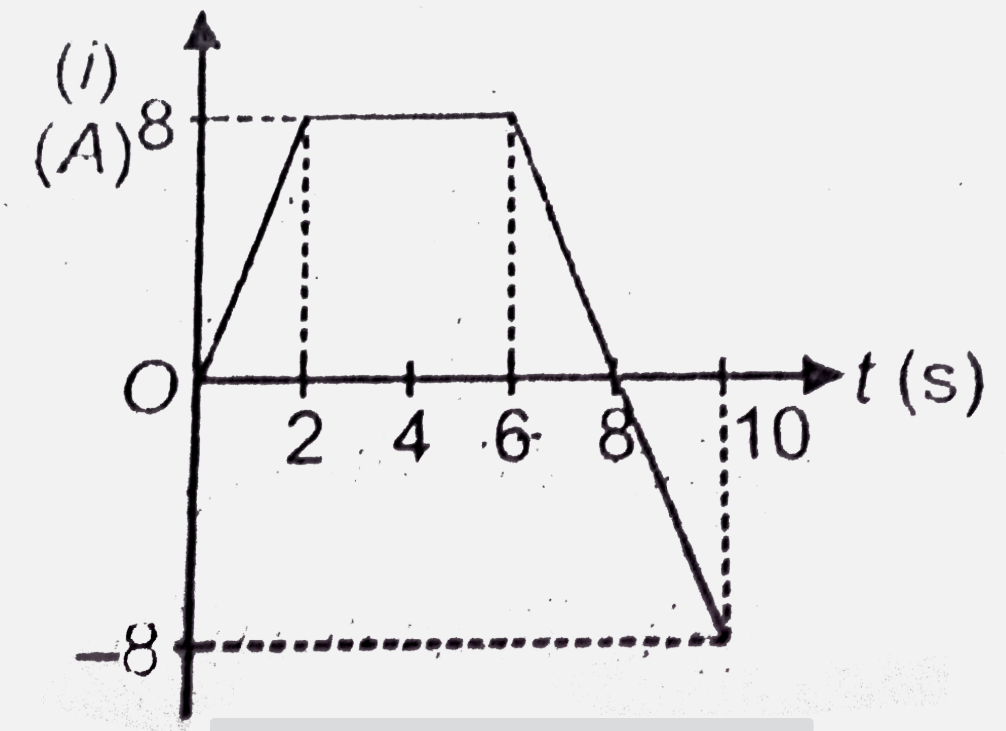
View SolutionThe current flowing through wire depends on time as, I=3t2+2t+5
The charge flowing through the cross - section of the wire in time t=0 to t=2 second is
View Solution
