If two coils are very tightly would one over the other, will M increase or decrease as compared to the case when the coils are placed some distance apart?
M will increase
Related Playlists
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-I)28videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-II)14videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise (Short Answer Questions)21videos
ELECTROMAGNETIC INDUCTION
- BOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)26videos
ELECTRIC FIELD AND POTENTIAL
- BOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II22videos
ELECTROMAGNETIC WAVES
Similar Questions
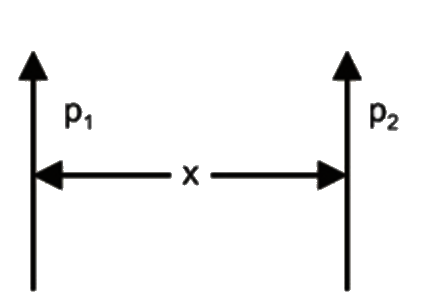
Find the force of interaction of two dipoles , if the two dipole moments are parallel to each other and placed at a distance x apart
 View Solution
View SolutionTwo coil are placed close to each other. The mutual inductance of the pair of coils depends upon.
View SolutionTwo coils are being moved out of magnetic field, one coil is moved rapidly and the other slowly. In which case is more work done and why?
View SolutionTwo identical parallel plate capacitors are connected in one case in parallel and in the other in series. In each case the plates of one capacitors are brought closer by a distance a and the plates of the outer are moved apart by the same distance a. Then
View SolutionAn electric kettle has two coils. When one of these is switched on, the water in the kettle boils in 6 minutes. When the other coil is switched on, the water boils in 3 minutes. If the two coils are connected in series, find the time taken to boil the water in the kettle.
View SolutionTwo circular coils mounted parallel to each other on the same axis carry steady currents. If an observer between the coils reports. that one coil is carrying a clockwise current i1 While the other is carrying a counter - clockwise current i2 then the two coils experience
View SolutionA coil of radius R carries current i1. Another concentric coil of radius r(r<<R) carries current i2. Planes of two coils are mutually perpendicular and both the coil are free to rotate about common diametre. Find maximum kinetic energy of smaller coil when both the coils are released, masses of coils are M and m, respectively.
View SolutionTwo coils are wound on the same iron rod so that the flux generated by one passes through the other . The primary coil has N turns in it and when a current 2 A flows through it the flux in it is 2.5×10−4 Wb . If the secondary coil has 12 turns the mutual inductance of te coils is ( assume the secondary coil is in open circuit )
View SolutionA coil of radius R carries a current i1. Another concentric coil of radius r(r<<R) carries a current i2. Planes of two coils are mutually perpendicular and both the coils are free to rotate about common diameter. Find maximum kinetic energy attained by the two coils, when both are released from rest. The masses of two coils are M and m respectively.
Strategy : The magnetic field due to coil of radius R is μ0i12R. This field exerts a torque on shorter coil. An equal and opposite torque is exerted by the shorter coil on larger coil. There is no external torque acting on the system. The angular momentum remains conserved. Also the total mechanical energy remains conserved. At any instant, let ω1andω2 represent the angular velocityView SolutionTwo coaxial coils are very close to each other and their mutual inductance is 10 mH. If a current (60 A) sin 500t is passed in one of the coils, then find the peak value of induced emf in the secondary coil.
View Solution


