What is an ideal inductor?
Step-by-Step Solution:
1. Definition of an Ideal Inductor: An ideal inductor is an electrical device that is characterized by having no resistance and no capacitance.
2. Properties of an Ideal Inductor:
- No Resistance: This means that the ideal inductor does not dissipate energy as heat. In practical terms, it does not lose energy when current flows through it.
- No Capacitance: This indicates that the ideal inductor does not store energy in an electric field, which is a property associated with capacitors.
3. Inductive Property: The only property that an ideal inductor possesses is inductance. Inductance is defined as the ability of the inductor to oppose changes in the current flowing through it.
4. Understanding Inductance:
- When the current through an inductor changes, it generates a back electromotive force (emf) that opposes the change in current. This is a fundamental principle of electromagnetic induction.
- The unit of inductance is Henry (H), and it quantifies how effectively an inductor can oppose changes in current.
5. Conclusion: Thus, an ideal inductor is a theoretical concept that helps in understanding inductive behavior in circuits without the complications introduced by resistance and capacitance.
- No Resistance: This means that the ideal inductor does not dissipate energy as heat. In practical terms, it does not lose energy when current flows through it.
- No Capacitance: This indicates that the ideal inductor does not store energy in an electric field, which is a property associated with capacitors.
- When the current through an inductor changes, it generates a back electromotive force (emf) that opposes the change in current. This is a fundamental principle of electromagnetic induction.
- The unit of inductance is Henry (H), and it quantifies how effectively an inductor can oppose changes in current.
Topper's Solved these Questions
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-I)
28 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Problems (Level-II)
14 Videos
- ELECTROMAGNETIC INDUCTIONBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC INDUCTIONEXERCISE - Exercise (Short Answer Questions)
21 Videos
- ELECTRIC FIELD AND POTENTIALBOOK - AAKASH SERIESCHAPTER - ELECTRIC FIELD AND POTENTIALEXERCISE - PROBLEMS (LEVEL-II)
26 Videos
- ELECTROMAGNETIC WAVESBOOK - AAKASH SERIESCHAPTER - ELECTROMAGNETIC WAVESEXERCISE - EXERCISE -II
22 Videos
Similar Questions
Knowledge Check
A condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor when the potential difference across the condenser reduces to V2 is
A(C(V1−V2)2L)12BC(V21−V22)LCC(V21+V22)LD(C(V21−V22)L)12
What is an ideal diode ?
View SolutionObtain the expression for the magnetic energy stored in an ideal inductor of self inductance L when a current I passes through it.
Hence obtain the expression for the energy density of magentic field produced in the inductor.
View SolutionA condenser of capacity C is charged to a potential difference of V1. The plates of the condenser are then connected to an ideal inductor of inductance L. The current through the inductor wehnn the potential difference across the condenser reduces to V2 is
View SolutionWhat is an ideal transformer ?
View SolutionAn ideal capacitor of capacitance 0.2μF is charged to a potential difference of 10V. The charging battery is than disconnected. The capacitor is then connected to an ideal inductor of self inductance 0.5mH. The current at a time when the potential difference across the capacitor is 5V is :
View SolutionA time varying voltage V= 2t (Volt) is applied across and ideal inductor of inductance L =2H as shown in Fig. Then (assume current to be zero at t =0)
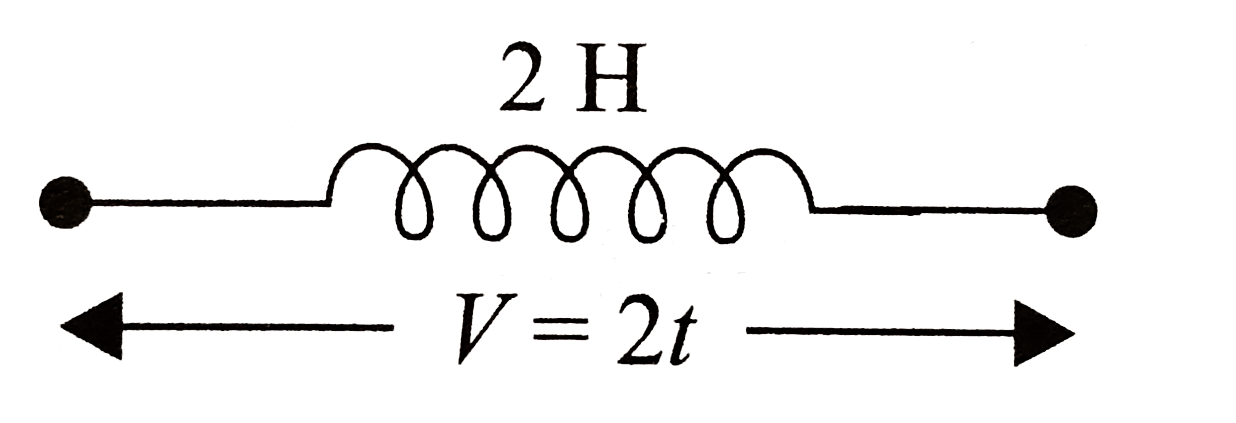
View SolutionWhat is an ideal liquid ?
View Solution
